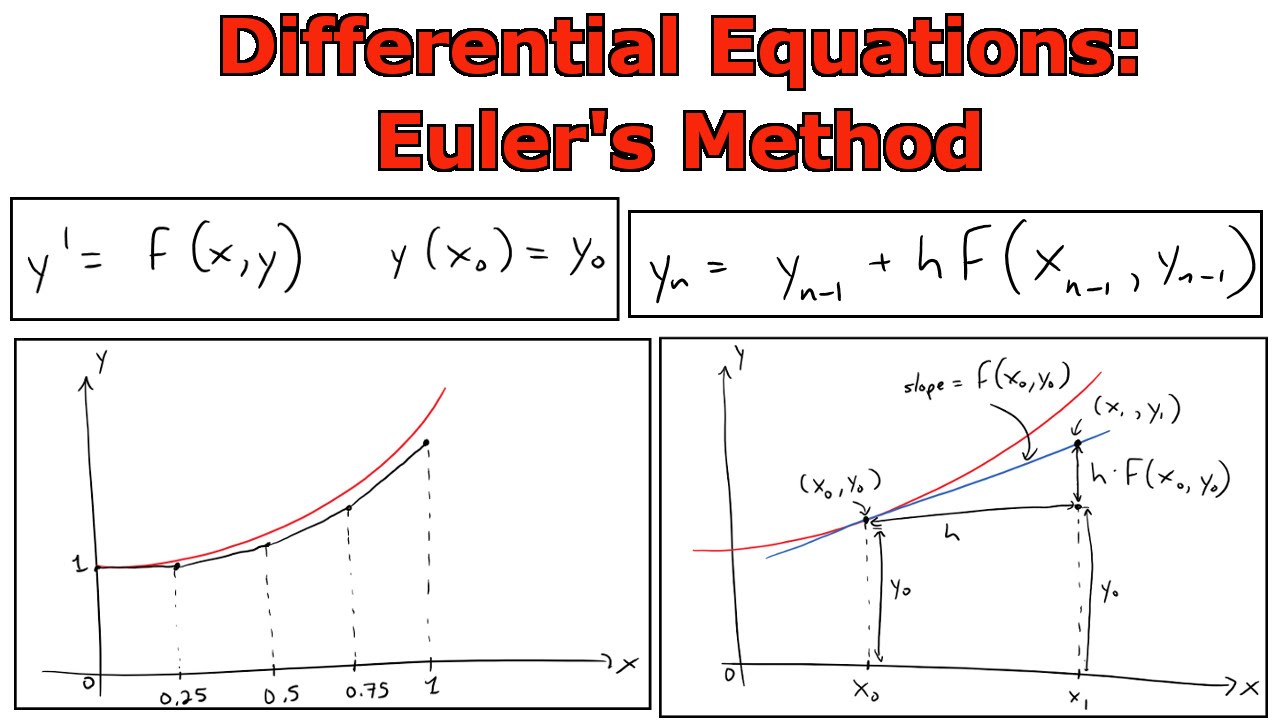
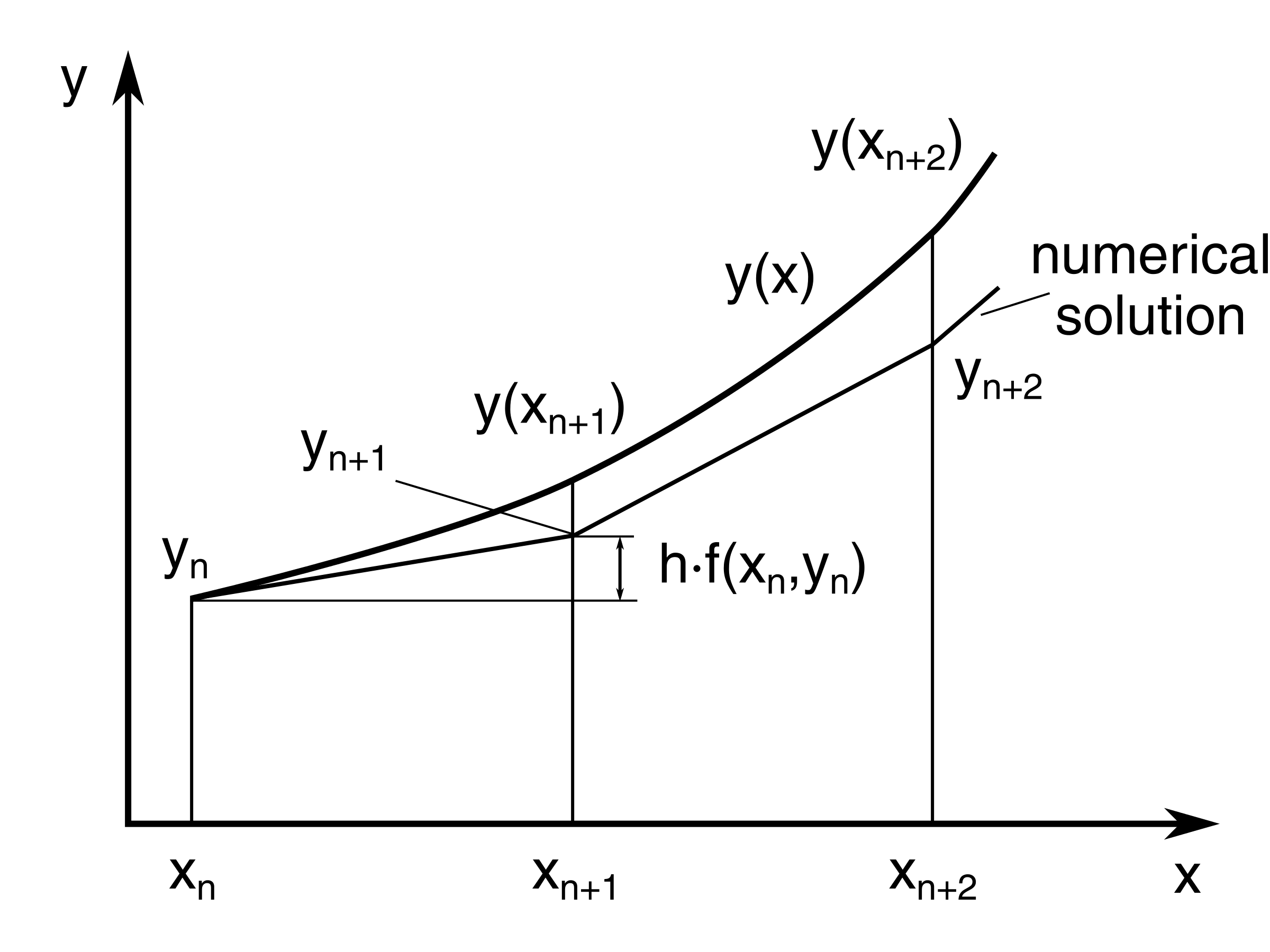
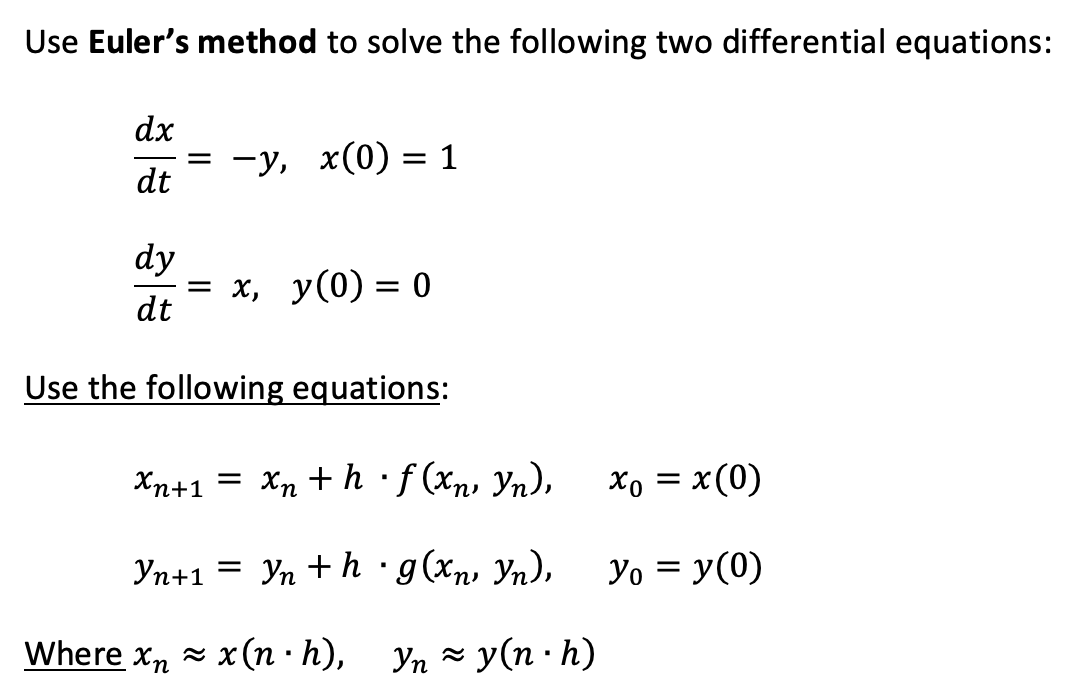
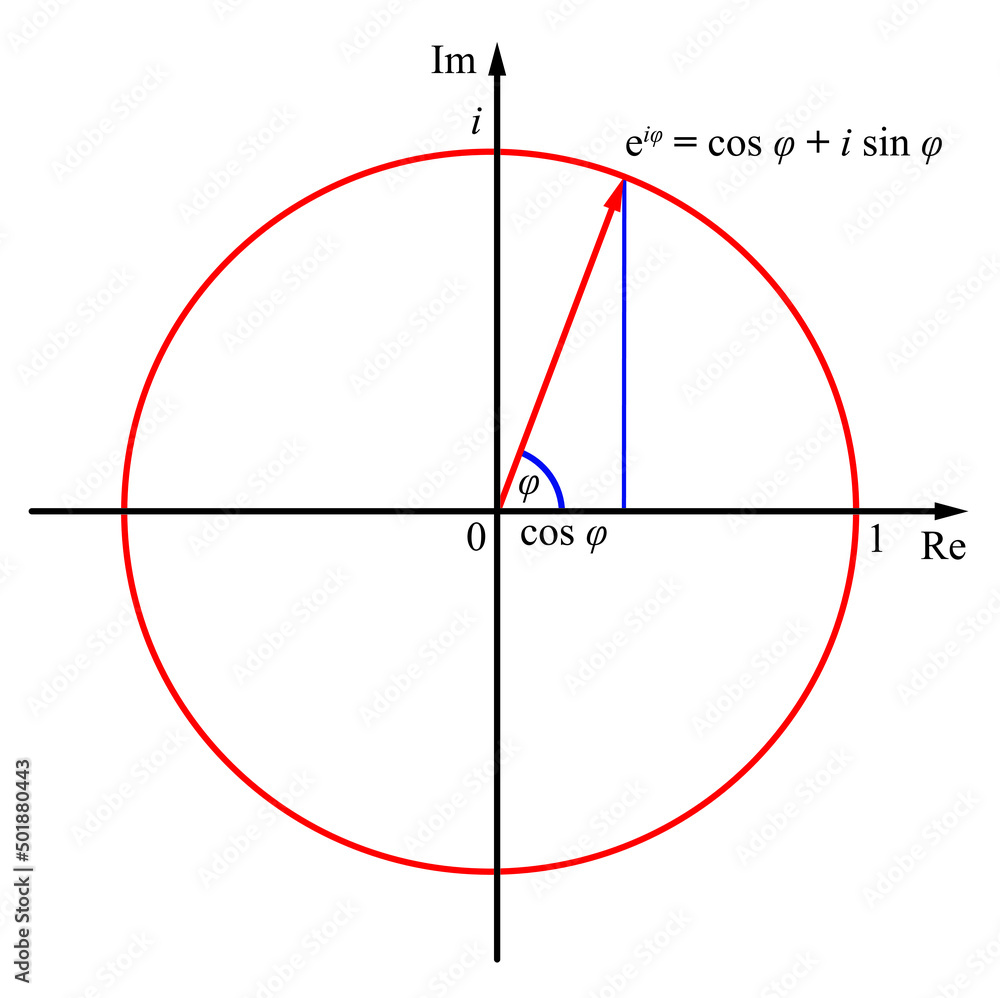
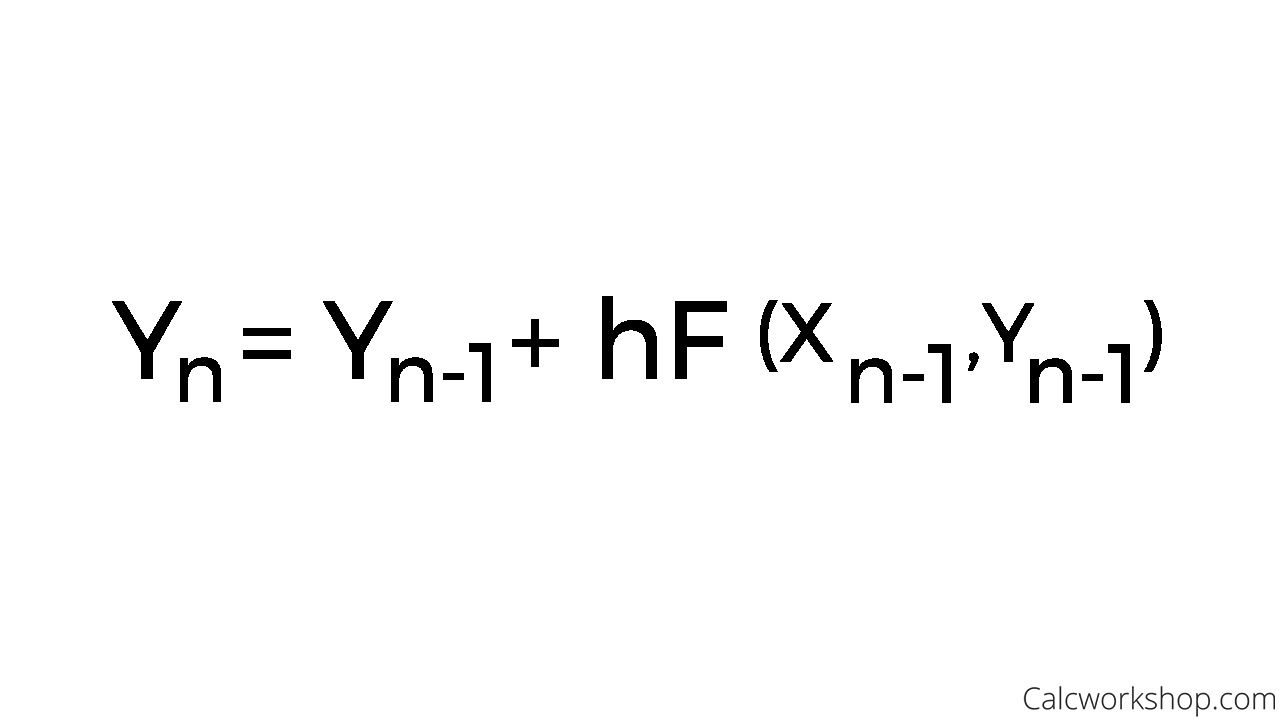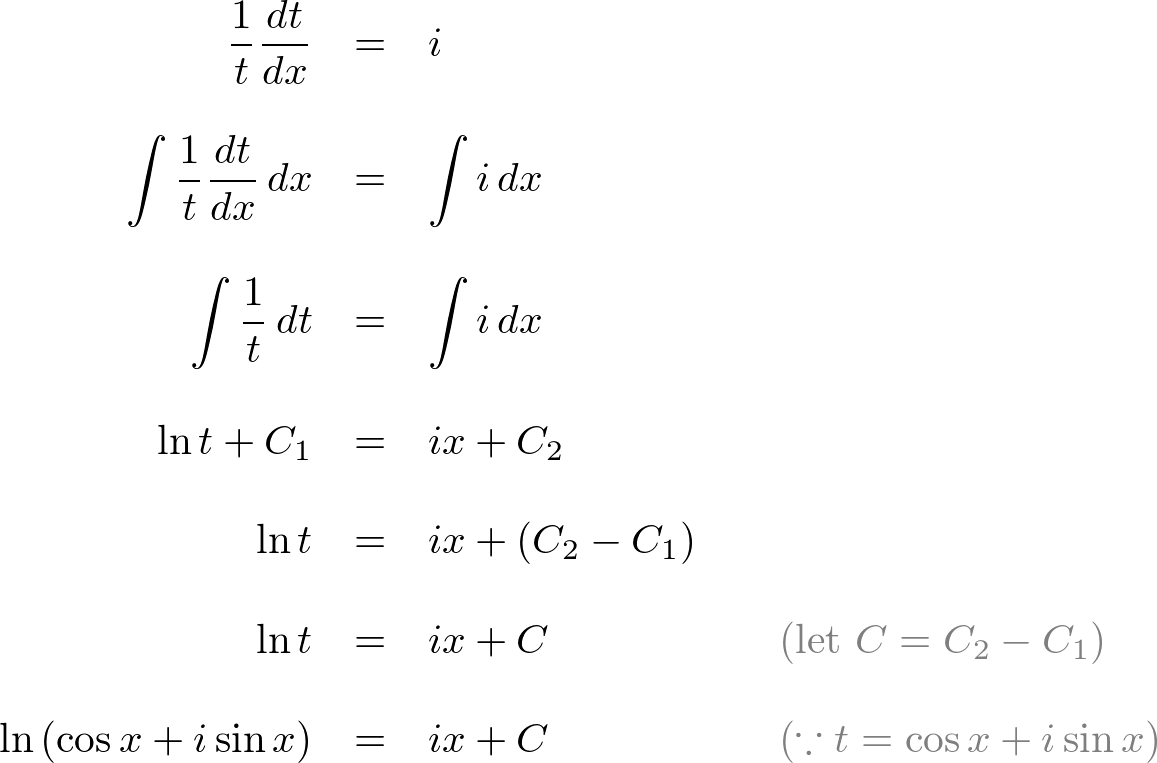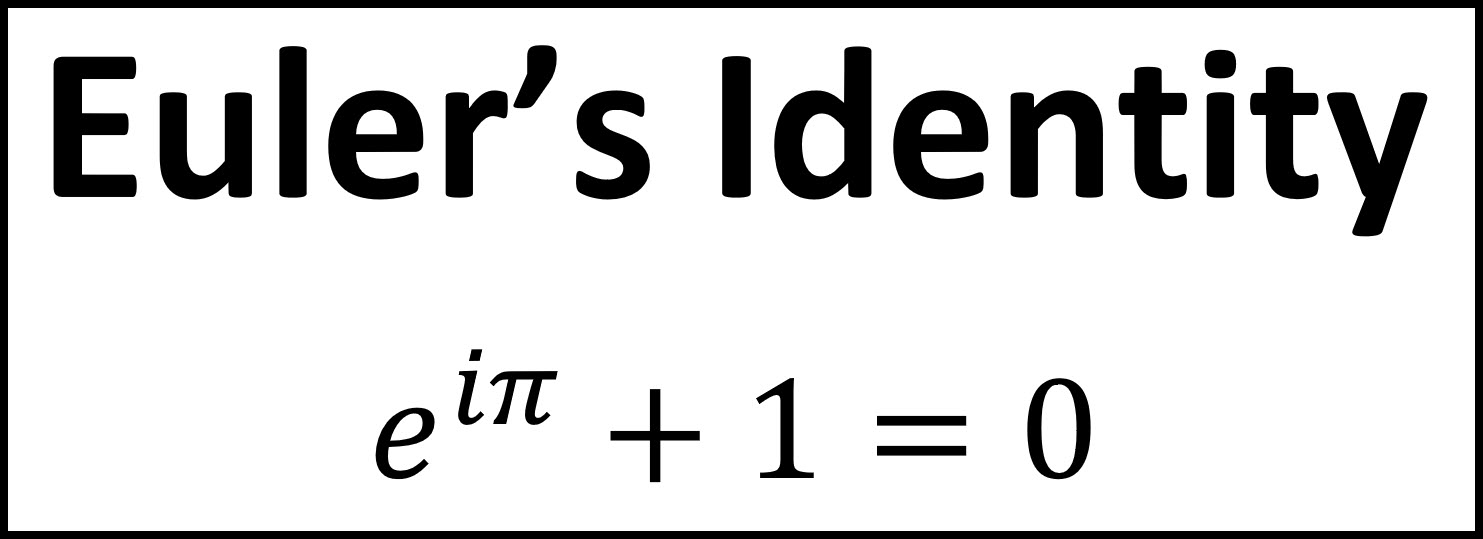Euler's Formula For Differential Equations - Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Euler’s formula, polar representation 1. Note that while this does not involve a. The complex plane complex numbers are represented geometrically by points in the plane: In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0.
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. The complex plane complex numbers are represented geometrically by points in the plane: Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Euler’s formula, polar representation 1.
Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Euler’s formula, polar representation 1. In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. The complex plane complex numbers are represented geometrically by points in the plane:
How to do Euler's Method? (Simply Explained in 4 Powerful Examples)
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. Euler’s formula, polar representation 1. The complex plane complex numbers are represented geometrically by points in the plane: Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of.
SOLUTION Cauchy euler s differential equations Studypool
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). The complex plane complex numbers are represented geometrically by points in the plane: Euler’s formula, polar representation 1. Note that while.
Proof Of Euler's Formula Using Taylor Series darelou
Euler’s formula, polar representation 1. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). The complex plane complex numbers are represented geometrically by points in the plane: Note that while this does not involve a. In this section we will discuss how to solve euler’s differential equation, ax^2y''.
Euler's Method Explained With Examples, 40 OFF
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. Euler’s formula, polar representation 1. Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). The complex plane complex numbers are represented geometrically.
Euler's Method Explained with Examples
Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. The complex plane complex numbers are represented geometrically by points in the plane: Euler’s formula, polar representation 1. Note that while.
Introduction to Euler's Formula Slideshow PPT
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. Euler’s formula, polar representation 1. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Note that while this does not involve a. The complex plane complex numbers are represented geometrically.
Answered Use Euler's method to solve the… bartleby
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. The complex plane complex numbers are represented geometrically by points in the plane: Note that while this does not involve a. Euler’s formula, polar representation 1. Euler’s method is based on the assumption that the tangent line to the integral curve of.
Mathematical Designing of Euler's Formula. Vector Illustration. Stock
Note that while this does not involve a. The complex plane complex numbers are represented geometrically by points in the plane: Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Euler’s formula, polar representation 1. In this section we will discuss how to solve euler’s differential equation, ax^2y''.
Eulers Formula Mathematics Vector Stock Vector (Royalty Free
Euler’s formula, polar representation 1. In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). The complex plane complex numbers are represented geometrically.
Euler's Formula
In this section we will discuss how to solve euler’s differential equation, ax^2y'' + bxy' +cy = 0. The complex plane complex numbers are represented geometrically by points in the plane: Euler’s formula, polar representation 1. Note that while this does not involve a. Euler’s method is based on the assumption that the tangent line to the integral curve of.
In This Section We Will Discuss How To Solve Euler’s Differential Equation, Ax^2Y'' + Bxy' +Cy = 0.
Euler’s formula, polar representation 1. The complex plane complex numbers are represented geometrically by points in the plane: Euler’s method is based on the assumption that the tangent line to the integral curve of equation 3.1.1 at (xi, y(xi)). Note that while this does not involve a.