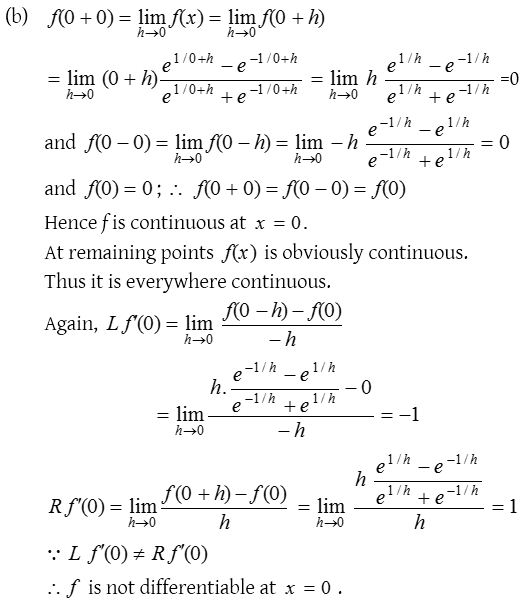
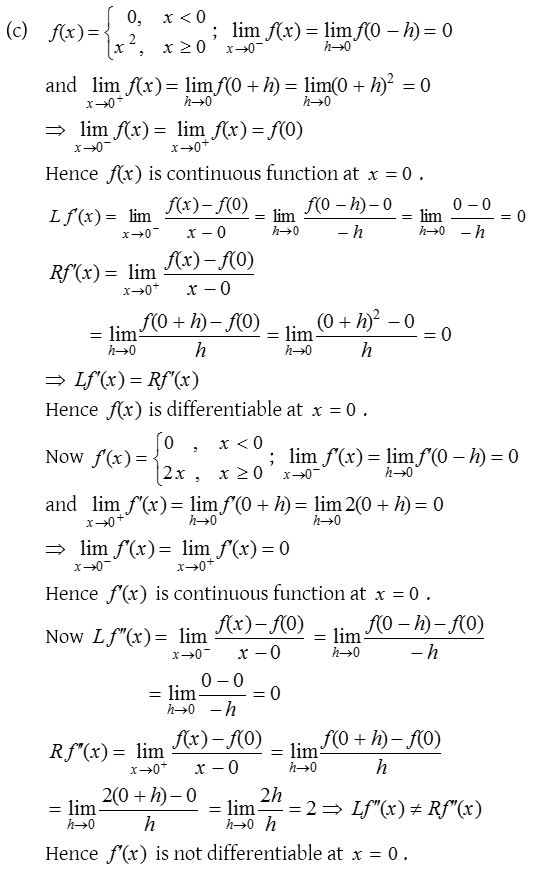
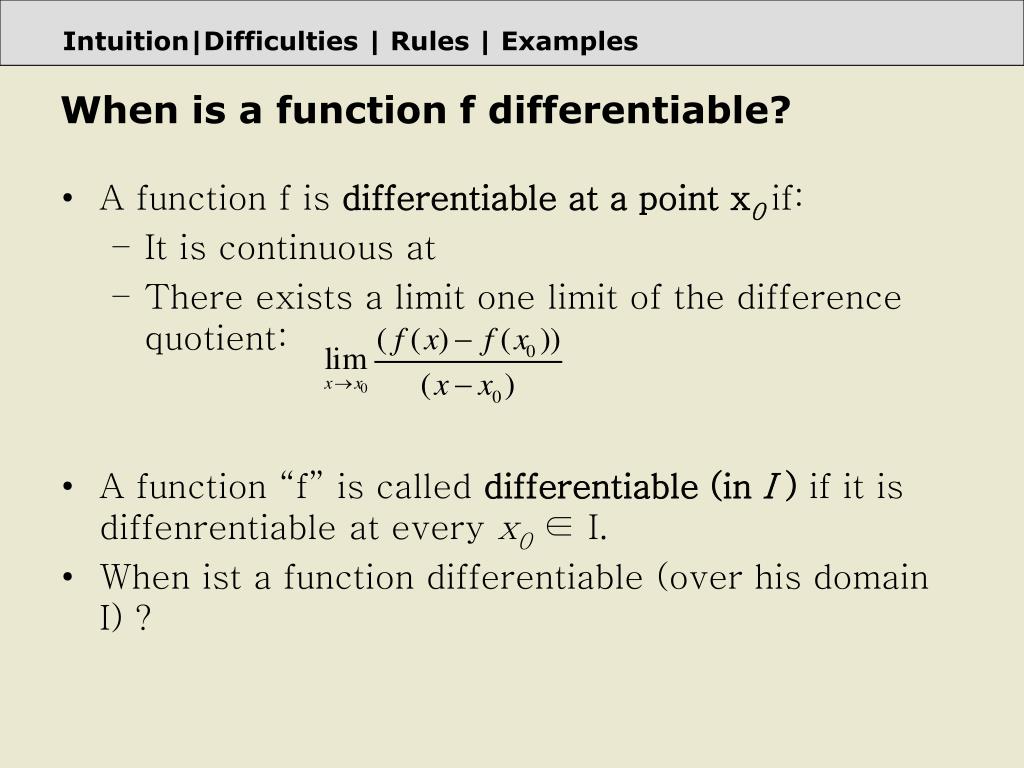
Differentiable Functions - In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
(PDF) Generalizeddifferentiable functions
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
Differentiable Function CBSE Library
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
calculus On Differentiable Functions (Khan Academy) Mathematics
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
Differentiable Design Galleries A Differentiable Approach to Explore
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
Differentiable Function A Plus Topper
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
Solved 3 Surprisingly, for most differentiable functions g
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
Differentiable function Wikiwand
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
PPT Differentiable Functions PowerPoint Presentation, free download
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
PPT Differentiable functions are Continuous PowerPoint Presentation
A diferentiable function is a function that can be approximated locally by a linear function. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
Differentiable vs. Continuous Functions Understanding the Distinctions
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. A diferentiable function is a function that can be approximated locally by a linear function.
A Diferentiable Function Is A Function That Can Be Approximated Locally By A Linear Function.
In calculus, a differentiable function is a continuous function whose derivative exists at all points on.