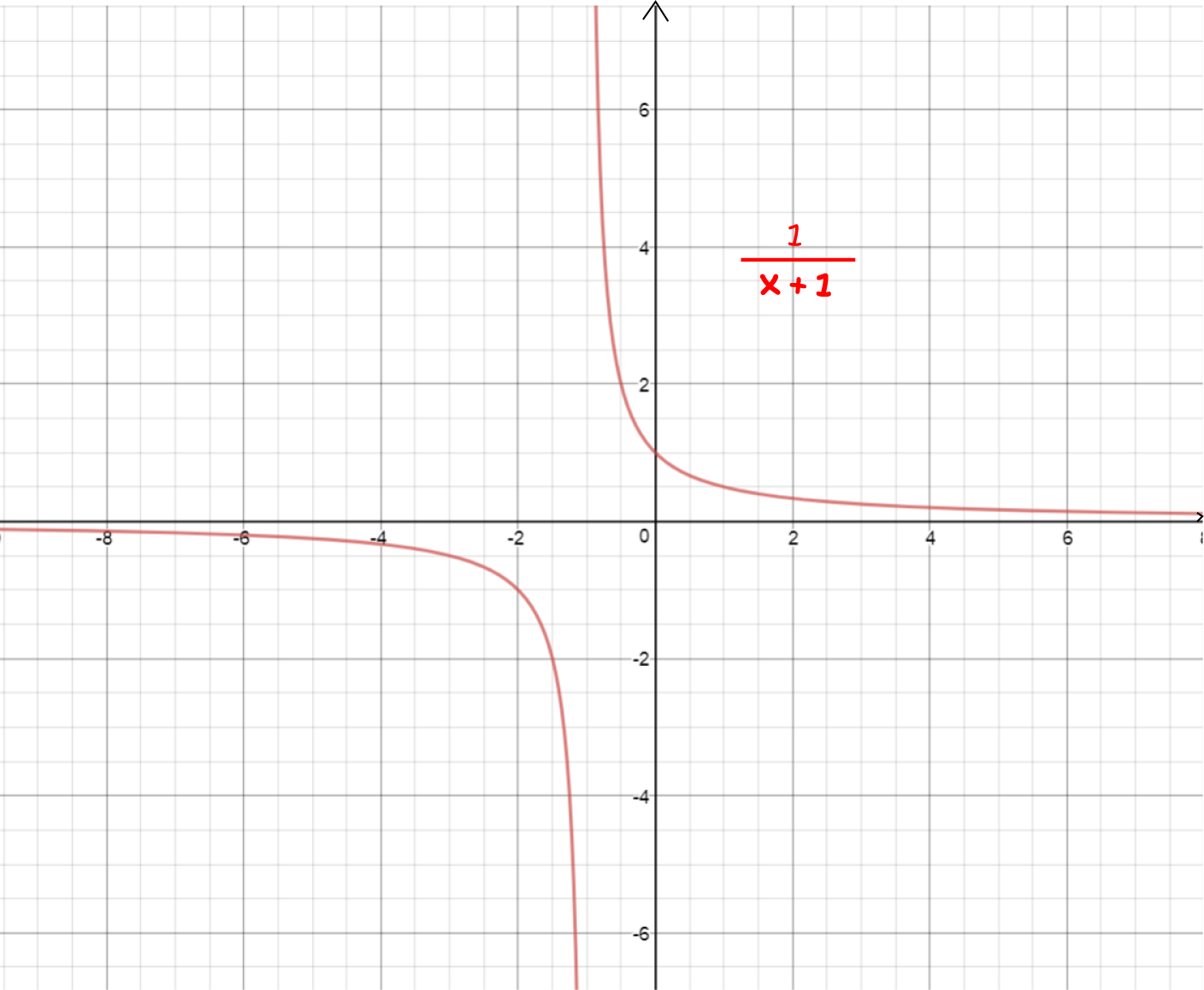
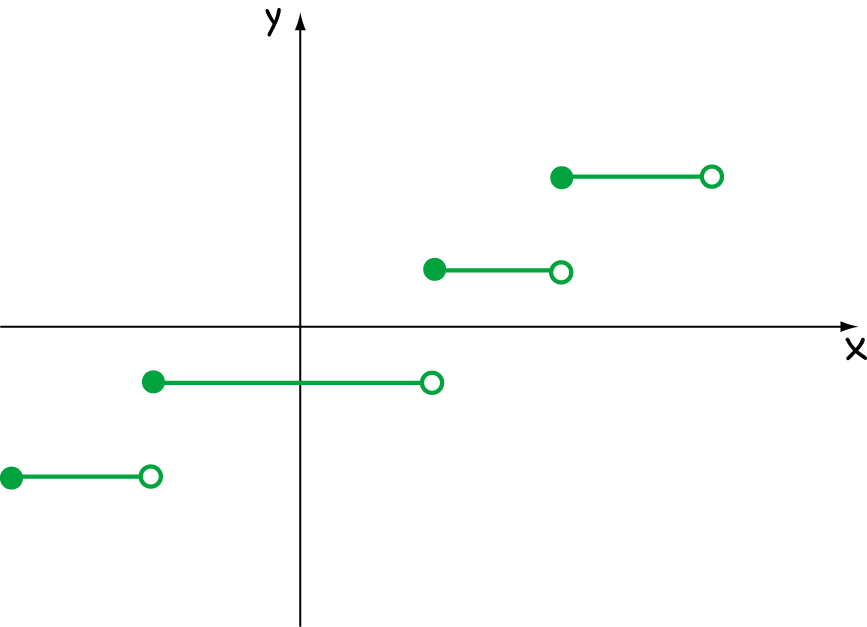
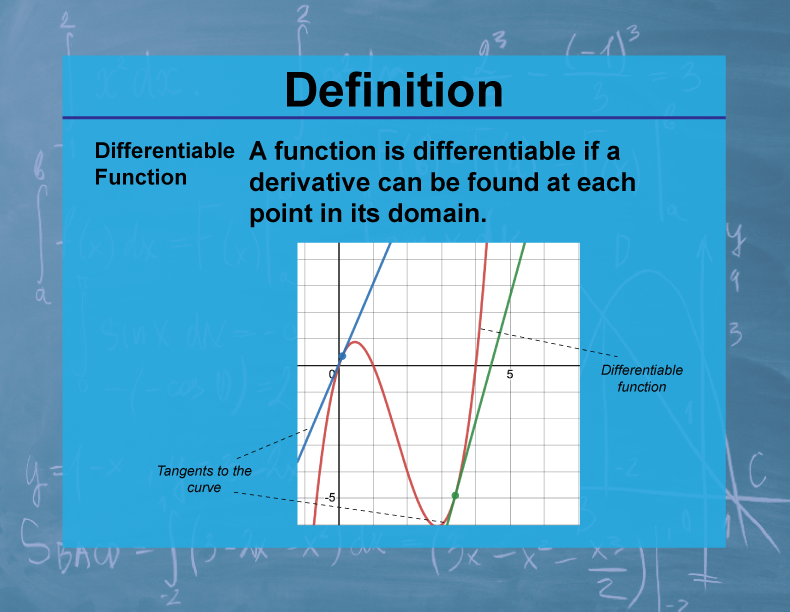
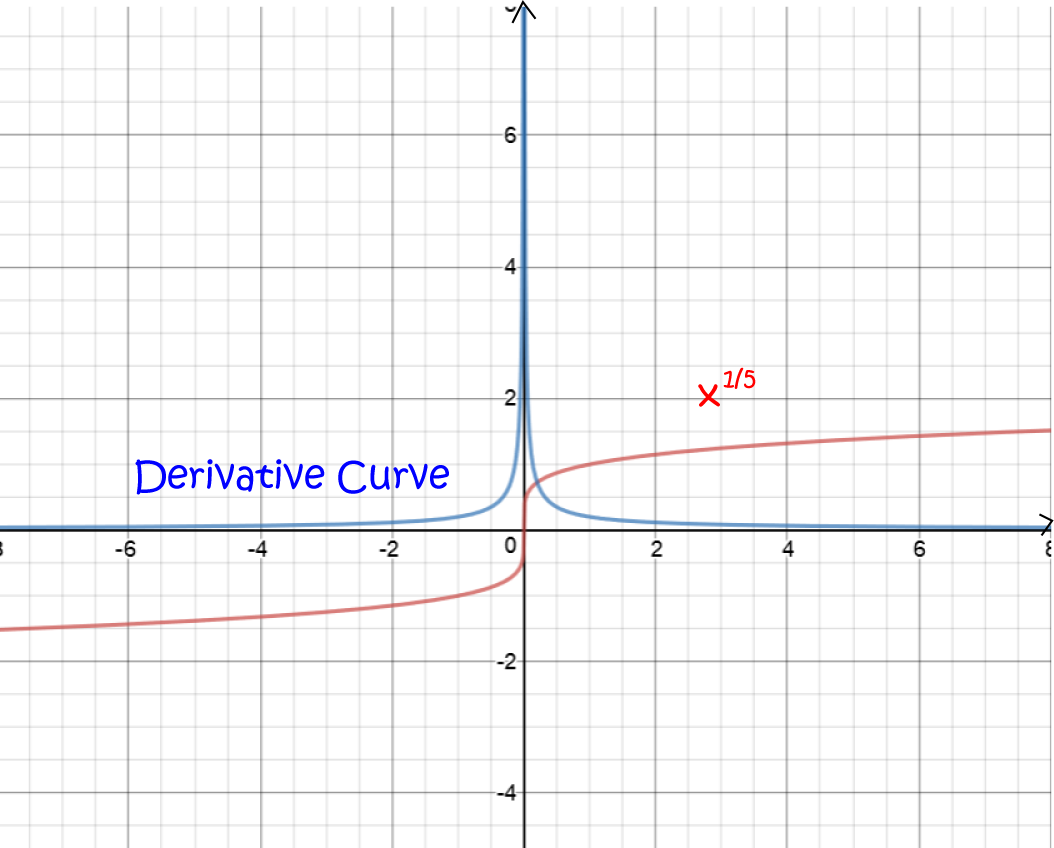
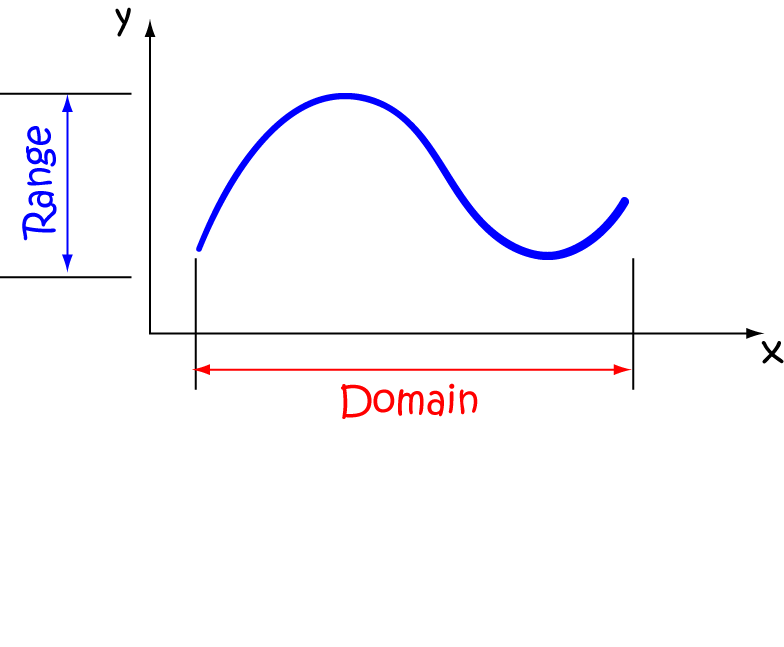
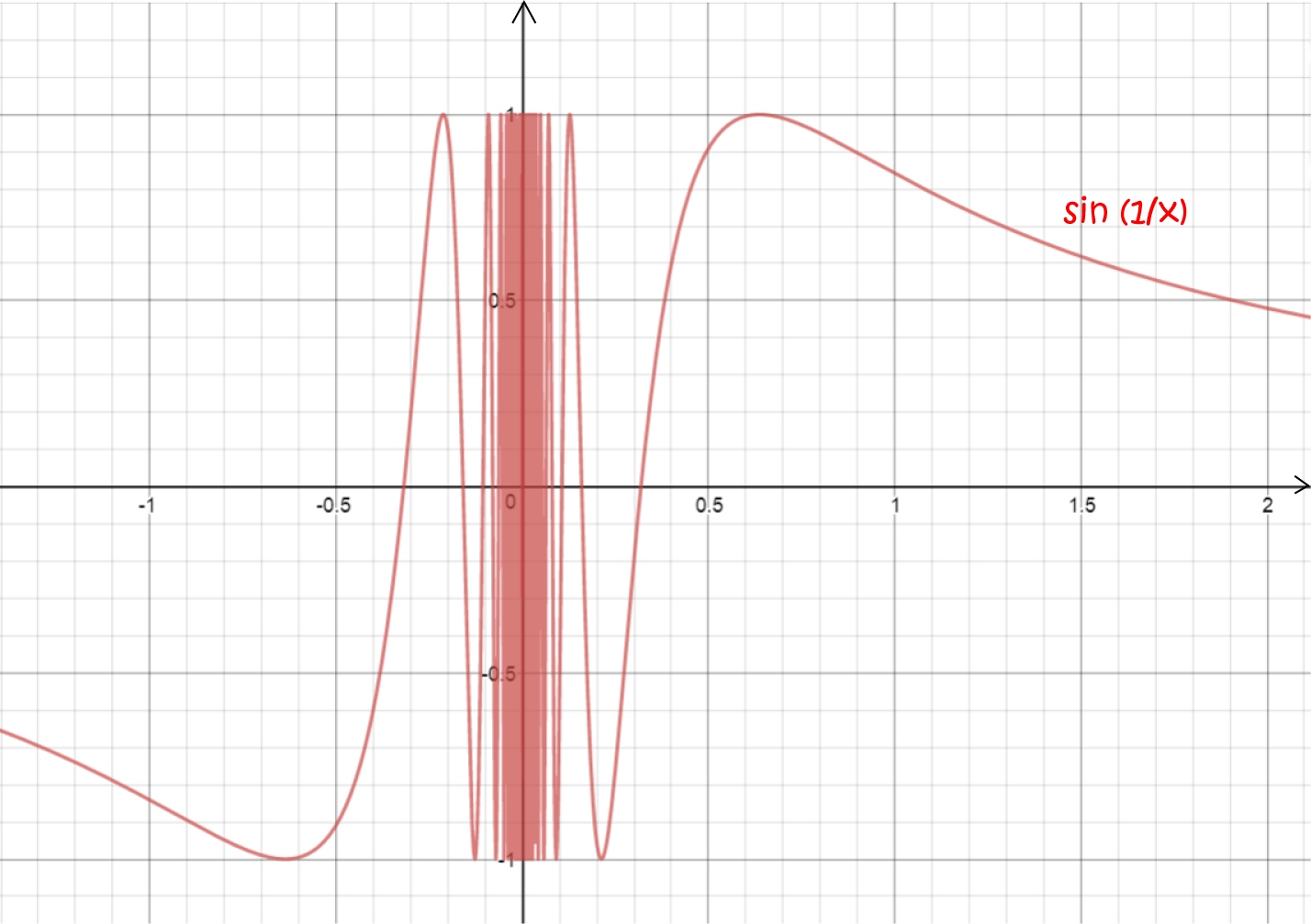
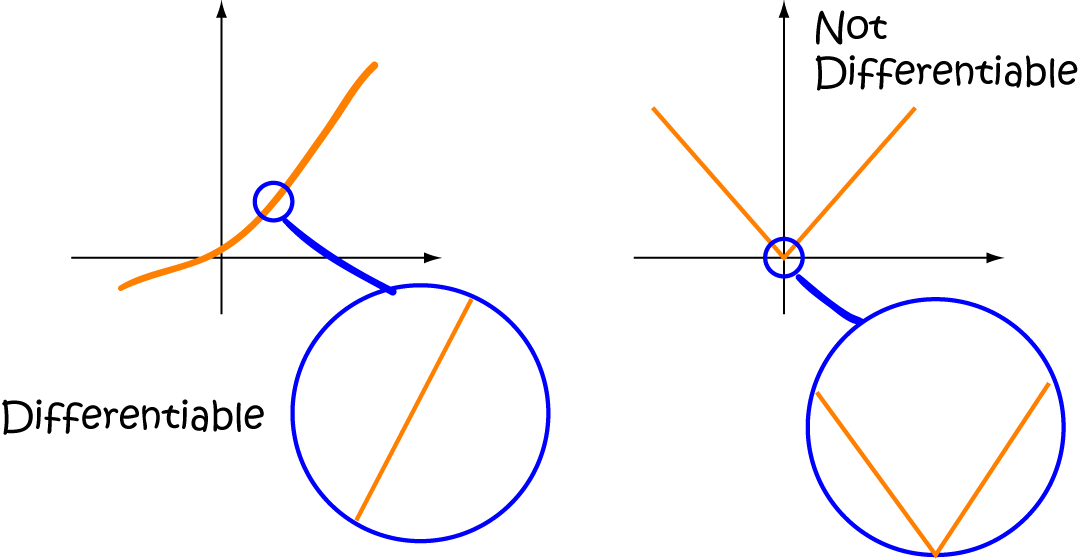
Differentiable In Calculus - We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable.
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to.
Use the total differential to. Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
What does it mean for a function to be differentiable? Calculus
Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Use the total differential to.
Differentiable function Wikiwand
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to.
What does it mean for a function to be differentiable? Calculus
We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable.
DefinitionCalculus TopicsDifferentiable Function Media4Math
Explain when a function of two variables is differentiable. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Use the total differential to. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is.
Differential Calculus Terms, Formulas, Rules, Examples
In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to.
What does it mean for a function to be differentiable? Calculus
Use the total differential to. Explain when a function of two variables is differentiable. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is.
What does it mean for a function to be differentiable? Calculus
We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to. Explain when a function of two variables is differentiable. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
What does it mean for a function to be differentiable? Calculus
Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. Use the total differential to. In calculus, a differentiable function is a continuous function whose derivative exists at all points on.
Differentiable vs. Continuous Functions Understanding the Distinctions
Use the total differential to. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is.
What does it mean for a function to be differentiable? Calculus
We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. Explain when a function of two variables is differentiable. Use the total differential to.
Use The Total Differential To.
Explain when a function of two variables is differentiable. In calculus, a differentiable function is a continuous function whose derivative exists at all points on. We studied differentials in section 4.4, where definition 18 states that if y = f(x) and f is.