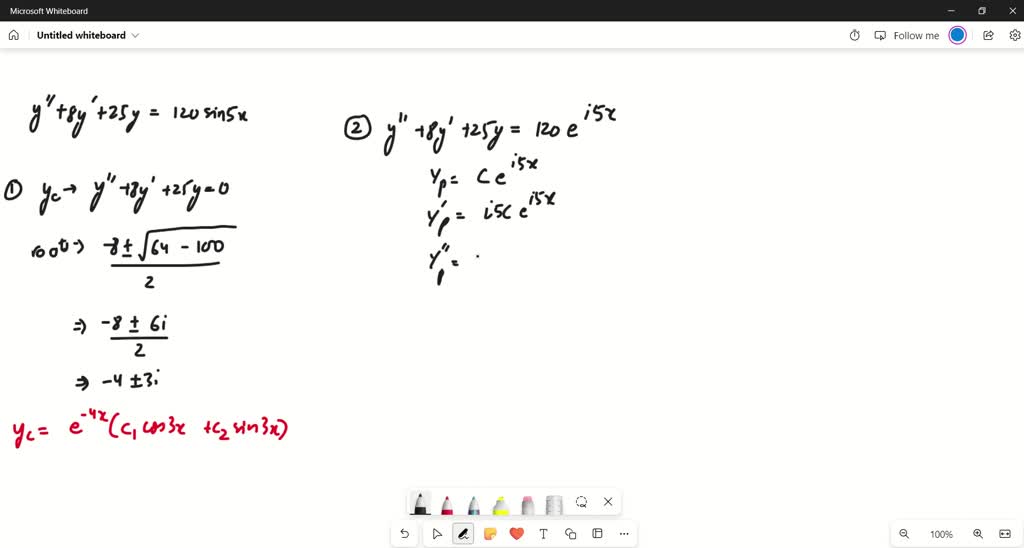
Differential Equations Complementary Solution - The complementary solution is only the solution to the homogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. A particular solution of a differential equation is a solution involving no unknown constants. In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation).
A particular solution of a differential equation is a solution involving no unknown constants. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. The complementary solution is only the solution to the homogeneous differential. In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation).
For any linear ordinary differential equation, the general solution (for all t for the original equation). In this section we will discuss the basics of solving nonhomogeneous differential. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. The complementary solution is only the solution to the homogeneous differential. A particular solution of a differential equation is a solution involving no unknown constants.
Differential Equations
The complementary solution is only the solution to the homogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. A particular solution of a differential equation is a solution involving no unknown constants. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. For any linear ordinary.
Solved For each of the given differential equations with the
A particular solution of a differential equation is a solution involving no unknown constants. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. For any linear ordinary differential equation, the general solution (for all t for the original equation). In this section we will discuss the basics of solving nonhomogeneous differential. Proof all we have to do is verify that if y.
[Solved] A nonhomogeneous differential equation, a complementary
For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. In this section we will discuss the basics of.
SOLVEDFind the general solution of the following differential
For any linear ordinary differential equation, the general solution (for all t for the original equation). A particular solution of a differential equation is a solution involving no unknown constants. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. In this section we will discuss.
SOLVEDFind the general solution of the following differential
The complementary solution is only the solution to the homogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation). Proof all we have to do is verify that if y is any solution.
[Solved] A nonhomogeneous differential equation, a complementary
The complementary solution is only the solution to the homogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. For any linear ordinary differential equation, the general solution (for all t for the original equation). Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. A particular.
Question Given The Differential Equation And The Complementary
The complementary solution is only the solution to the homogeneous differential. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. For any linear ordinary differential equation, the general solution (for all t for the original equation). In this.
Differential Equations Complementary Mathematics Studocu
Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential. A particular solution of a differential equation is a solution involving no unknown constants.
[Solved] . 1. Find the general solution to the differential equation
Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential. In this section we will discuss the basics of solving nonhomogeneous differential. A particular solution of a differential equation is a solution involving no unknown constants.
Solved Given the differential equation and the complementary
A particular solution of a differential equation is a solution involving no unknown constants. Proof all we have to do is verify that if y is any solution of equation 1, then y yp is a solution of. The complementary solution is only the solution to the homogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general. For any linear ordinary.
Proof All We Have To Do Is Verify That If Y Is Any Solution Of Equation 1, Then Y Yp Is A Solution Of.
A particular solution of a differential equation is a solution involving no unknown constants. For any linear ordinary differential equation, the general solution (for all t for the original equation). In this section we will discuss the basics of solving nonhomogeneous differential. Given a differential equation, [latex]y''+p(t)y'+q(t)y=g(t)[/latex], the general.