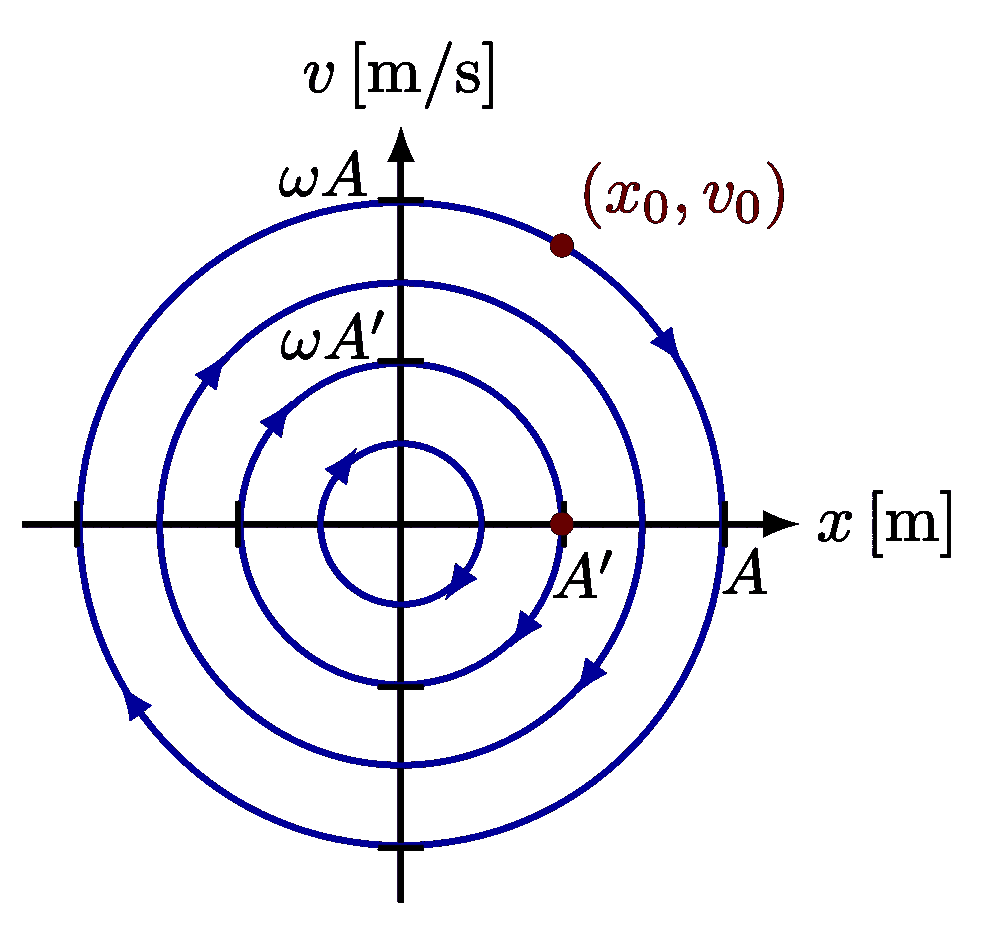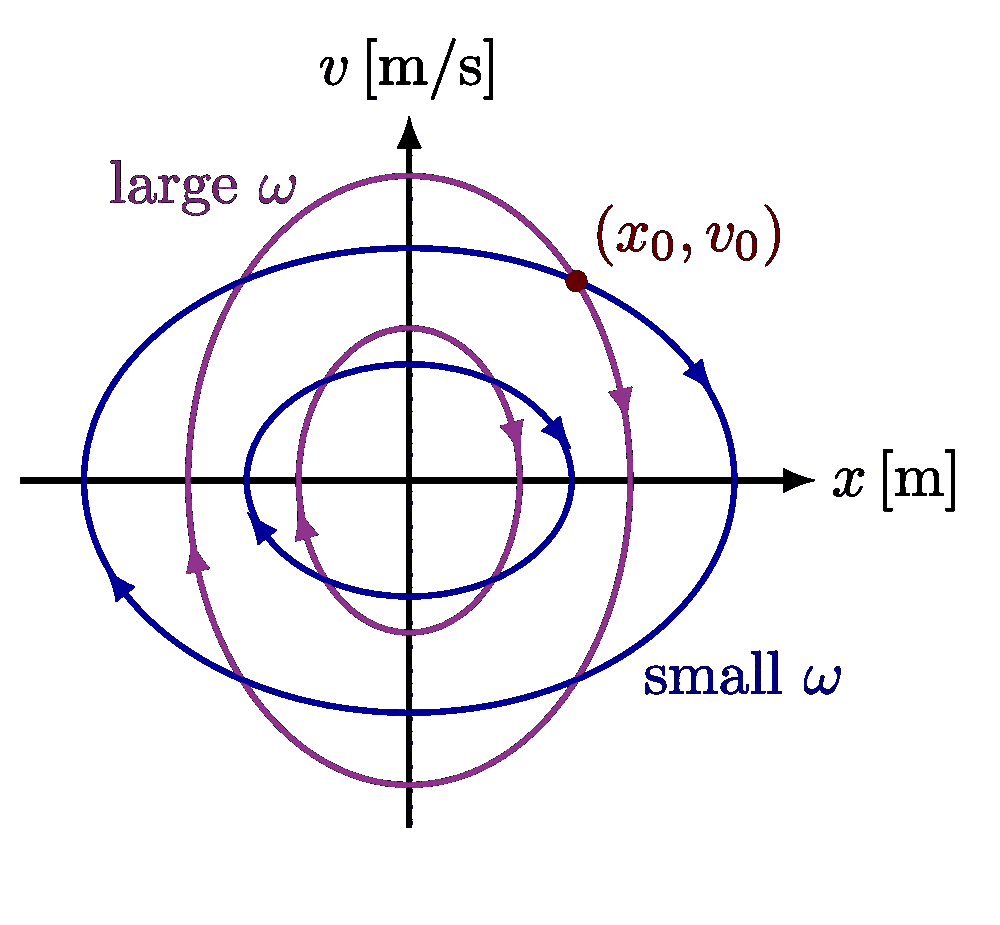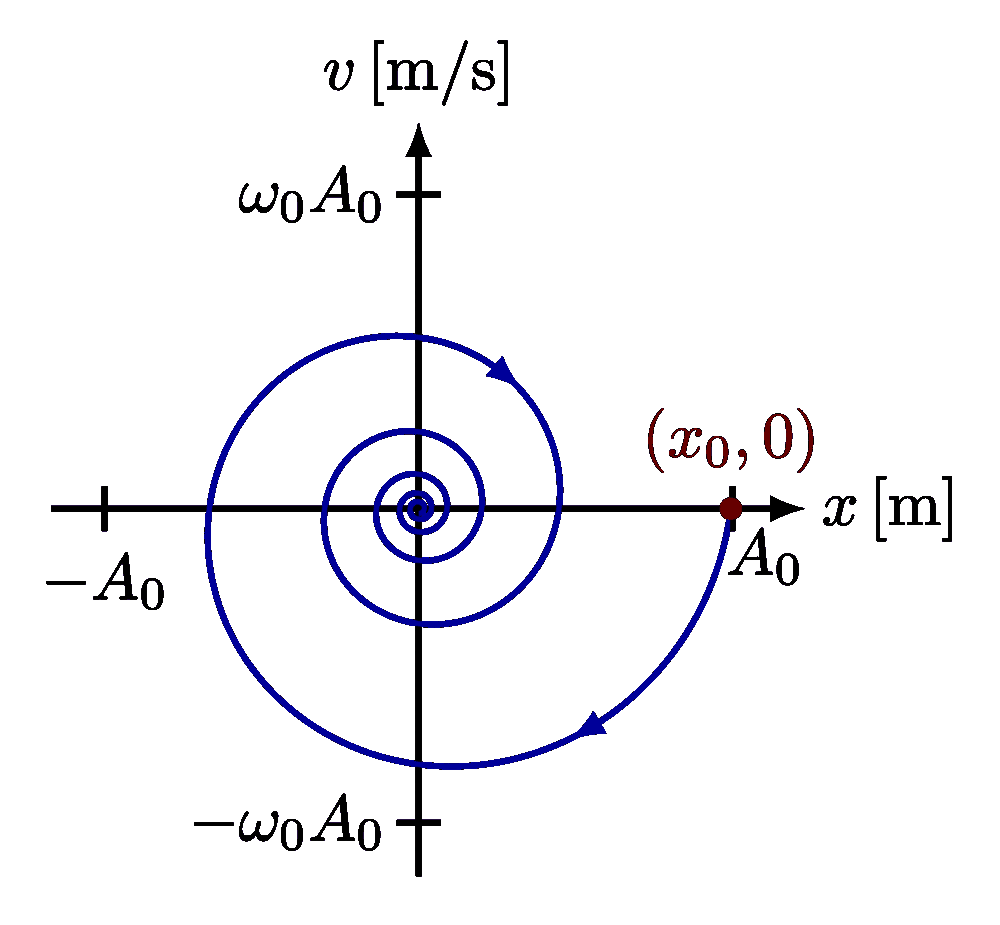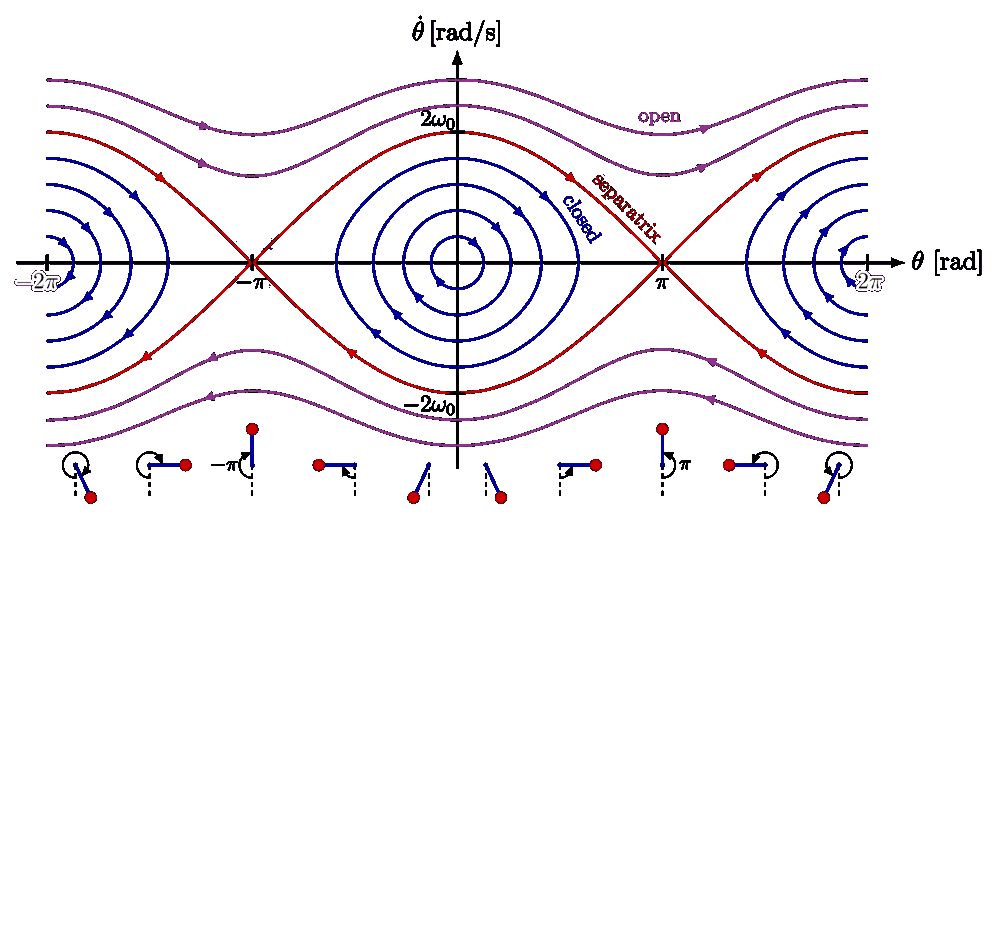
Differential Equations Springs - To determine the differential equation describing oscillations of the mass, we analyze the forces. Frictionless, unforced spring# suppose that we have a ball. Equations of spring motion# 2.1. The general solution of the differential equation is. We saw one involving a. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. We want to find all the forces on.
Frictionless, unforced spring# suppose that we have a ball. We want to find all the forces on. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. The general solution of the differential equation is. We saw one involving a. To determine the differential equation describing oscillations of the mass, we analyze the forces. Equations of spring motion# 2.1.
We saw one involving a. The general solution of the differential equation is. Equations of spring motion# 2.1. Frictionless, unforced spring# suppose that we have a ball. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. To determine the differential equation describing oscillations of the mass, we analyze the forces. We want to find all the forces on.
/aae14611c6e6e45b3bd99783f827f01c.jpg)
Textbooks Differential Equations Freeup
To determine the differential equation describing oscillations of the mass, we analyze the forces. Frictionless, unforced spring# suppose that we have a ball. We want to find all the forces on. Equations of spring motion# 2.1. We saw one involving a.
differential equations
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. Equations of spring motion# 2.1. The general solution of the differential equation is. To determine the differential equation describing oscillations of the mass, we analyze the forces. We want to find all the forces on.
[Solved] . Applications of Second Order EquationsSprings Damped
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. The general solution of the differential equation is. Equations of spring motion# 2.1. To determine the differential equation describing oscillations of the mass, we analyze the forces. We want to find all the forces on.
[Solved] . Applications of Second Order EquationsSprings Damped
Equations of spring motion# 2.1. We saw one involving a. Frictionless, unforced spring# suppose that we have a ball. To determine the differential equation describing oscillations of the mass, we analyze the forces. The general solution of the differential equation is.
-musIHb.png)
Practice FirstOrder Equations Brilliant
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. To determine the differential equation describing oscillations of the mass, we analyze the forces. The general solution of the differential equation is. Equations of spring motion# 2.1. We saw one involving a.
differential equations
The general solution of the differential equation is. Equations of spring motion# 2.1. Frictionless, unforced spring# suppose that we have a ball. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. To determine the differential equation describing oscillations of the mass, we analyze the forces.
differential equations
Frictionless, unforced spring# suppose that we have a ball. Equations of spring motion# 2.1. We want to find all the forces on. We saw one involving a. The general solution of the differential equation is.
Differential Equations Tutoring Costa Comprehensive Tutoring
Equations of spring motion# 2.1. We saw one involving a. The general solution of the differential equation is. We want to find all the forces on. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot.
Practice Differential Equations I Brilliant
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. We saw one involving a. Equations of spring motion# 2.1. We want to find all the forces on. Frictionless, unforced spring# suppose that we have a ball.
[Solved] . Applications of Second Order EquationsSprings Damped
We want to find all the forces on. Frictionless, unforced spring# suppose that we have a ball. Equations of spring motion# 2.1. The general solution of the differential equation is. We saw one involving a.
We Want To Find All The Forces On.
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot. Frictionless, unforced spring# suppose that we have a ball. We saw one involving a. To determine the differential equation describing oscillations of the mass, we analyze the forces.
Equations Of Spring Motion# 2.1.
The general solution of the differential equation is.