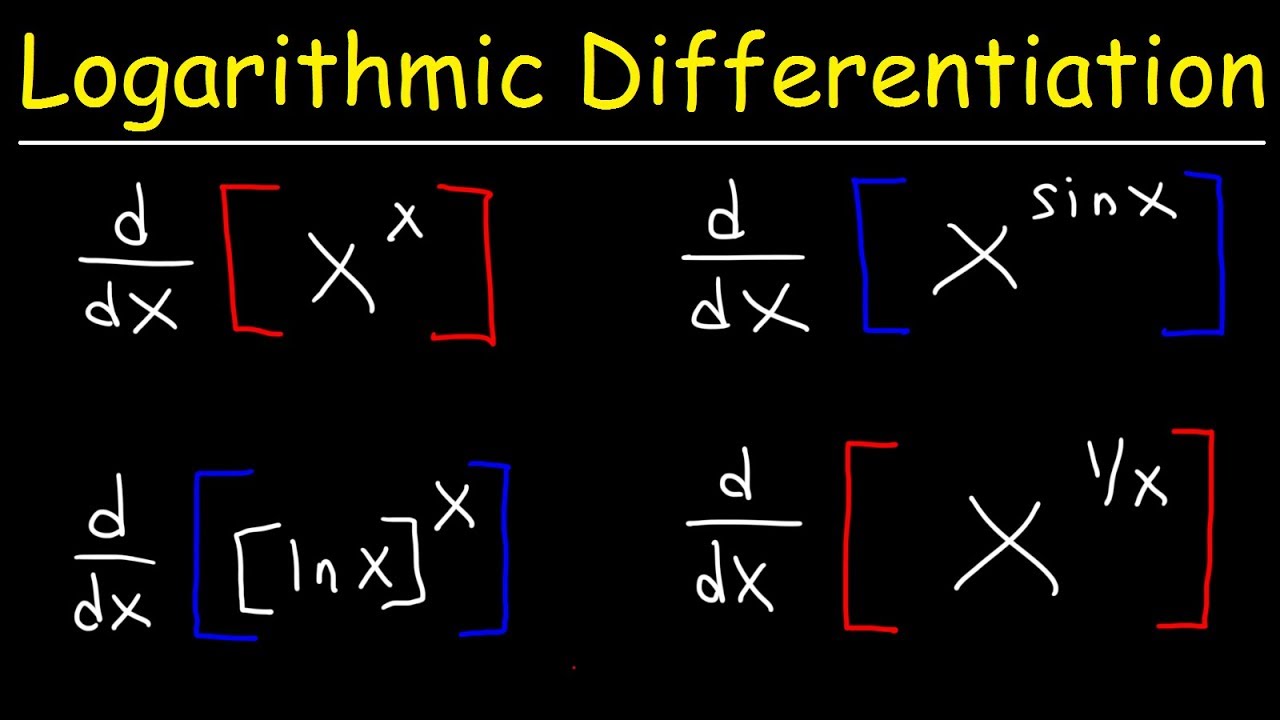
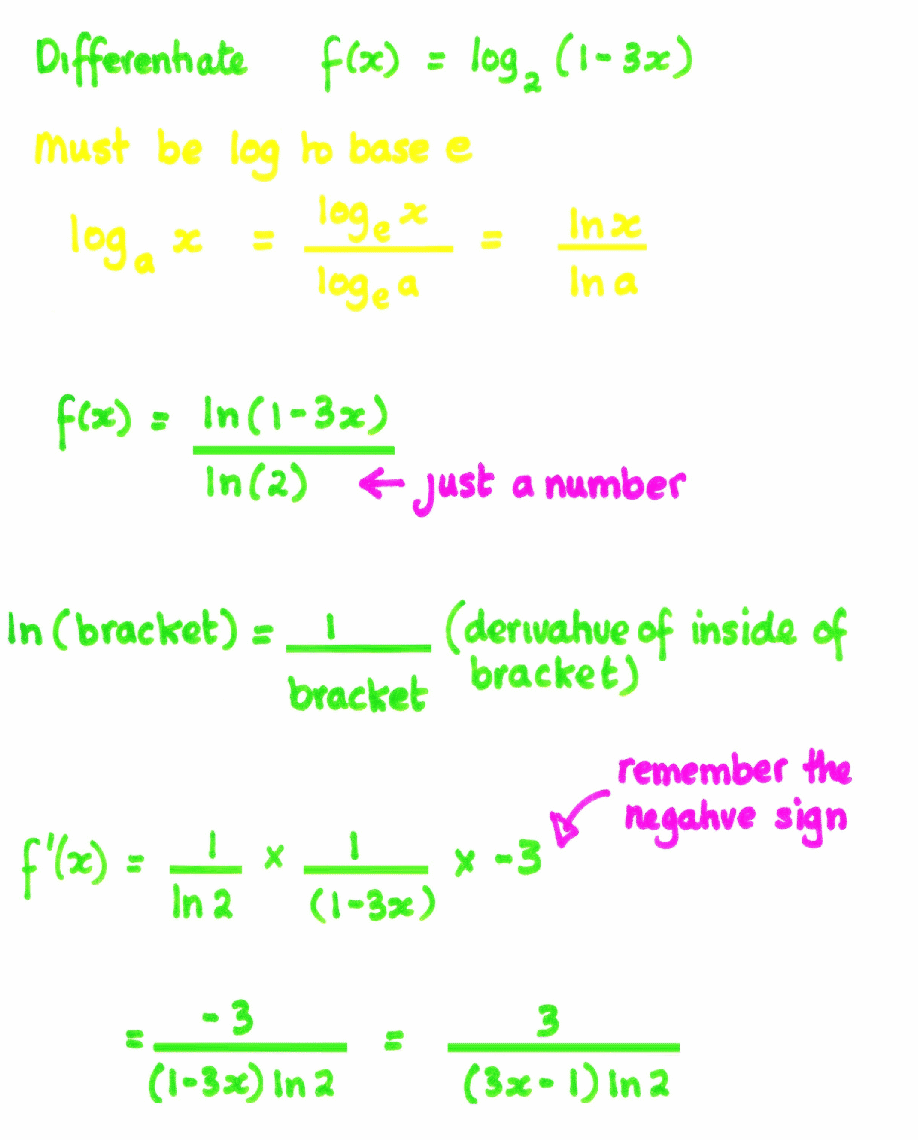Differentiate Logs - Logarithmic differentiation gives an alternative method for. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. In this section we will discuss logarithmic differentiation. However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Derivatives of logarithmic functions are mainly based on the chain rule.
Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. In this section we will discuss logarithmic differentiation. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation gives an alternative method for.
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Logarithmic differentiation gives an alternative method for. Derivatives of logarithmic functions are mainly based on the chain rule. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. However, we can generalize it for any differentiable function with. In this section we will discuss logarithmic differentiation.
Ex 5.5, 7 Differentiate the function (log x)^x + x^log x
However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation gives an alternative method for. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. The derivative of log x is 1/(x ln 10) and.
Differentiate Ln X
Derivatives of logarithmic functions are mainly based on the chain rule. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation gives an alternative method for. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of.
Using Logs to help differentiate
However, we can generalize it for any differentiable function with. In this section we will discuss logarithmic differentiation. Logarithmic differentiation gives an alternative method for. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Derivatives of logarithmic functions are mainly based on the chain rule.
Ex 5.5, 7 Differentiate the function (log x)^x + x^log x
The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a.
HOW TO DIFFERENTIATE USING LOG
In this section we will discuss logarithmic differentiation. However, we can generalize it for any differentiable function with. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. Logarithmic differentiation gives an alternative method for. Logarithmic differentiation allows us to.
Ex 5.4, 8 Differentiate log (log x) Chapter 5 Class 12
Derivatives of logarithmic functions are mainly based on the chain rule. Logarithmic differentiation gives an alternative method for. In this section we will discuss logarithmic differentiation. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. The derivative of log x is 1/(x ln 10) and the derivative of log x with base.
Differentiate Ln X
Derivatives of logarithmic functions are mainly based on the chain rule. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. In this section we will discuss logarithmic differentiation. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\).
Differentiate Ln X
Logarithmic differentiation gives an alternative method for. Derivatives of logarithmic functions are mainly based on the chain rule. The derivative of log x is 1/(x ln 10) and the derivative of log x with base a is 1/(x ln a) and the derivative of ln x is 1/x. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or.
Derivatives Natural Logs and Exponentials Task card activities, Task
Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Derivatives of logarithmic functions are mainly based on the chain rule. However, we can generalize it for any differentiable function with. Logarithmic differentiation gives an alternative method for. In this section we will discuss logarithmic differentiation.
Solved 2. Differentiate the functions (a) f(x) = logs (3 +
Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation gives an alternative method for. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain.
The Derivative Of Log X Is 1/(X Ln 10) And The Derivative Of Log X With Base A Is 1/(X Ln A) And The Derivative Of Ln X Is 1/X.
However, we can generalize it for any differentiable function with. In this section we will discuss logarithmic differentiation. Derivatives of logarithmic functions are mainly based on the chain rule. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by.
Logarithmic Differentiation Gives An Alternative Method For.
Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic.