Differentiate Sec 3X - The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. Differentiate both sides of the equation. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx. What is the derivative of y = sec3(x)? What is the derivative of y = sec3(x)? Differentiate the right side of the equation. The derivative of y y with respect to x x is y' y ′. The solution is, for problems like these, y = f. Thus, sec3x is an equivalent statement to 1 (cosx)3.
Thus, sec3x is an equivalent statement to 1 (cosx)3. The solution is, for problems like these, y = f. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). Differentiate the right side of the equation. The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. Secx is equal to 1 cosx. What is the derivative of y = sec3(x)? What is the derivative of y = sec3(x)? The derivative of y y with respect to x x is y' y ′. Differentiate both sides of the equation.
Differentiate both sides of the equation. What is the derivative of y = sec3(x)? Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). What is the derivative of y = sec3(x)? Differentiate the right side of the equation. The derivative of y y with respect to x x is y' y ′. The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. Thus, sec3x is an equivalent statement to 1 (cosx)3. The solution is, for problems like these, y = f. Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx.
Solved 1. Differentiate sec x and csc x using quotient
Differentiate both sides of the equation. The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec.
Ex 5.2, 4 Differentiate sec (tan (root x)) Class 12 Finding deri
Differentiate the right side of the equation. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). The solution is, for problems like these, y = f. What is the derivative of.
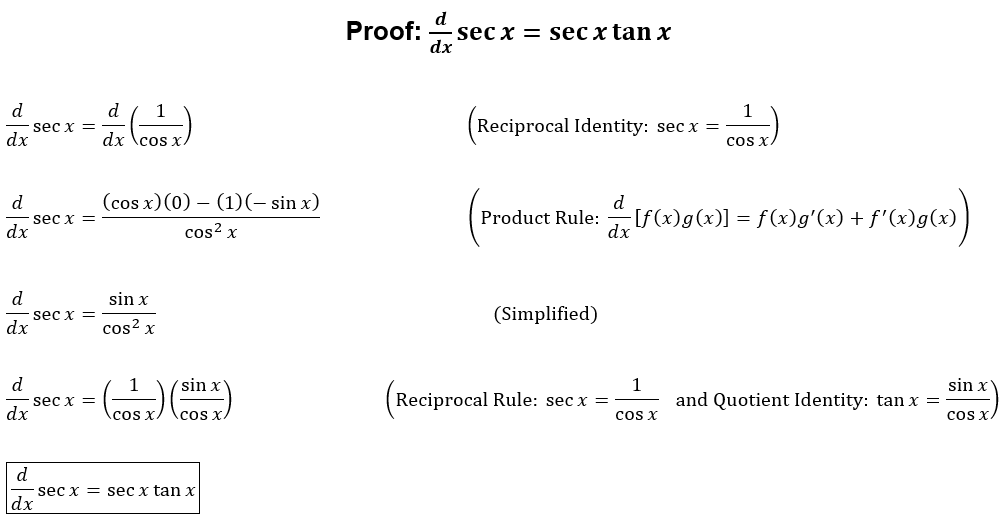
Brandi's Buzzar Blog Proof Derivative sec x = sec x tan x
Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx. Thus, sec3x is an equivalent statement to 1 (cosx)3. Secx is equal to 1 cosx. The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. The solution is, for problems like these, y = f.
[Solved] . 1 Let f(x) = Find f'(x). (+3 sec(3x2 2)) 3
Differentiate both sides of the equation. Secx is equal to 1 cosx. What is the derivative of y = sec3(x)? Differentiate the right side of the equation. Thus, sec3x is an equivalent statement to 1 (cosx)3.
Differentiate each of the following w.r.t. x sec^3 (x^2 + 1)
The solution is, for problems like these, y = f. What is the derivative of y = sec3(x)? Differentiate both sides of the equation. Secx is equal to 1 cosx. The derivative of y y with respect to x x is y' y ′.
65. Differentiate log sec x using first principle
The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. The derivative of y y with respect to x x is y' y ′. Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx. Secx is equal to 1 cosx. Differentiate the right side of the equation.
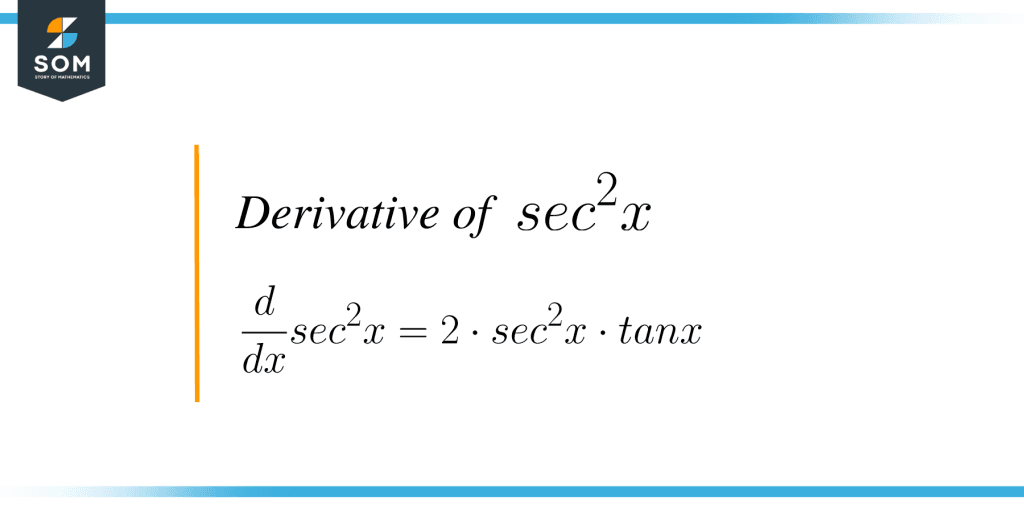
Derivative of Sec^2x Detailed Explanation and Examples The Story of
Thus, sec3x is an equivalent statement to 1 (cosx)3. Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx. Differentiate the right side of the equation. What is the derivative of y = sec3(x)? The solution is, for problems like these, y = f.
Derivative of Sec^2x Detailed Explanation and Examples The Story of
Thus, sec3x is an equivalent statement to 1 (cosx)3. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). What is the derivative of y = sec3(x)? Secx is equal to 1.
Differentiate sin(2x3) from first principles Maths Limits and
Secx is equal to 1 cosx. The solution is, for problems like these, y = f. What is the derivative of y = sec3(x)? Differentiate the right side of the equation. The derivative of y y with respect to x x is y' y ′.
Differentiate sec5x w.r.t. x using first principle.
Secx is equal to 1 cosx. Differentiate the right side of the equation. What is the derivative of y = sec3(x)? What is the derivative of y = sec3(x)? The derivative of y y with respect to x x is y' y ′.
The Derivative Of Y Y With Respect To X X Is Y' Y ′.
What is the derivative of y = sec3(x)? The solution is, for problems like these, y = f. The differentiation formula for the secant trigonometry ratio is given by \[\dfrac{d}{{dx}}(\sec (x)) = \sec (x).\tan (x)\],. Thus, sec3x is an equivalent statement to 1 (cosx)3.
Differentiate The Right Side Of The Equation.
Secx is equal to 1 cosx. Y' = 3 ⋅ sec2x ⋅ secx ⋅ tanx. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = sec(x) f (x) = sec (x). Differentiate both sides of the equation.