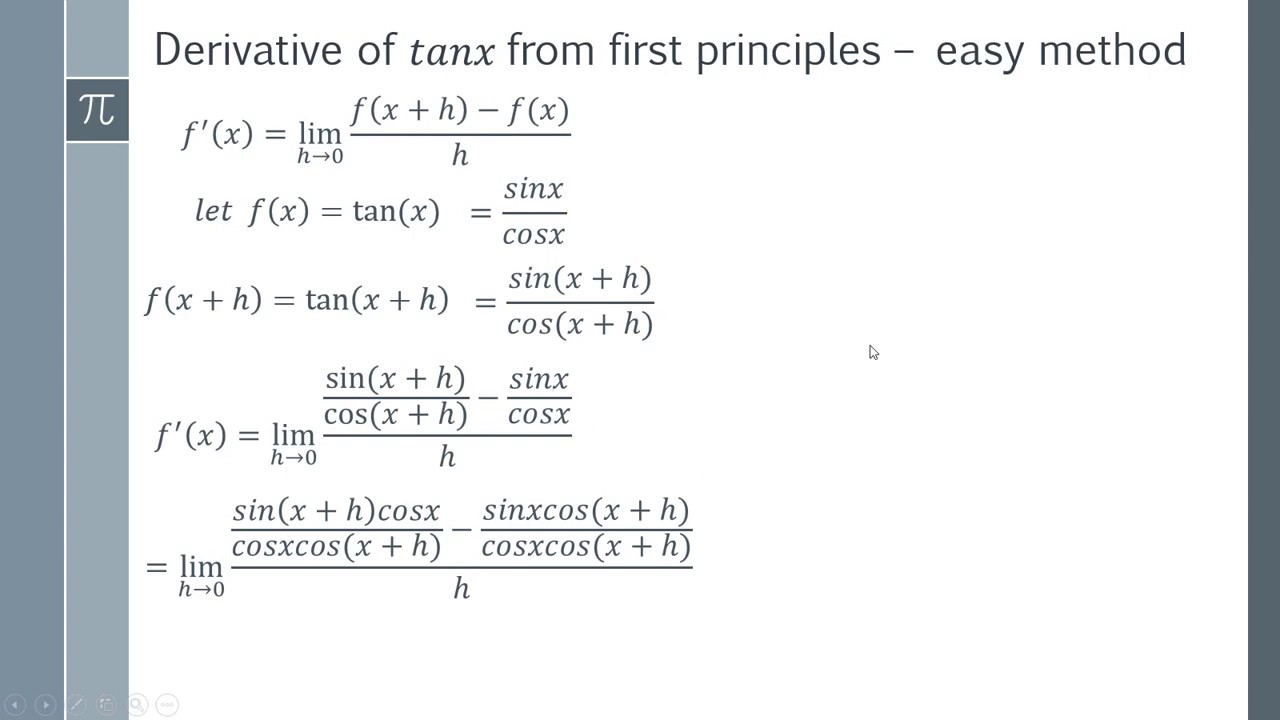
Differentiate Tan - Since tan x = sin x / cos x, we can replace the trigonometry identity with this. The function y=tan x can be differentiated easily. The derivative of tan x with respect to x is the square of sec x. Learn the derivative of tan x along with its proof and also see some examples using the same. All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x) = sin (x)/cos (x). Since we have a function divided by a function we can use the. We learn how to find the derivative of sin, cos and tan functions, and see some examples. I.e., d/dx (tan x) = sec^2 x. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule.
The function y=tan x can be differentiated easily. We learn how to find the derivative of sin, cos and tan functions, and see some examples. Since tan x = sin x / cos x, we can replace the trigonometry identity with this. The derivative of tan x with respect to x is the square of sec x. Learn the derivative of tan x along with its proof and also see some examples using the same. I.e., d/dx (tan x) = sec^2 x. Since we have a function divided by a function we can use the. All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x) = sin (x)/cos (x). The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule.
Since tan x = sin x / cos x, we can replace the trigonometry identity with this. I.e., d/dx (tan x) = sec^2 x. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule. Learn the derivative of tan x along with its proof and also see some examples using the same. Since we have a function divided by a function we can use the. All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x) = sin (x)/cos (x). The derivative of tan x with respect to x is the square of sec x. The function y=tan x can be differentiated easily. We learn how to find the derivative of sin, cos and tan functions, and see some examples.
Derivative of Tan^1 x Detailed Explanation and Examples The Story
Since tan x = sin x / cos x, we can replace the trigonometry identity with this. Since we have a function divided by a function we can use the. All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x).
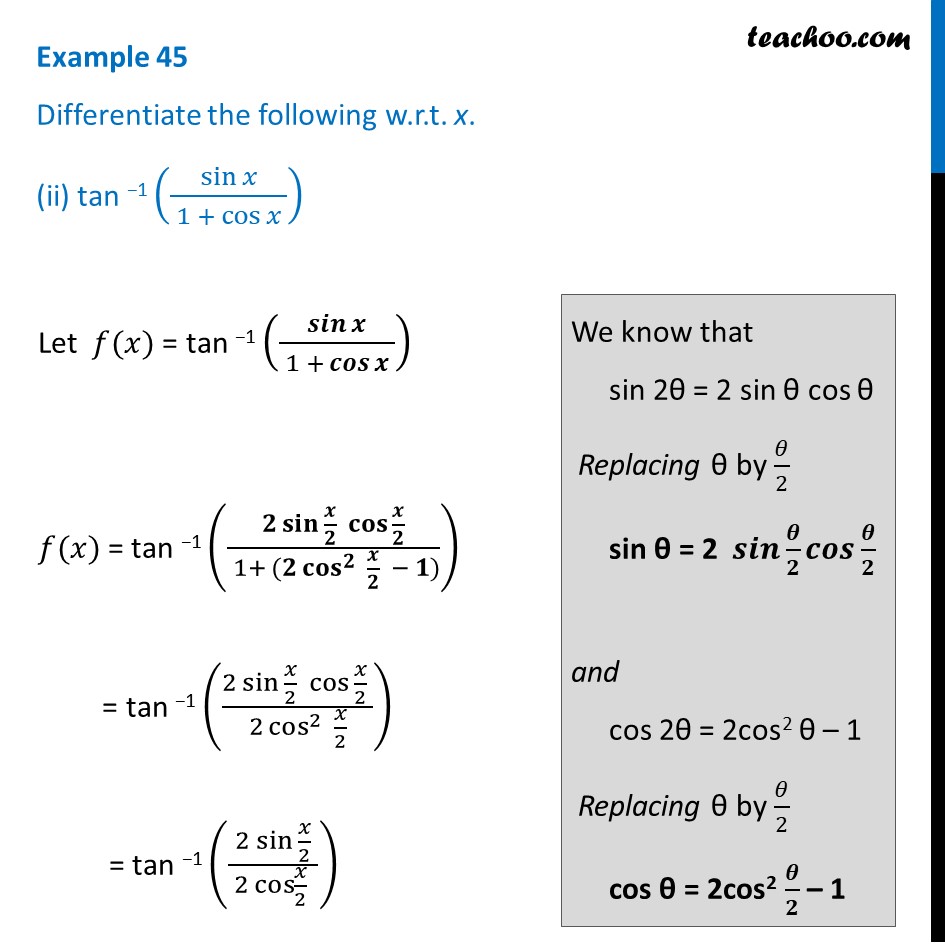
Example 45 (ii) Differentiate tan^1 (sin x/ (1 + cos x)) Teachoo
Since tan x = sin x / cos x, we can replace the trigonometry identity with this. The function y=tan x can be differentiated easily. Learn the derivative of tan x along with its proof and also see some examples using the same. Since we have a function divided by a function we can use the. We learn how to.
Derivative of tan(x) from first principles YouTube
I.e., d/dx (tan x) = sec^2 x. The function y=tan x can be differentiated easily. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule. Since tan x = sin x / cos x, we can replace the trigonometry identity with this..
Differentiating inverse tan(x/a) ExamSolutions Maths Revision YouTube
Since we have a function divided by a function we can use the. We learn how to find the derivative of sin, cos and tan functions, and see some examples. Learn the derivative of tan x along with its proof and also see some examples using the same. The function y=tan x can be differentiated easily. All derivatives of circular.
What is the Derivative of tan(x)? [FULL SOLUTION]
The function y=tan x can be differentiated easily. Learn the derivative of tan x along with its proof and also see some examples using the same. I.e., d/dx (tan x) = sec^2 x. Since tan x = sin x / cos x, we can replace the trigonometry identity with this. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2.
Derivative of tangent x, sec x & tan x Longer Free Tutorial Get
Since we have a function divided by a function we can use the. Learn the derivative of tan x along with its proof and also see some examples using the same. We learn how to find the derivative of sin, cos and tan functions, and see some examples. The function y=tan x can be differentiated easily. The derivative of tan.
How to Find the Derivative of tanx from First Principles YouTube
We learn how to find the derivative of sin, cos and tan functions, and see some examples. Since we have a function divided by a function we can use the. The function y=tan x can be differentiated easily. The derivative of tan x with respect to x is the square of sec x. All derivatives of circular trigonometric functions can.
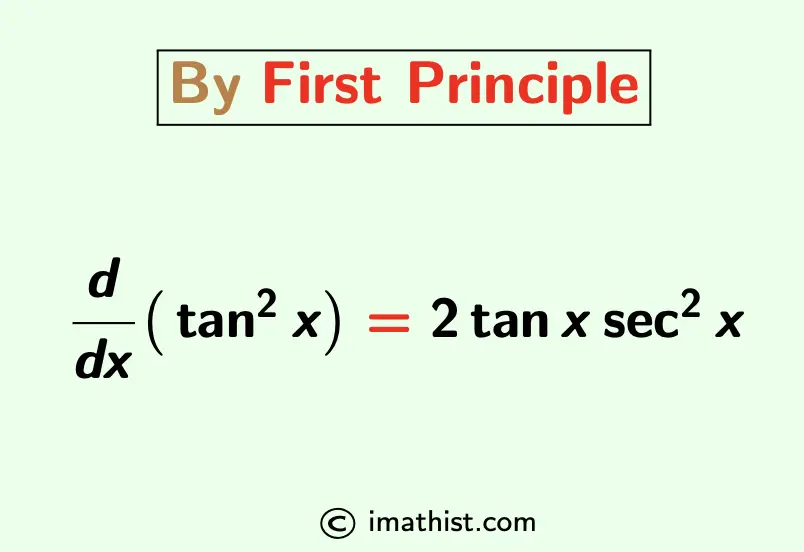
Derivative of tan^2x by First Principle iMath
The function y=tan x can be differentiated easily. Since we have a function divided by a function we can use the. All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x) = sin (x)/cos (x). We learn how to find.
Derivative of Tan Differentiation & Formula Lesson
Learn the derivative of tan x along with its proof and also see some examples using the same. The function y=tan x can be differentiated easily. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule. I.e., d/dx (tan x) = sec^2.
Derivative of Tangent x Formula, Rules, Examples
All derivatives of circular trigonometric functions can be found from those of sin (x) and cos (x) by means of the quotient rule applied to functions such as tan (x) = sin (x)/cos (x). I.e., d/dx (tan x) = sec^2 x. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods.
We Learn How To Find The Derivative Of Sin, Cos And Tan Functions, And See Some Examples.
Since we have a function divided by a function we can use the. Learn the derivative of tan x along with its proof and also see some examples using the same. Since tan x = sin x / cos x, we can replace the trigonometry identity with this. The function y=tan x can be differentiated easily.
All Derivatives Of Circular Trigonometric Functions Can Be Found From Those Of Sin (X) And Cos (X) By Means Of The Quotient Rule Applied To Functions Such As Tan (X) = Sin (X)/Cos (X).
I.e., d/dx (tan x) = sec^2 x. The derivative of tan (𝑥)tan (x) is sec2 (𝑥)sec 2 (x), and it can be derived using several methods including the limit definition, quotient rule, and chain rule. The derivative of tan x with respect to x is the square of sec x.
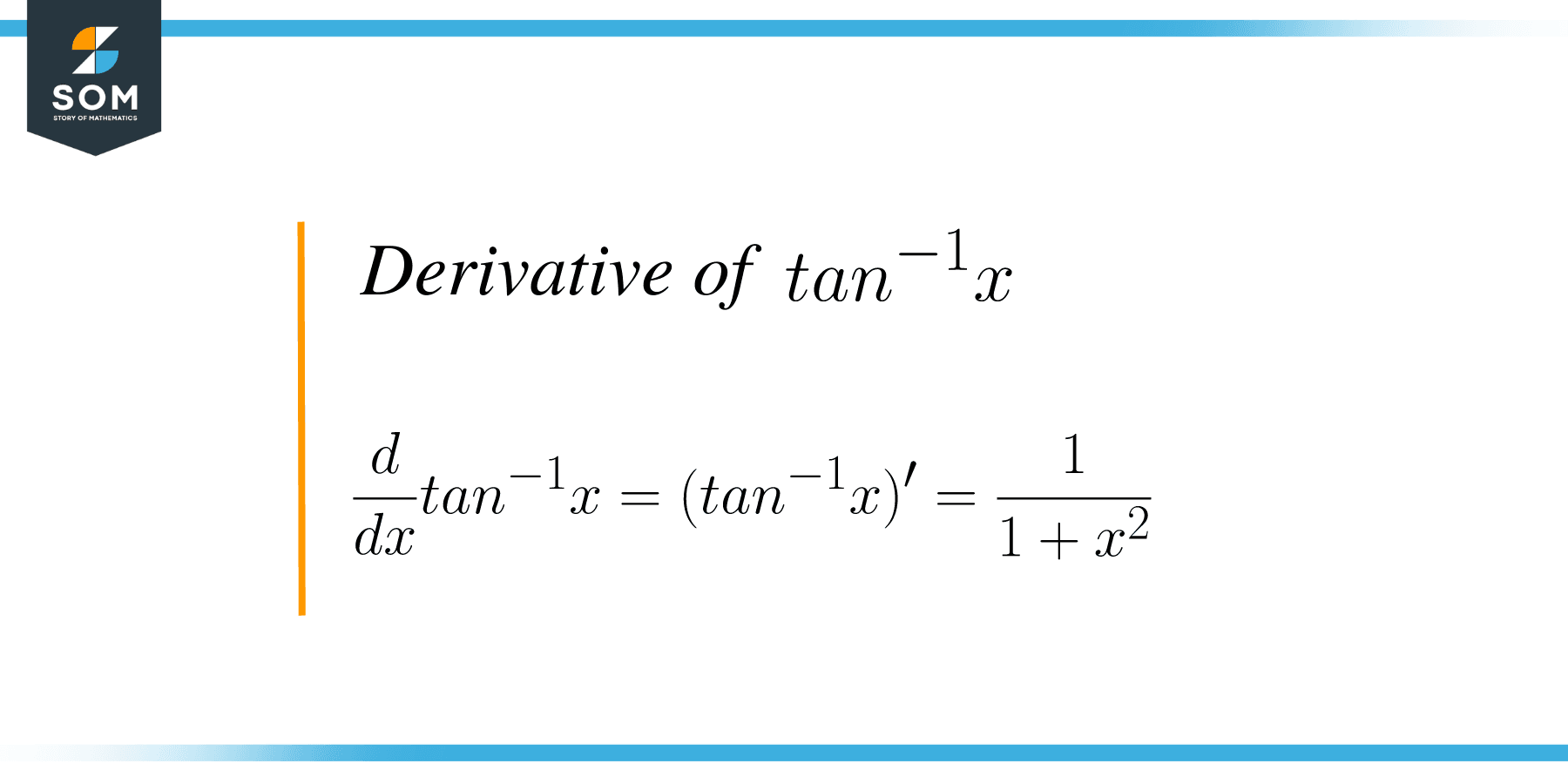

![What is the Derivative of tan(x)? [FULL SOLUTION]](https://www.epsilonify.com/wp-content/uploads/2022/09/derivative-of-tanx-1024x576.png)