Differentiate Vs Derivative - I hope this answers your question. The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. It's the same as the difference between 'product' and 'production',. In general, is a function. $\begingroup$ the derivative of a function in a point is a number; We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. This is more a matter of language than mathematics. A derivative is the change in a function ($\frac{dy}{dx}$); A derivative is what you get as a result of differentiation. The process of finding [computing] a derivative is called differentiation.
The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. I hope this answers your question. A derivative is the change in a function ($\frac{dy}{dx}$); In general, is a function. $\begingroup$ the derivative of a function in a point is a number; The process of finding [computing] a derivative is called differentiation. This is more a matter of language than mathematics. A derivative is what you get as a result of differentiation. We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. It's the same as the difference between 'product' and 'production',.
The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. In general, is a function. $\begingroup$ the derivative of a function in a point is a number; This is more a matter of language than mathematics. A derivative is what you get as a result of differentiation. A derivative is the change in a function ($\frac{dy}{dx}$); It's the same as the difference between 'product' and 'production',. We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. The process of finding [computing] a derivative is called differentiation. I hope this answers your question.
What Is A Derivative? Definition And Example
The process of finding [computing] a derivative is called differentiation. This is more a matter of language than mathematics. We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. A derivative is what you get as a result of differentiation. It's the same as the difference between.
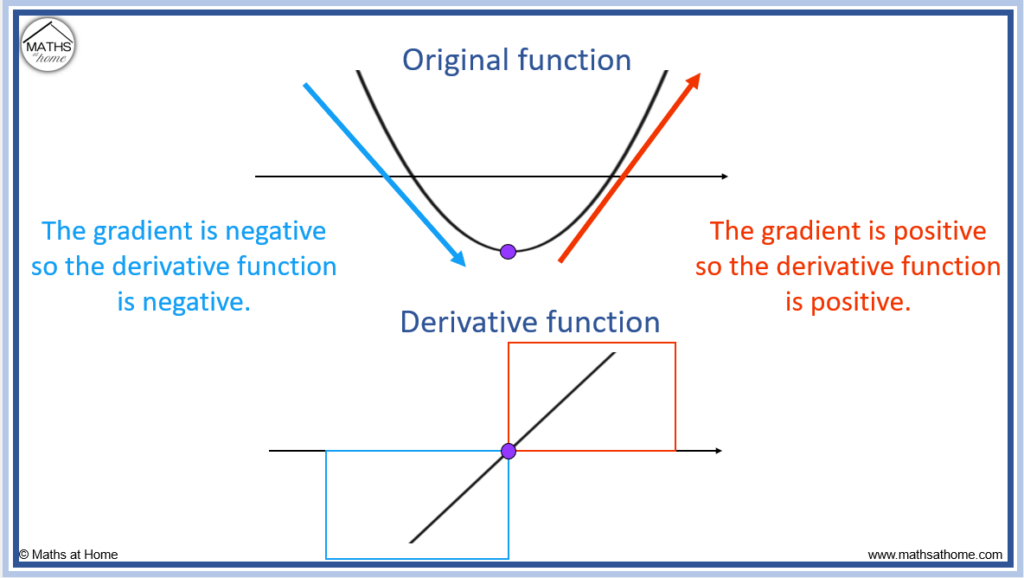
How to Sketch the Graph of the Derivative
This is more a matter of language than mathematics. A derivative is the change in a function ($\frac{dy}{dx}$); The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. A derivative is what you get as a result of differentiation. It's the same as the difference.
Calculus Worksheets Differentiation Rules Worksheets Worksheets Library
In general, is a function. $\begingroup$ the derivative of a function in a point is a number; A derivative is the change in a function ($\frac{dy}{dx}$); We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. The partial derivative notation is used to specify the derivative of.
Integrate vs. differentiate Math examples
A derivative is what you get as a result of differentiation. The process of finding [computing] a derivative is called differentiation. The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. I hope this answers your question. We can also define a derivative in terms.
How to Sketch the Graph of the Derivative
A derivative is the change in a function ($\frac{dy}{dx}$); In general, is a function. The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. I hope this answers your question. The process of finding [computing] a derivative is called differentiation.
Derivative Instruments A Complete Guide I BalancingEverything
We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. It's the same as the difference between 'product' and 'production',. A derivative is the change in a function ($\frac{dy}{dx}$); $\begingroup$ the derivative of a function in a point is a number; The partial derivative notation is used.
Differential vs. Derivative — What’s the Difference?
This is more a matter of language than mathematics. It's the same as the difference between 'product' and 'production',. The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. We can also define a derivative in terms of differentials as the ratio of differentials of.
Differential vs Derivative When To Use Each One In Writing?
In general, is a function. I hope this answers your question. The process of finding [computing] a derivative is called differentiation. We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. This is more a matter of language than mathematics.
Differentiate vs. Distinguish What’s the Difference?
A derivative is the change in a function ($\frac{dy}{dx}$); We can also define a derivative in terms of differentials as the ratio of differentials of function by the differential of a variable. It's the same as the difference between 'product' and 'production',. This is more a matter of language than mathematics. I hope this answers your question.
Download Difference, Differentiate, Anders. RoyaltyFree Stock
The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. I hope this answers your question. $\begingroup$ the derivative of a function in a point is a number; We can also define a derivative in terms of differentials as the ratio of differentials of function.
The Process Of Finding [Computing] A Derivative Is Called Differentiation.
This is more a matter of language than mathematics. The partial derivative notation is used to specify the derivative of a function of more than one variable with respect to one of its variables. A derivative is the change in a function ($\frac{dy}{dx}$); It's the same as the difference between 'product' and 'production',.
We Can Also Define A Derivative In Terms Of Differentials As The Ratio Of Differentials Of Function By The Differential Of A Variable.
In general, is a function. I hope this answers your question. A derivative is what you get as a result of differentiation. $\begingroup$ the derivative of a function in a point is a number;