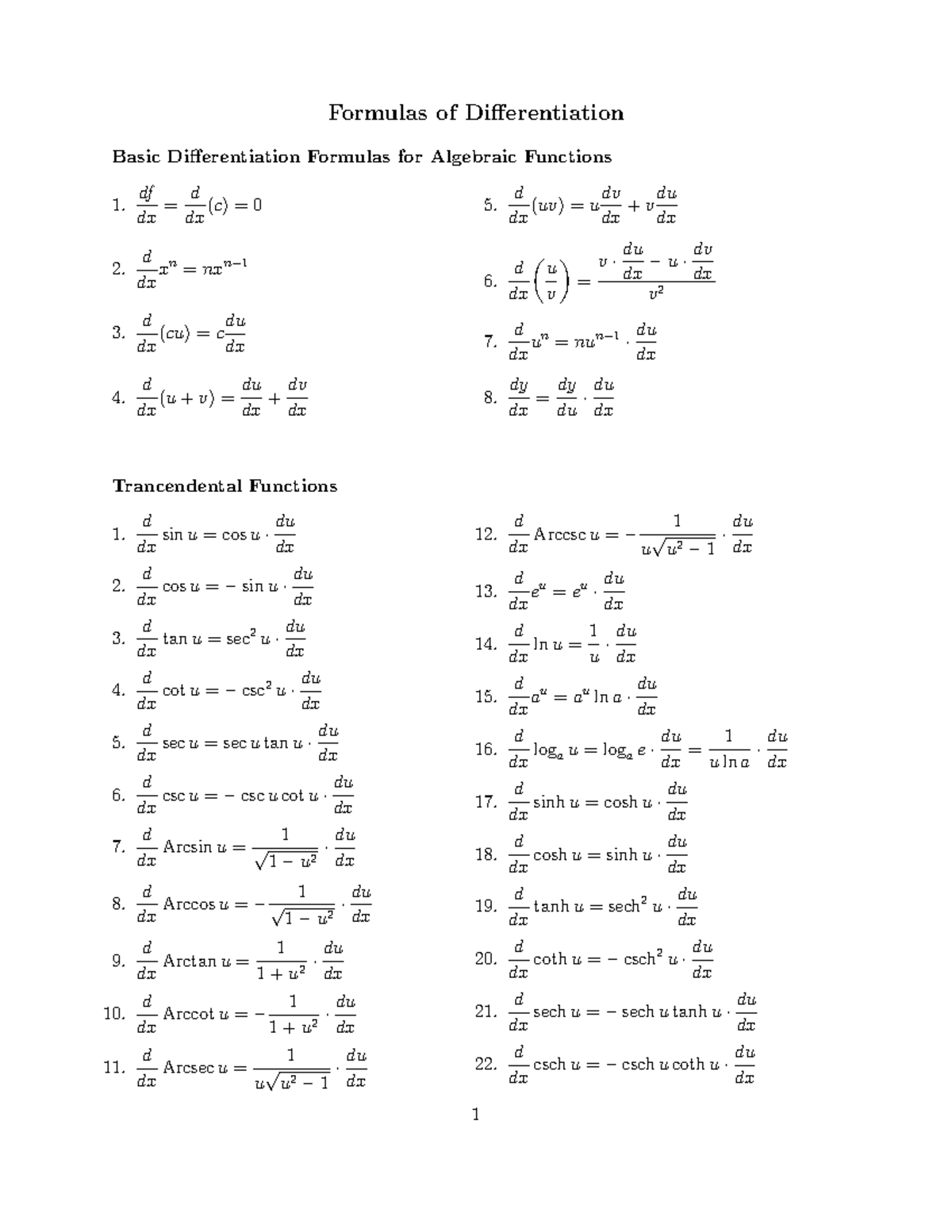
Differentiation And Integration Of Trigonometric Functions - How do i know which trig identities to use? A function y=f(x) is continuous at x=a if i. R strategy for evaluating sin: N (x)dx (a) if the 2power n of cosine is odd (n =2k. Two of the derivatives will be. Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. List of integrals of inverse trig functions; Note the difference between the ± and ∓ symbols! List of integrals of hyperbolic functions; Even and odd functions 1.
List of integrals of inverse trig functions; R strategy for evaluating sin: Note the difference between the ± and ∓ symbols! N (x)dx (a) if the 2power n of cosine is odd (n =2k. A function y=f(x) is continuous at x=a if i. List of integrals of hyperbolic functions; Even and odd functions 1. Two of the derivatives will be. We’ll start this process off by taking a look at the derivatives of the six trig functions. List of integrals of inverse hyperbolic functions;
Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. Even and odd functions 1. A function y=f(x) is continuous at x=a if i. We’ll start this process off by taking a look at the derivatives of the six trig functions. List of integrals of inverse trig functions; Note the difference between the ± and ∓ symbols! N (x)dx (a) if the 2power n of cosine is odd (n =2k. How do i know which trig identities to use? Two of the derivatives will be. List of integrals of hyperbolic functions;
integration formulas5 Trigonometric Identities
How do i know which trig identities to use? We’ll start this process off by taking a look at the derivatives of the six trig functions. A function y=f(x) is continuous at x=a if i. Note the difference between the ± and ∓ symbols! Even and odd functions 1.
Integration of Trigonometric Functions
Two of the derivatives will be. Even and odd functions 1. A function y=f(x) is continuous at x=a if i. N (x)dx (a) if the 2power n of cosine is odd (n =2k. Note the difference between the ± and ∓ symbols!
Integration of trigonometric functions. To solve use multiple angle formula
Two of the derivatives will be. We’ll start this process off by taking a look at the derivatives of the six trig functions. Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. Note the difference between the ± and ∓ symbols! N (x)dx (a) if the 2power n of cosine is.
(PDF) Mnemonics of Basic Differentiation and Integration for
How do i know which trig identities to use? Two of the derivatives will be. N (x)dx (a) if the 2power n of cosine is odd (n =2k. List of integrals of inverse hyperbolic functions; Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =.
Differentiation And Integration Formulas Of Trigonometric Functions
R strategy for evaluating sin: Note the difference between the ± and ∓ symbols! We’ll start this process off by taking a look at the derivatives of the six trig functions. A function y=f(x) is continuous at x=a if i. Two of the derivatives will be.
Integration of Trigonometric Functions
Note the difference between the ± and ∓ symbols! How do i know which trig identities to use? List of integrals of inverse hyperbolic functions; Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. A function y=f(x) is continuous at x=a if i.
(PDF) Mnemonics of Basic Differentiation and Integration for
List of integrals of inverse hyperbolic functions; Note the difference between the ± and ∓ symbols! List of integrals of inverse trig functions; A function y=f(x) is continuous at x=a if i. Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =.
Formulas Differentiation Integration Trigonometric Substitution
A function y=f(x) is continuous at x=a if i. List of integrals of inverse trig functions; Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. Even and odd functions 1. N (x)dx (a) if the 2power n of cosine is odd (n =2k.
Differentiation of Trigonometric Functions Trig Derivatives
List of integrals of hyperbolic functions; N (x)dx (a) if the 2power n of cosine is odd (n =2k. We’ll start this process off by taking a look at the derivatives of the six trig functions. Two of the derivatives will be. R strategy for evaluating sin:
Integration of Trigonometric Functions
Two of the derivatives will be. How do i know which trig identities to use? List of integrals of inverse trig functions; Recall from the definition of an antiderivative that, if $\frac{d}{dx} f(x) = g(x),$ then $\int g(x) dx =. N (x)dx (a) if the 2power n of cosine is odd (n =2k.
Even And Odd Functions 1.
A function y=f(x) is continuous at x=a if i. How do i know which trig identities to use? Note the difference between the ± and ∓ symbols! List of integrals of inverse hyperbolic functions;
Recall From The Definition Of An Antiderivative That, If $\Frac{D}{Dx} F(X) = G(X),$ Then $\Int G(X) Dx =.
N (x)dx (a) if the 2power n of cosine is odd (n =2k. List of integrals of hyperbolic functions; Two of the derivatives will be. We’ll start this process off by taking a look at the derivatives of the six trig functions.
R Strategy For Evaluating Sin:
List of integrals of inverse trig functions;