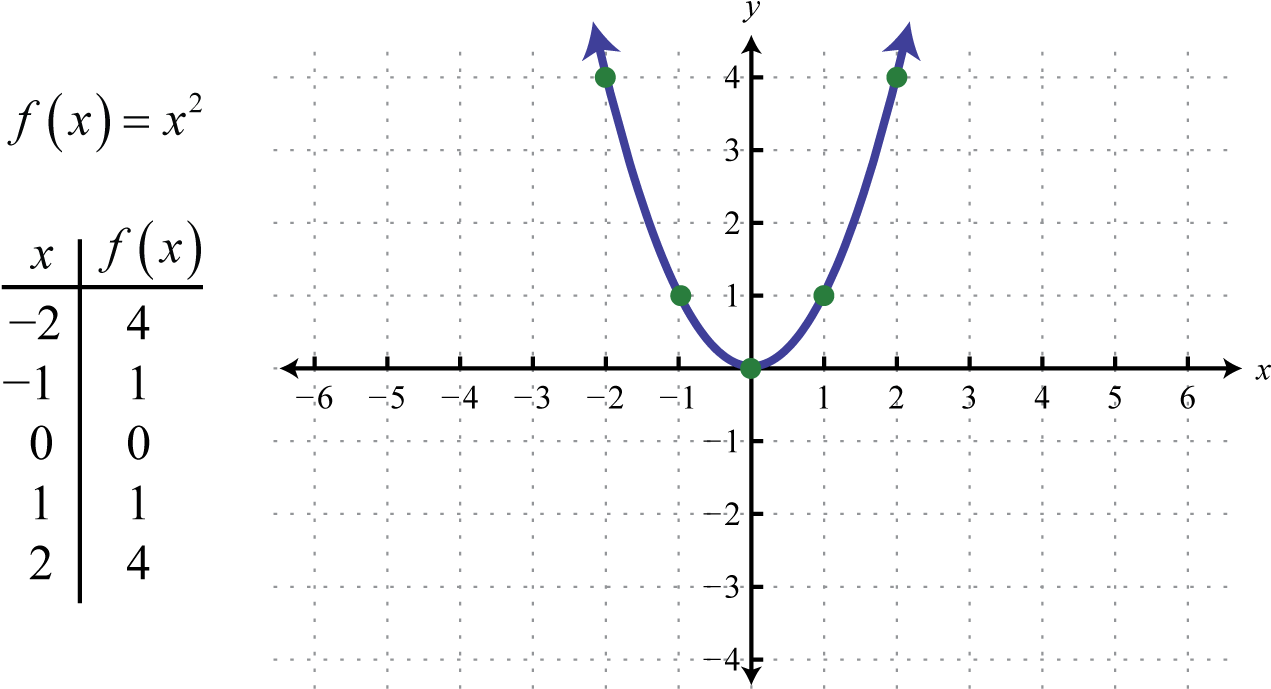
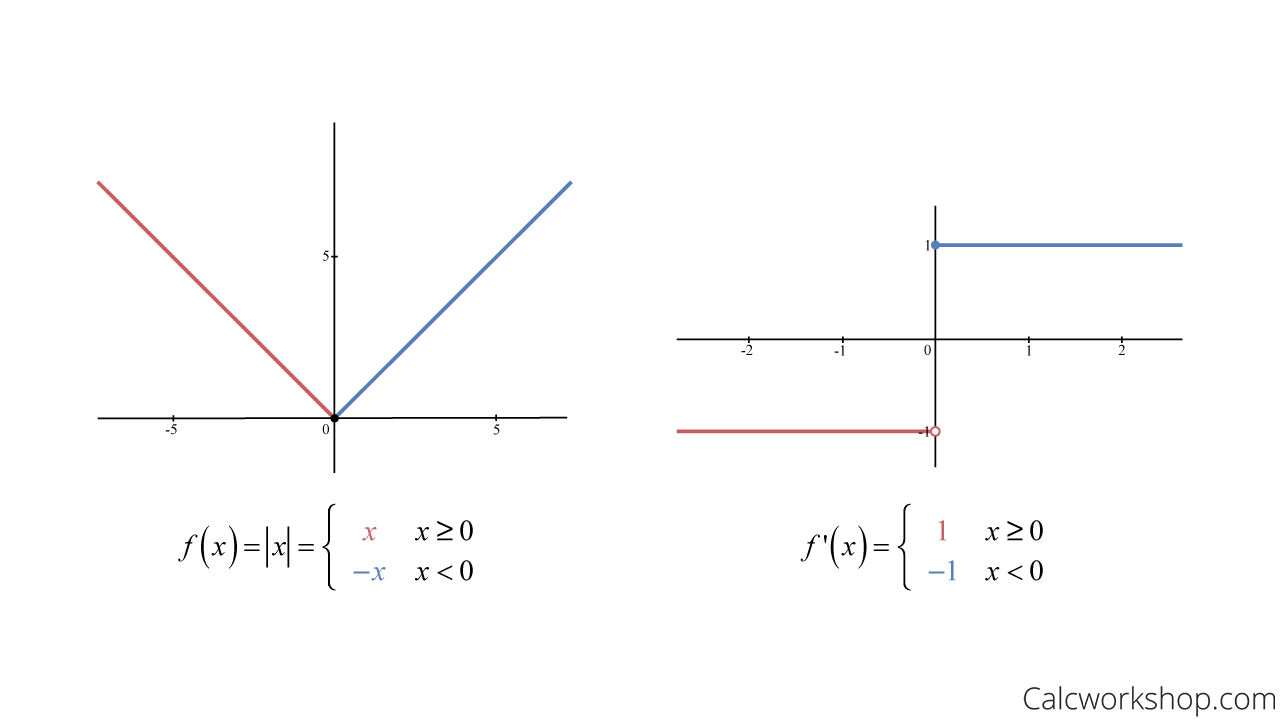
Differentiation Of Absolute Function - The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Let $\size x$ be the absolute value of $x$ for real $x$. The absolute value function | x | is defined. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. D dx |u| = u |u| ⋅ du dx. To understand the derivative of the absolute value function | x |, let’s break it down step by step. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Y' = 2(x −2) 2√(x − 2)2 → power rule. What is the derivative of an absolute value?
$\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Y' = 2(x −2) 2√(x − 2)2 → power rule. Let $\size x$ be the absolute value of $x$ for real $x$. What is the derivative of an absolute value? To understand the derivative of the absolute value function | x |, let’s break it down step by step. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. D dx |u| = u |u| ⋅ du dx. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. The absolute value function | x | is defined.
$\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. What is the derivative of an absolute value? Y' = 2(x −2) 2√(x − 2)2 → power rule. To understand the derivative of the absolute value function | x |, let’s break it down step by step. Let $\size x$ be the absolute value of $x$ for real $x$. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. D dx |u| = u |u| ⋅ du dx. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. The absolute value function | x | is defined.
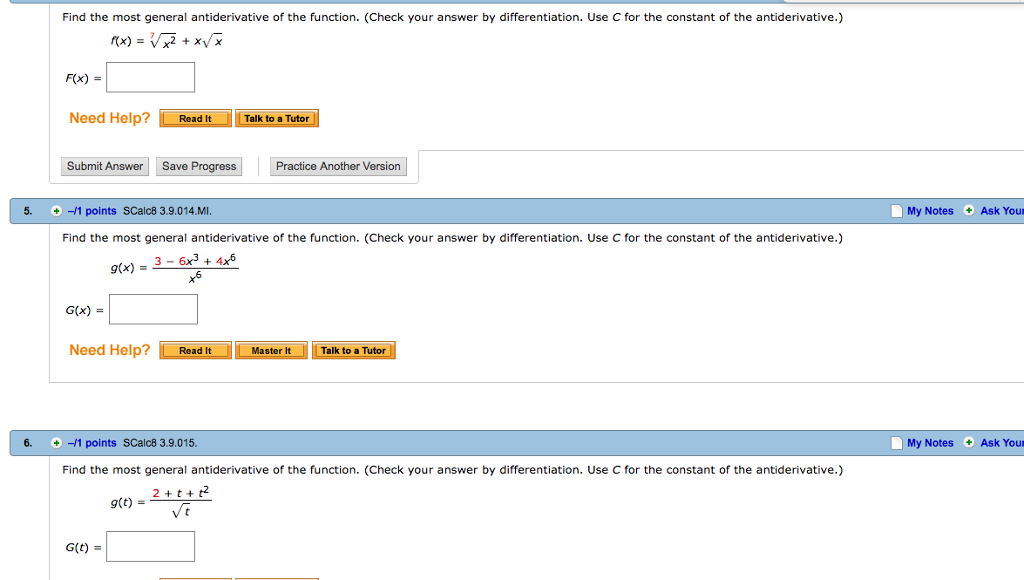
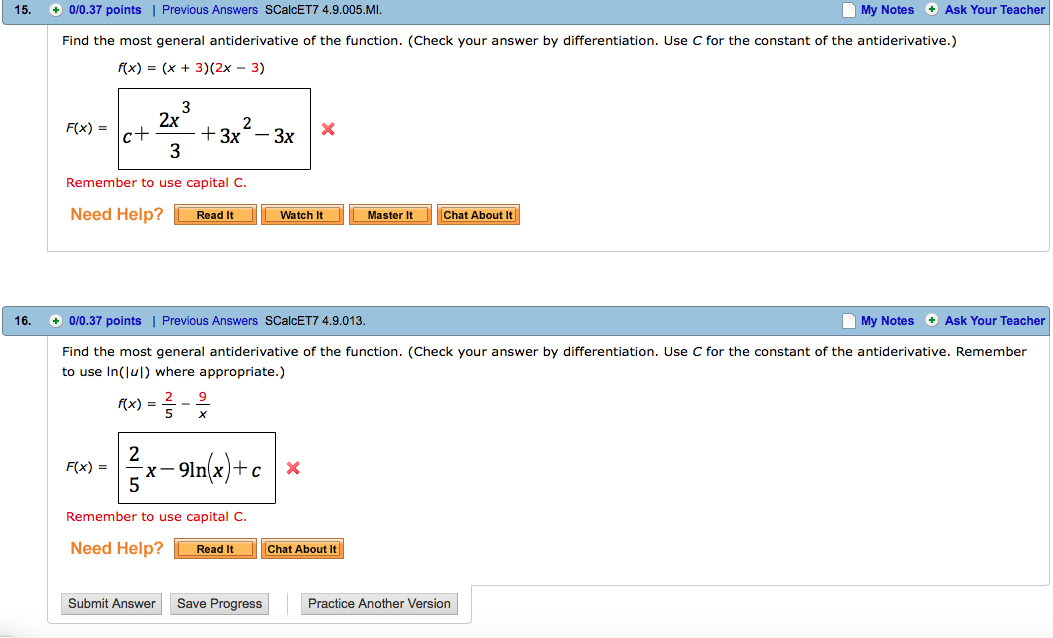
Solved Find the most general antiderivative of the function.
Let $\size x$ be the absolute value of $x$ for real $x$. D dx |u| = u |u| ⋅ du dx. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Y' = 2(x −2) 2√(x − 2)2 → power rule. What is the derivative of an absolute value?
Download Example Of Absolute Function ClipartKey
Y' = 2(x −2) 2√(x − 2)2 → power rule. D dx |u| = u |u| ⋅ du dx. The absolute value function | x | is defined. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. What is the derivative of an absolute value?
Finding the Derivative of Absolute Value Overview & Examples Video
The absolute value function | x | is defined. To understand the derivative of the absolute value function | x |, let’s break it down step by step. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. The derivative of absolute value (function) is defined as the rate of change or the slope of.
Differentiate Absolute Value Function
To understand the derivative of the absolute value function | x |, let’s break it down step by step. Let $\size x$ be the absolute value of $x$ for real $x$. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Since an absolute value function is represented by the graph of two “linear” equations.
Differentiate Absolute Value Function
The absolute value function | x | is defined. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Y' = 2(x −2) 2√(x − 2)2 → power rule. Let $\size x$ be the absolute value of $x$ for real $x$. D dx |u| = u |u|.
1 Absolute Function and Graph It PDF
Y' = 2(x −2) 2√(x − 2)2 → power rule. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. D dx |u| = u |u| ⋅ du dx. The absolute value function | x | is defined. Let $\size x$ be the absolute value of.
Differentiate Absolute Value Function
To understand the derivative of the absolute value function | x |, let’s break it down step by step. Let $\size x$ be the absolute value of $x$ for real $x$. The absolute value function | x | is defined. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Y' = 2(x −2) 2√(x.
Solved Find the most general antiderivative of the function
Y' = 2(x −2) 2√(x − 2)2 → power rule. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. The absolute value function | x | is defined. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. To understand the.
Absolute Value Function Khan Academy at Lemuel McLaughlin blog
Let $\size x$ be the absolute value of $x$ for real $x$. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. D dx |u| = u |u| ⋅ du dx. Y' = 2(x −2) 2√(x − 2)2 → power rule. To understand the derivative of the.
Solved Find the most general antiderivative of the function.
Let $\size x$ be the absolute value of $x$ for real $x$. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. D dx |u| = u |u| ⋅ du dx. Y' = 2(x −2) 2√(x − 2)2 → power rule. The derivative of absolute value (function) is defined as the rate of change or.
The Absolute Value Function | X | Is Defined.
Let $\size x$ be the absolute value of $x$ for real $x$. What is the derivative of an absolute value? Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$.
D Dx |U| = U |U| ⋅ Du Dx.
To understand the derivative of the absolute value function | x |, let’s break it down step by step. Y' = 2(x −2) 2√(x − 2)2 → power rule. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point.