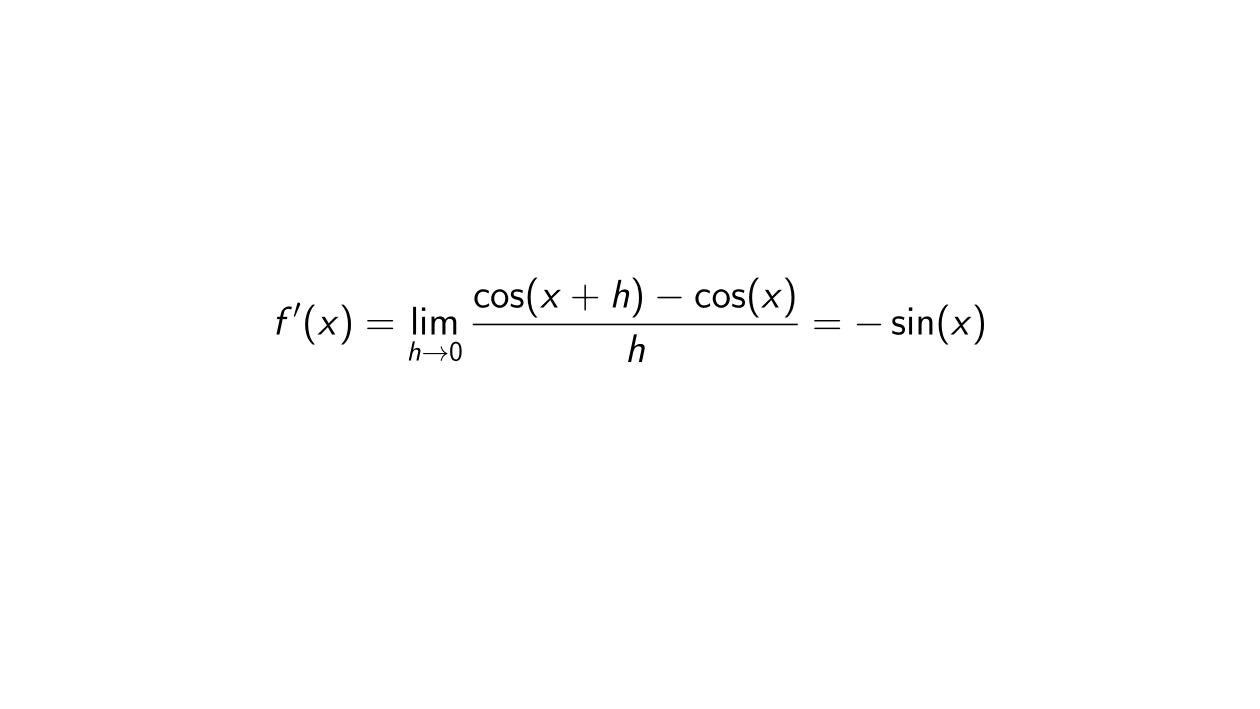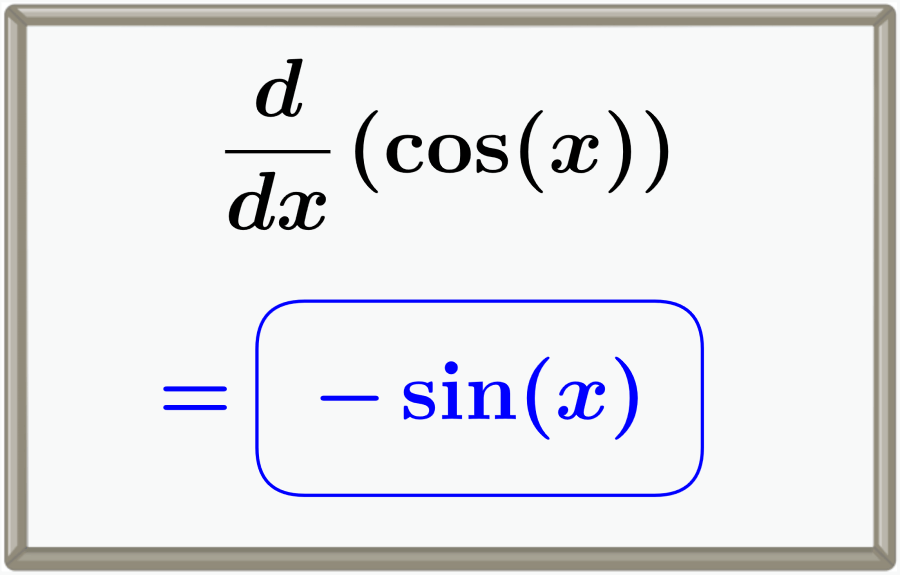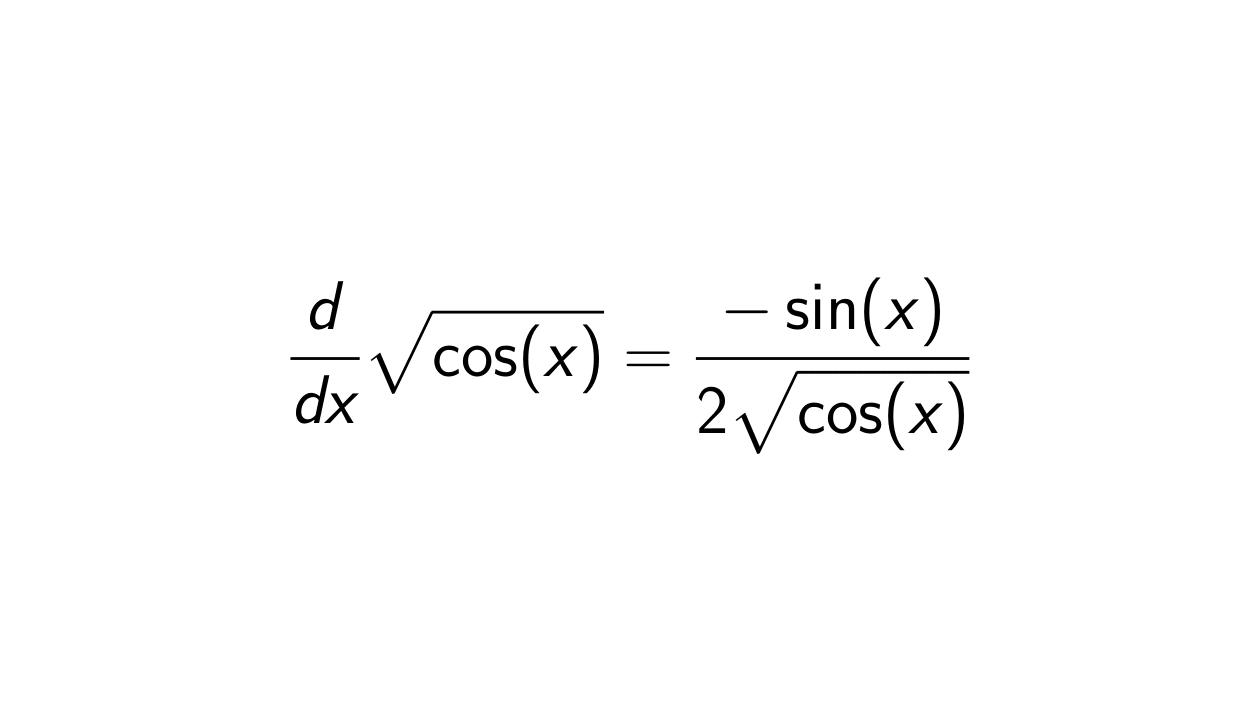Differentiation Of Cosx - We use a technique called logarithmic differentiation to differentiate this kind of function. The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions :
Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : The 2 which will be useful here are: In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. We use a technique called logarithmic differentiation to differentiate this kind of function. Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then
In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : We use a technique called logarithmic differentiation to differentiate this kind of function.
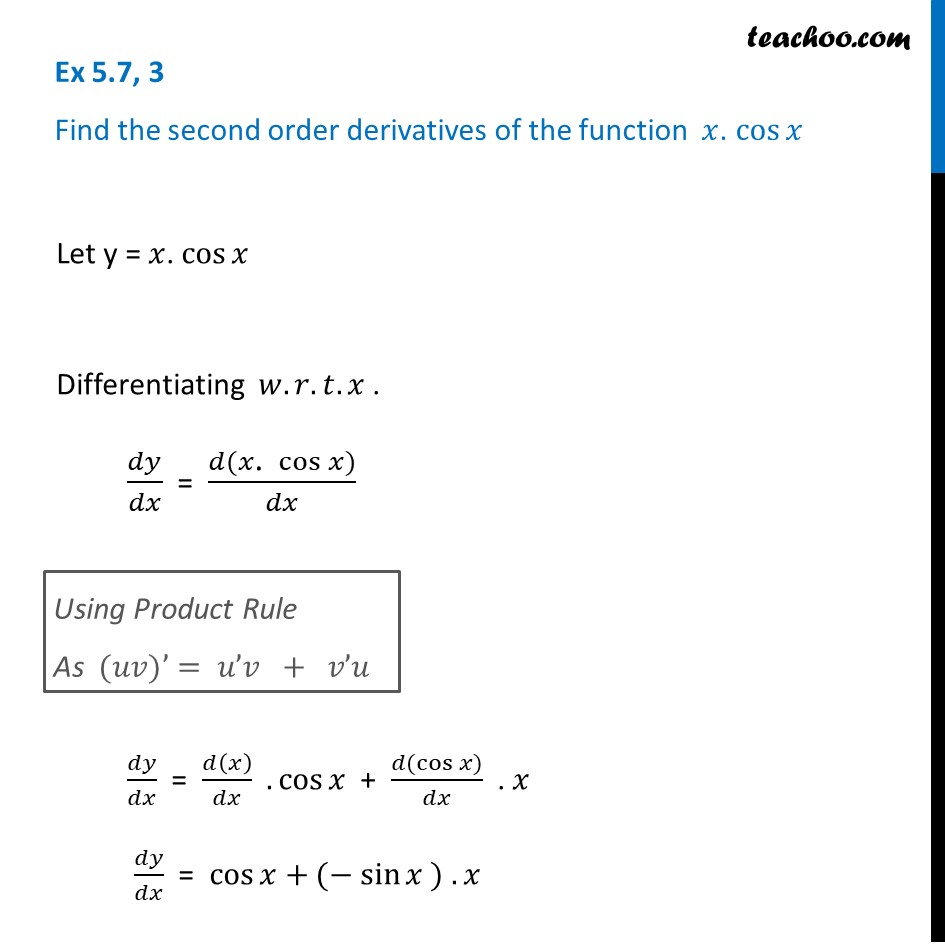
Ex 5.7, 3 Find second order derivatives of x cosx Ex 5.7
Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. We use a technique called logarithmic differentiation to differentiate this kind of function. The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx).
to how cos(x) solve 1 5576666 cosx/1 sinx cosx=tan(x/2)
We use a technique called logarithmic differentiation to differentiate this kind of function. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the.
Differentiation of Trigonometric Functions Kunduz
The 2 which will be useful here are: In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : We use a technique called logarithmic differentiation to differentiate this kind of function. Reminder ∙ d dx (sinx) = cosx and d dx (cosx).
What is the differentiation of cosx with respect to x equals to?
Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. We use a technique called logarithmic differentiation to differentiate this kind of function. Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the.
Derivative of Cosine, cos(x) Formula, Proof, and Graphs Neurochispas
Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then The 2 which will be useful here are: Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos.
Derivative of square root cos(x) Detailed Solution Epsilonify
Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then The 2 which will be.
y = cot^1(cosxsinx/cosx+sinx) Find the derivative Maths Inverse
We use a technique called logarithmic differentiation to differentiate this kind of function. In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx).
Differentiating f(x)=cosx Using a Specific Rule Calculus
We use a technique called logarithmic differentiation to differentiate this kind of function. Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : The 2 which will be useful here are: In short,.
Ex 5.7, 3 Find second order derivatives of x cosx Ex 5.7
Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. We use a technique called logarithmic differentiation to differentiate this kind of function. The 2.
Derivative of Cos(x) Formula, Proof in Easy Steps, Formula, Solved
We use a technique called logarithmic differentiation to differentiate this kind of function. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then In short,.
We Use A Technique Called Logarithmic Differentiation To Differentiate This Kind Of Function.
In short, we let y = (cos (x))^x, then, ln (y) = ln ( (cos (x))^x) ln (y. Dy/dx=sinx*sin (cosx) first from the differentiation of trigonometric functions : The 2 which will be useful here are: Reminder ∙ d dx (sinx) = cosx and d dx (cosx) = − sinx to differentiate xsinx use the product rule given f (x) = g(x)h(x) then