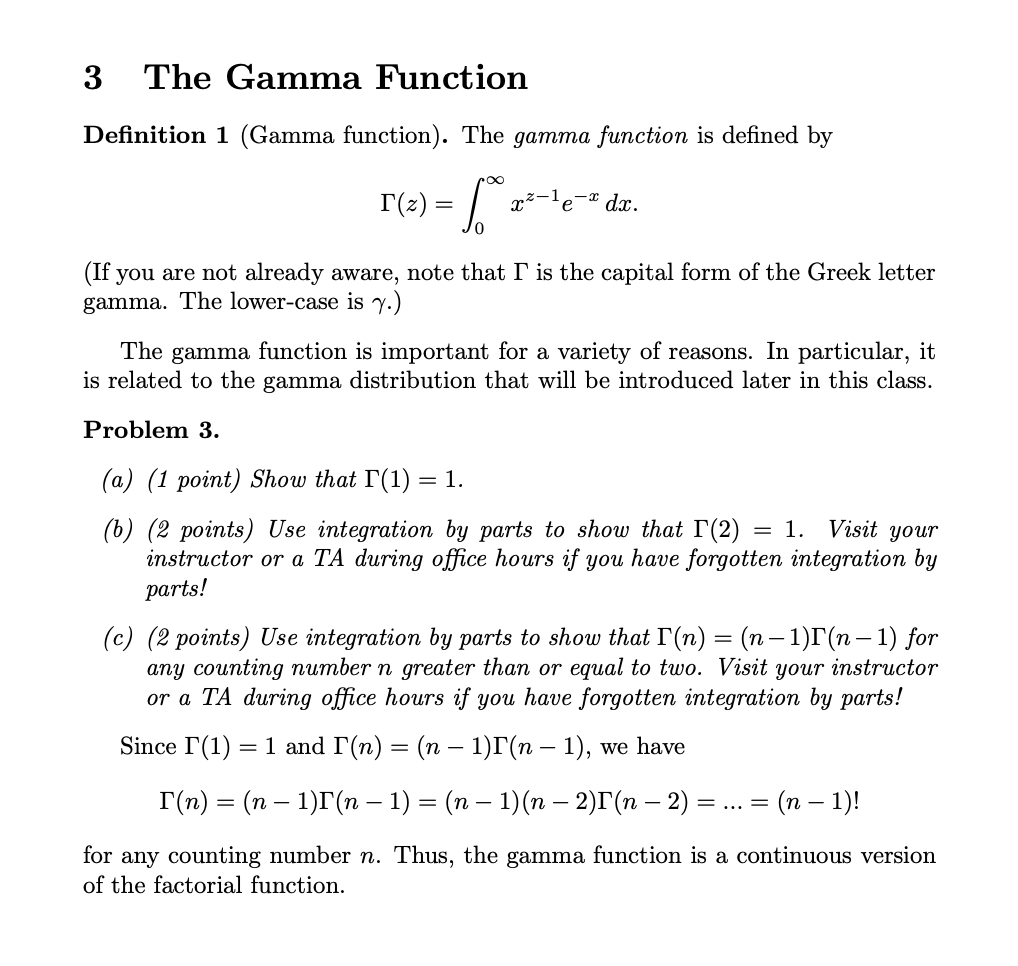
Differentiation Of Gamma Function - In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. The formal definition is given. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. $\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e.
In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. $\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. The formal definition is given.
In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. The formal definition is given. $\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at.
SOLUTION Gamma function notes Studypool
It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. The formal definition is given. In this.
SOLUTION Gamma function notes Studypool
$\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. The derivatives of the gamma functions , , , and , and their inverses and with respect to.
Gamma Function and Gamma Probability Density Function Academy
$\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for.
Gamma function Wikiwand
In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The formal definition is given. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e −.
Gamma Function — Intuition, Derivation, and Examples Negative numbers
In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. $\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. The formal.
SOLUTION Gamma function notes Studypool
The formal definition is given. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with respect to x yields γ ′ (x) = ∫∞ 0e. In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. It is a function whose derivative.
Gamma Function Formula Example with Explanation
$\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. It is.
SOLUTION Gamma function notes Studypool
The formal definition is given. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. Consider the integral form of.
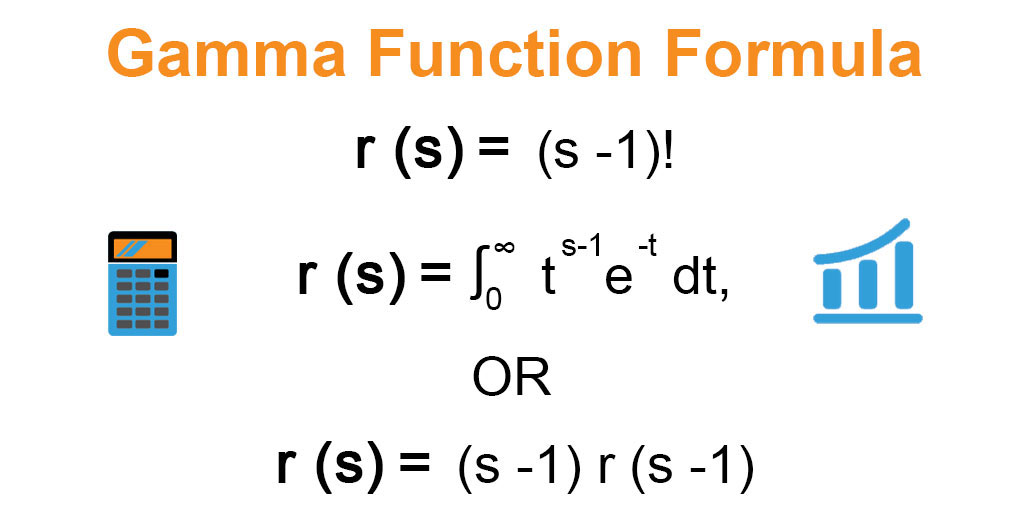
Calculations With the Gamma Function
The formal definition is given. $\map {\gamma'} 1$ denotes the derivative of the gamma function evaluated at. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. Consider the integral form of the gamma function, γ(x) = ∫∞ 0e − ttx − 1dt taking the derivative with.
Solved 3 The Gamma Function Definition 1 (Gamma function).
It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary. In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The derivatives of the gamma functions , , , and , and their inverses and with respect to.
$\Map {\Gamma'} 1$ Denotes The Derivative Of The Gamma Function Evaluated At.
In this note, i will sketch some of the main properties of the logarithmic derivative∗ of the gamma function. The derivatives of the gamma functions , , , and , and their inverses and with respect to the parameter can be represented in terms of the. The formal definition is given. It is a function whose derivative is not contained in c(x, γ) or any elementary extension thereof, for a suitable definition of elementary.






/Complex_gamma_function_abs-6ca390af978c43c091f0c4af8eff24d5.jpg)