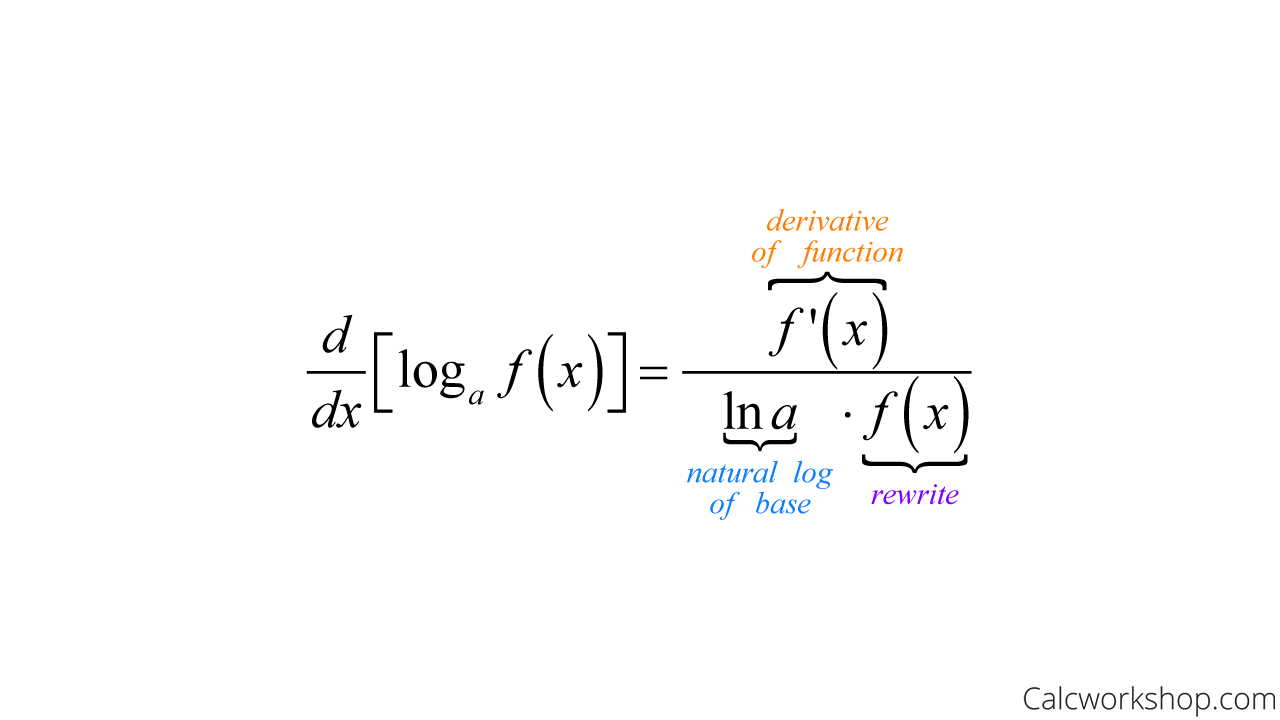
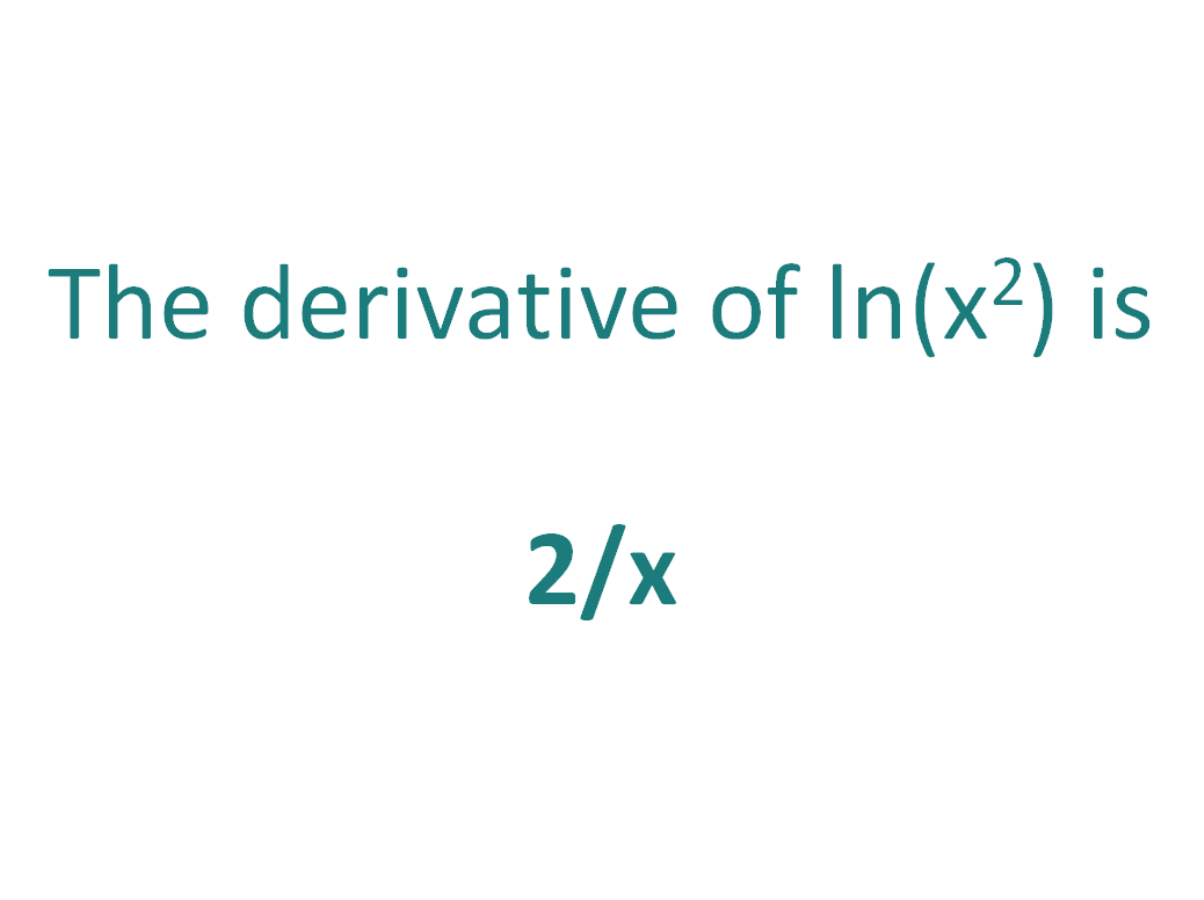Differentiation Of Ln X 1 - We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Define e = lim h →. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Type in any function derivative to get the solution, steps and graph.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Define e = lim h →. Type in any function derivative to get the solution, steps and graph.
Define e = lim h →. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Type in any function derivative to get the solution, steps and graph. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y.
Derivative of ln x (Natural Log) Formula Differentiation of ln x (2024)
Define e = lim h →. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Type in any function derivative to get the solution, steps and graph. We can use a formula to find the derivative.
Differentiation Formulas Ln
As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Define e = lim h.
Differentiate Ln X 2
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Type in any function derivative to get the solution, steps and graph. Define e = lim h →. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point.
Derivative of ln x Explained. The Education
Define e = lim h →. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Type in any function derivative to get the solution, steps and graph. We can use a formula to find the derivative.
What Is The Differentiation Process For [math]\ln(x), 51 OFF
As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Type in any function derivative to get the solution, steps and graph. Define e = lim h →. We can use a formula to find the derivative.
Differentiate Ln X 2 Warrentrust
As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Type in any function derivative.
How do you find the derivative of f(x) = ln(e^x x)? Socratic
Type in any function derivative to get the solution, steps and graph. Define e = lim h →. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point.
Differentiation Formulas Ln
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point of its domain, and (ln) ′ (y) = 1 elny = 1 y. Type in any function derivative.
Derivative of Ln X ElihasPope
Define e = lim h →. Type in any function derivative to get the solution, steps and graph. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point.
Differentiation Formulas Ln
Type in any function derivative to get the solution, steps and graph. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Define e = lim h →. As (ex) ′ = ex ≠ 0 for all x, we know that ln has a derivative at each point.
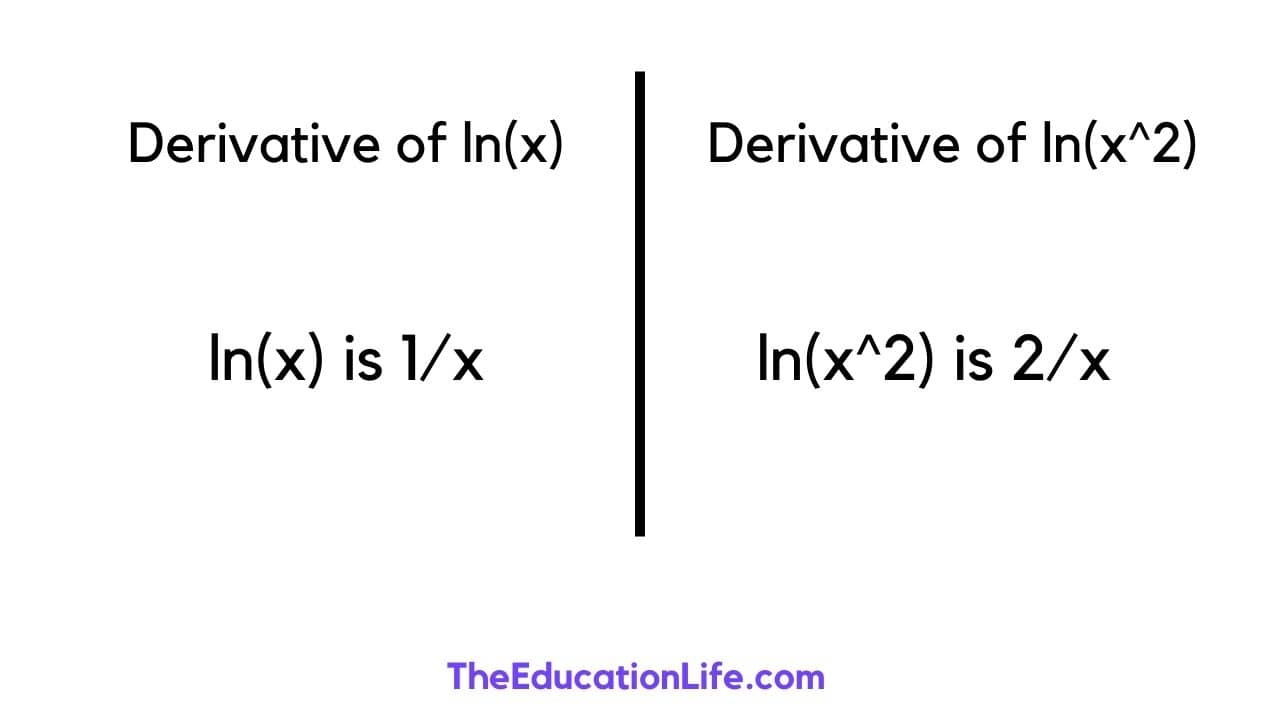
As (Ex) ′ = Ex ≠ 0 For All X, We Know That Ln Has A Derivative At Each Point Of Its Domain, And (Ln) ′ (Y) = 1 Elny = 1 Y.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Type in any function derivative to get the solution, steps and graph. Define e = lim h →.
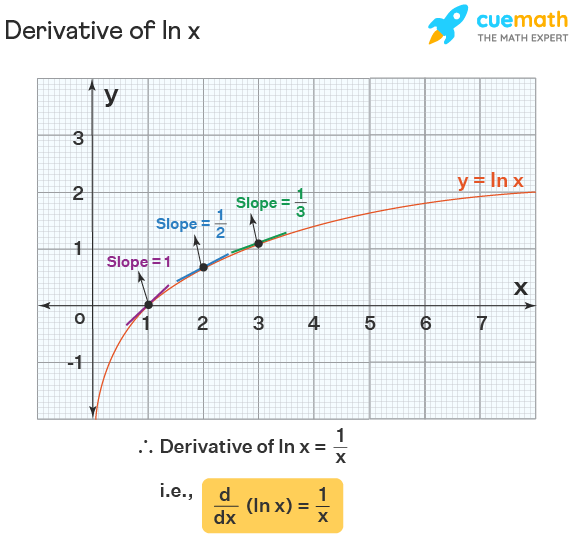


![What Is The Differentiation Process For [math]\ln(x), 51 OFF](https://i.ytimg.com/vi/v1gD42-vlBk/maxresdefault.jpg)