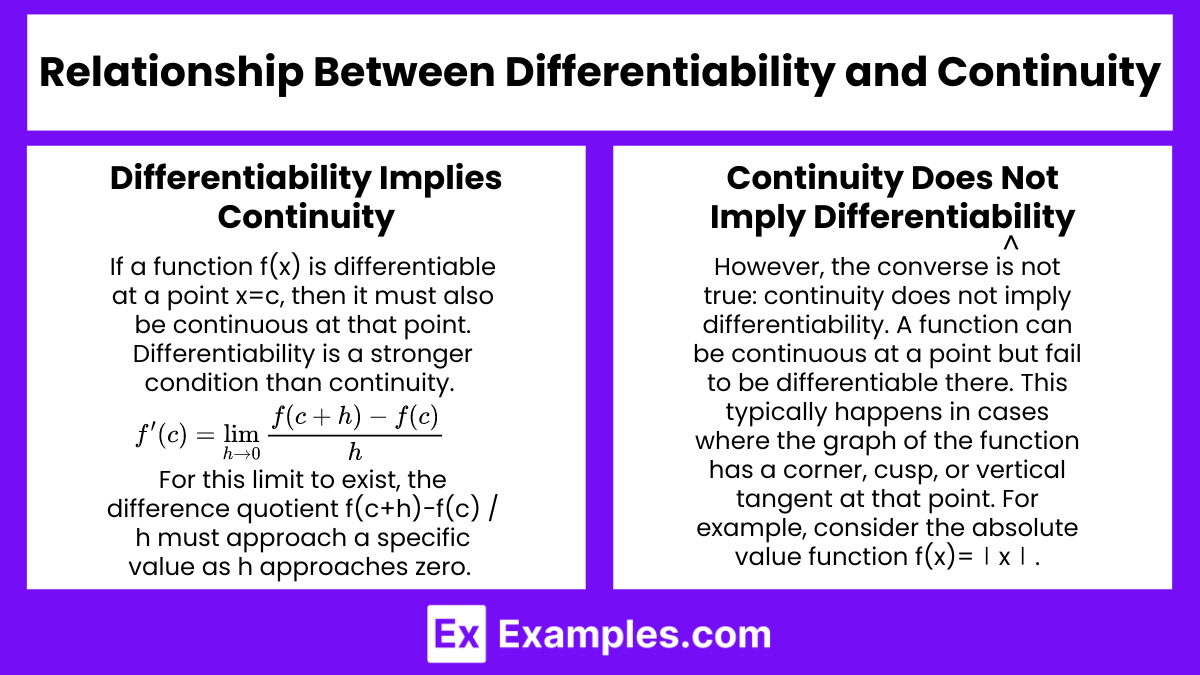
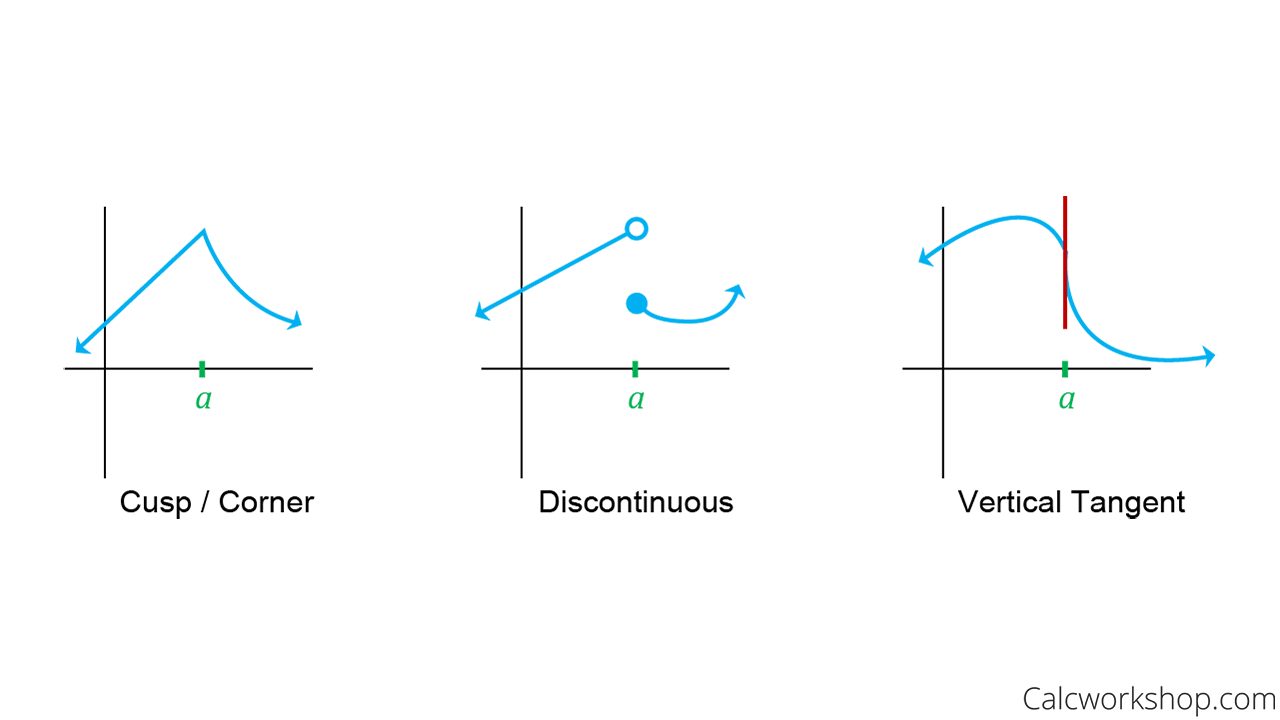
Does Continuity Imply Differentiability - Learn why any differentiable function is automatically continuous, and see the proof and examples. Continuity refers to a definition of the concept of a function that varies without. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. In other words, if a function can be differentiated at a point, it is. The web page also explains the. Differentiability is a stronger condition than continuity. Relation between continuity and differentiability:
In other words, if a function can be differentiated at a point, it is. Differentiability is a stronger condition than continuity. Relation between continuity and differentiability: Continuity refers to a definition of the concept of a function that varies without. Learn why any differentiable function is automatically continuous, and see the proof and examples. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. The web page also explains the. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0.
Continuity refers to a definition of the concept of a function that varies without. Relation between continuity and differentiability: The web page also explains the. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. Differentiability is a stronger condition than continuity. Learn why any differentiable function is automatically continuous, and see the proof and examples. In other words, if a function can be differentiated at a point, it is.
Unit 2.2 Connecting Differentiability and Continuity (Notes
Continuity refers to a definition of the concept of a function that varies without. Differentiability is a stronger condition than continuity. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. The web page also explains the. Learn why any differentiable function is automatically.
Which of the following is true? (a) differentiability does not imply
Differentiability is a stronger condition than continuity. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. Relation between continuity and differentiability: In other words, if a function can be differentiated at a point, it is. Learn why any differentiable function is automatically continuous,.
calculus What does differentiability imply in this proof
Learn why any differentiable function is automatically continuous, and see the proof and examples. Continuity refers to a definition of the concept of a function that varies without. Relation between continuity and differentiability: In other words, if a function can be differentiated at a point, it is. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y).
Continuity and Differentiability (Fully Explained w/ Examples!)
Differentiability is a stronger condition than continuity. The web page also explains the. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. Continuity refers to a definition of the concept of a function that varies without. Differentiability requires that f(x) − f(y) →.
Continuity & Differentiability
Differentiability is a stronger condition than continuity. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. In other words, if a function can be differentiated at a point, it is. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y).
derivatives Differentiability Implies Continuity (Multivariable
Differentiability is a stronger condition than continuity. Continuity refers to a definition of the concept of a function that varies without. Learn why any differentiable function is automatically continuous, and see the proof and examples. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. Continuity requires that f(x) − f(y) → 0.
Which of the following is true? (a) differentiability does not imply
Continuity refers to a definition of the concept of a function that varies without. Differentiability is a stronger condition than continuity. The web page also explains the. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. In other words, if a function can.
Which of the following is true? (a) differentiability does not imply
Relation between continuity and differentiability: Continuity refers to a definition of the concept of a function that varies without. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. Learn why any differentiable function is automatically continuous, and see the proof and examples. Continuity requires that f(x) − f(y) → 0 f (x).
derivatives Differentiability Implies Continuity (Multivariable
Relation between continuity and differentiability: Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. In other words, if a function can be differentiated at a point, it is. Differentiability is a stronger condition than continuity. Continuity refers to a definition of the concept of a function that varies without.
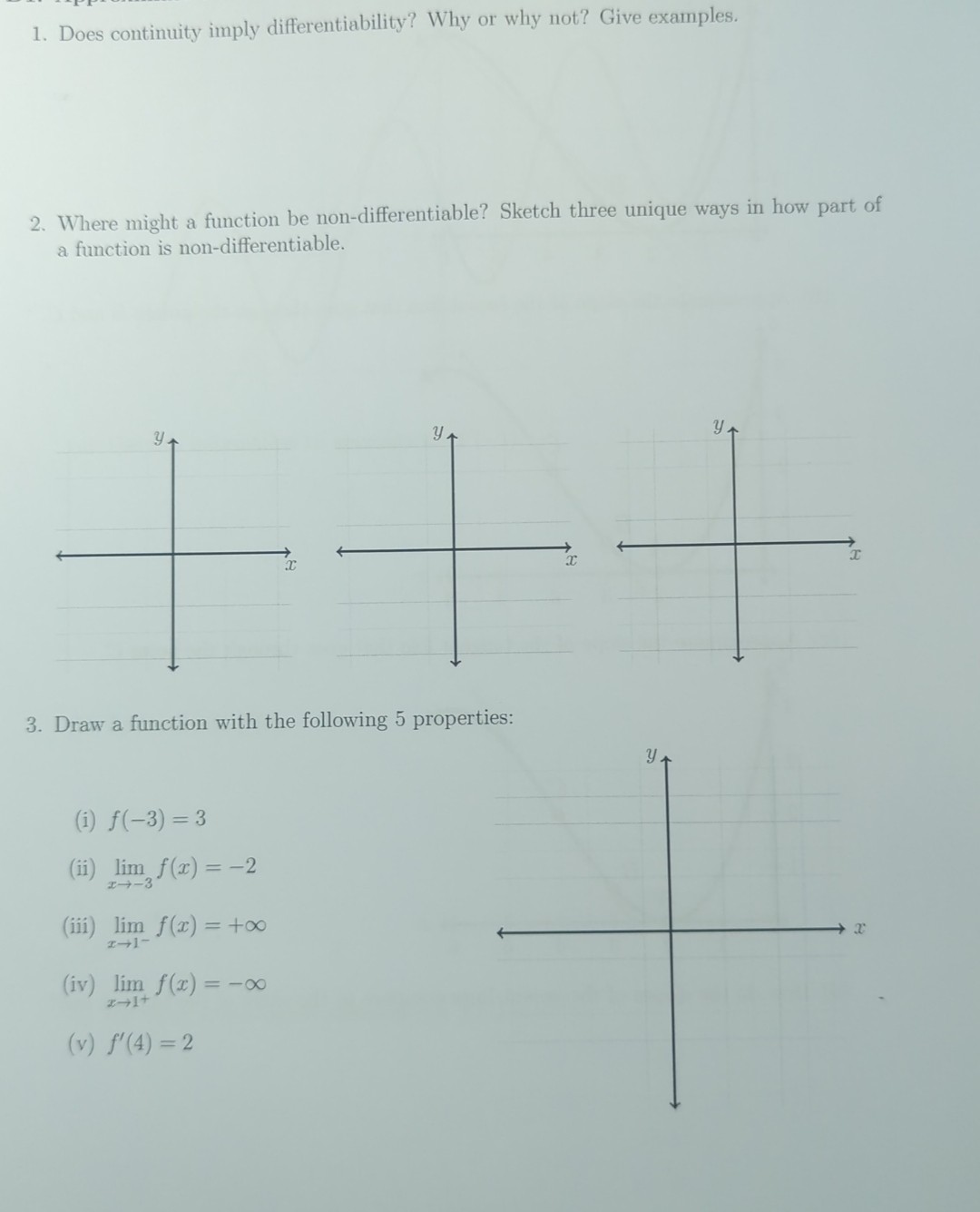
Solved Does continuity imply differentiability? Why or why
Learn why any differentiable function is automatically continuous, and see the proof and examples. The web page also explains the. In other words, if a function can be differentiated at a point, it is. Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0..
In Other Words, If A Function Can Be Differentiated At A Point, It Is.
Relation between continuity and differentiability: Continuity requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x − y → 0 x − y → 0. Differentiability requires that f(x) − f(y) → 0 f (x) − f (y) → 0 as x. Differentiability is a stronger condition than continuity.
The Web Page Also Explains The.
Learn why any differentiable function is automatically continuous, and see the proof and examples. Continuity refers to a definition of the concept of a function that varies without.