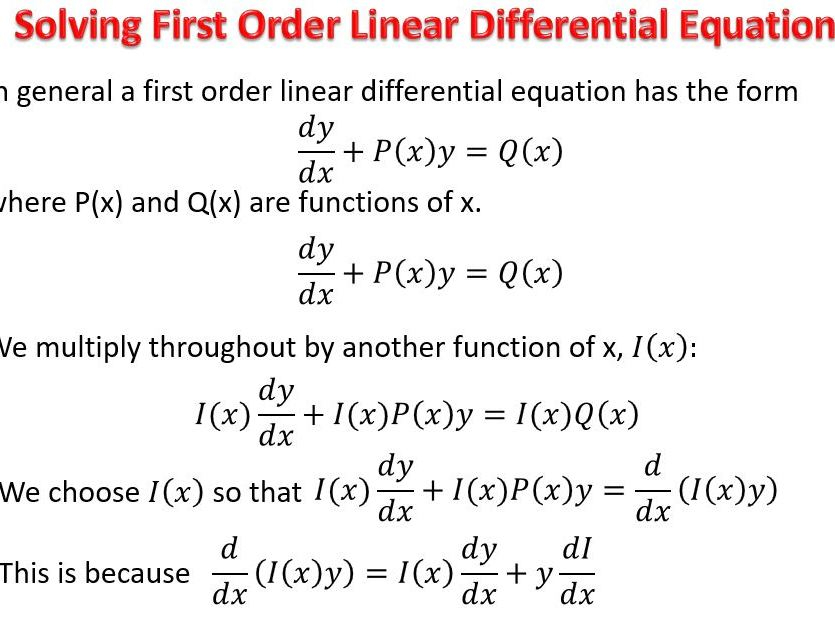
First Order Nonhomogeneous Differential Equation

First Order Nonhomogeneous Differential Equation - Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. In this section we will discuss the basics of solving nonhomogeneous differential equations. We define the complimentary and. Let us first focus on the nonhomogeneous first order equation.

Let us first focus on the nonhomogeneous first order equation. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. In this section we will discuss the basics of solving nonhomogeneous differential equations. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. We define the complimentary and.

Let us first focus on the nonhomogeneous first order equation. In this section we will discuss the basics of solving nonhomogeneous differential equations. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. We define the complimentary and.

Solved Consider the first order nonhomogeneous differential

Let us first focus on the nonhomogeneous first order equation. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. We define the complimentary and. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. A differential equation of type \[y' + a\left( x \right)y = f\left( x.

[Free Solution] In Chapter 6, you solved the firstorder linear

A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. We define the complimentary and. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. In this section we will discuss the basics.

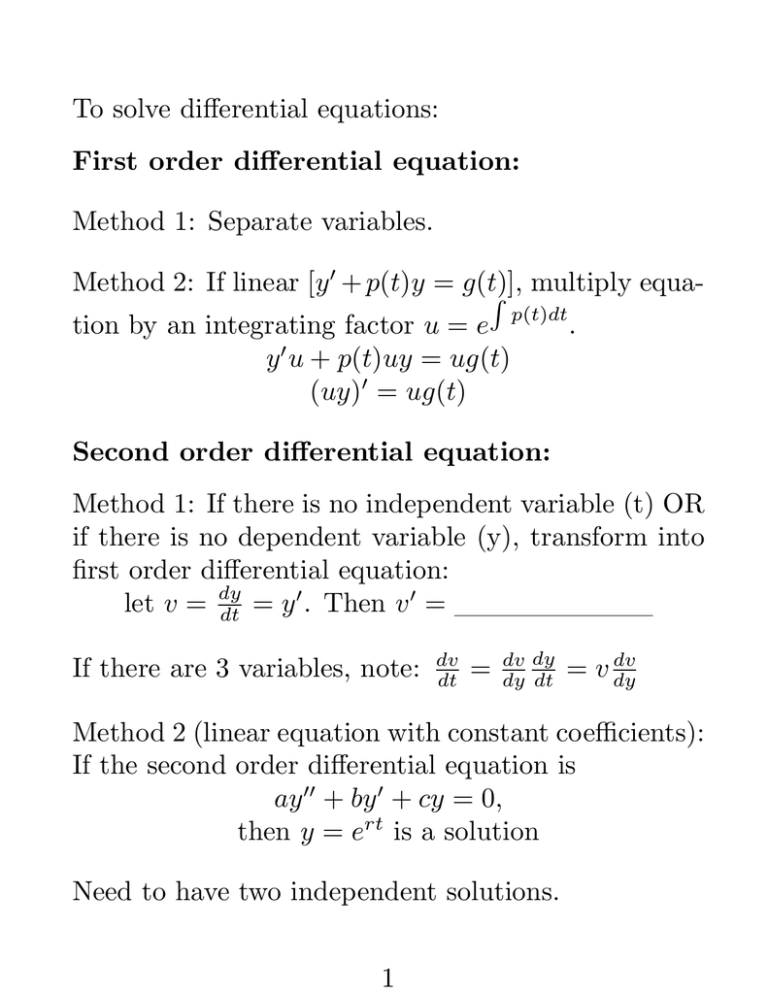
To solve differential equations First order differential equation

Let us first focus on the nonhomogeneous first order equation. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t).

solve the initial value problem first order differential equation

Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. We define the complimentary and. Let us first focus on the nonhomogeneous first order equation. In this section we will discuss the basics of solving nonhomogeneous differential equations. A differential equation of type \[y' + a\left( x \right)y = f\left( x.

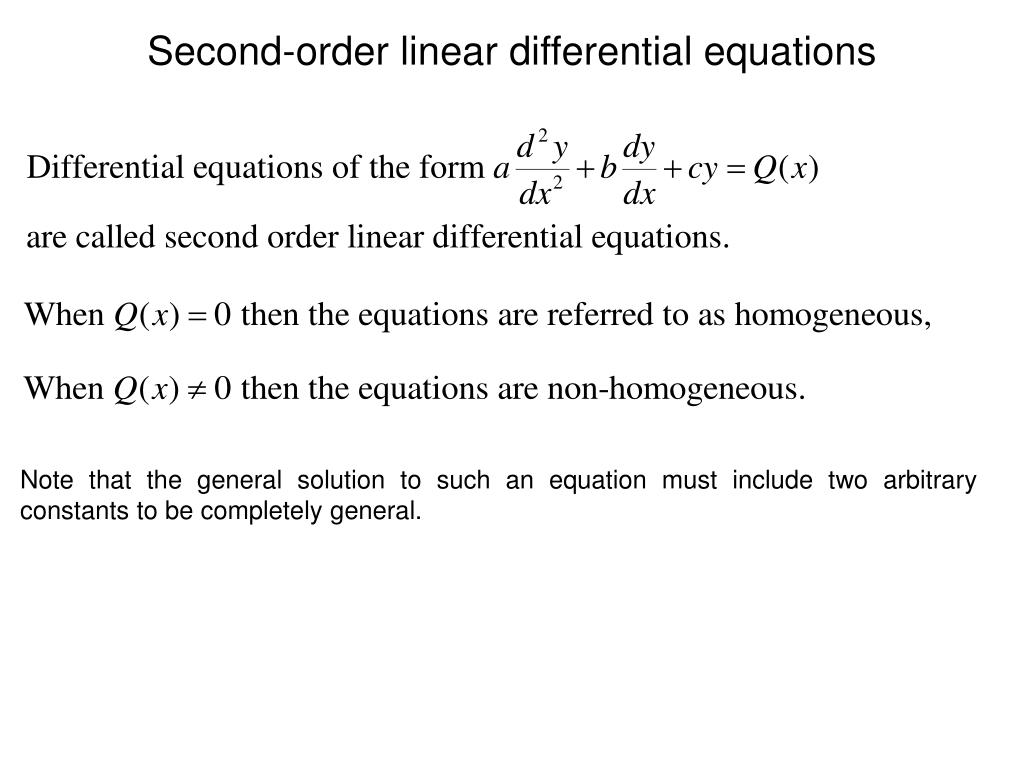
Second Order Differential Equation Solved Find The Second Order

Let us first focus on the nonhomogeneous first order equation. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. In this section.

Differential Equation Calculator

Let us first focus on the nonhomogeneous first order equation. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is.

SOLVED The equation is linear homogeneous differential equation of

→x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. Let us first focus on the nonhomogeneous first order equation. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear. Let us first.

[Solved] Problem 1. A firstorder nonhomogeneous linear d

Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. We define the complimentary and. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. Let us first focus on the nonhomogeneous first order equation. In this section we will discuss the basics of solving nonhomogeneous differential equations.

(PDF) Solution of First Order Linear Non Homogeneous Ordinary

In this section we will discuss the basics of solving nonhomogeneous differential equations. We define the complimentary and. →x ′ (t) = a→x(t) + →f(t), where a is a constant matrix. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x.

First Order Differential Equation Worksheet Equations Worksheets

In This Section We Will Discuss The Basics Of Solving Nonhomogeneous Differential Equations.

Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) = a\vec{x}(t) + \vec{f}(t) , \end{equation*} where. We define the complimentary and. Let us first focus on the nonhomogeneous first order equation. A differential equation of type \[y' + a\left( x \right)y = f\left( x \right),\] where a ( x ) and f ( x ) are continuous functions of x , is called a linear.