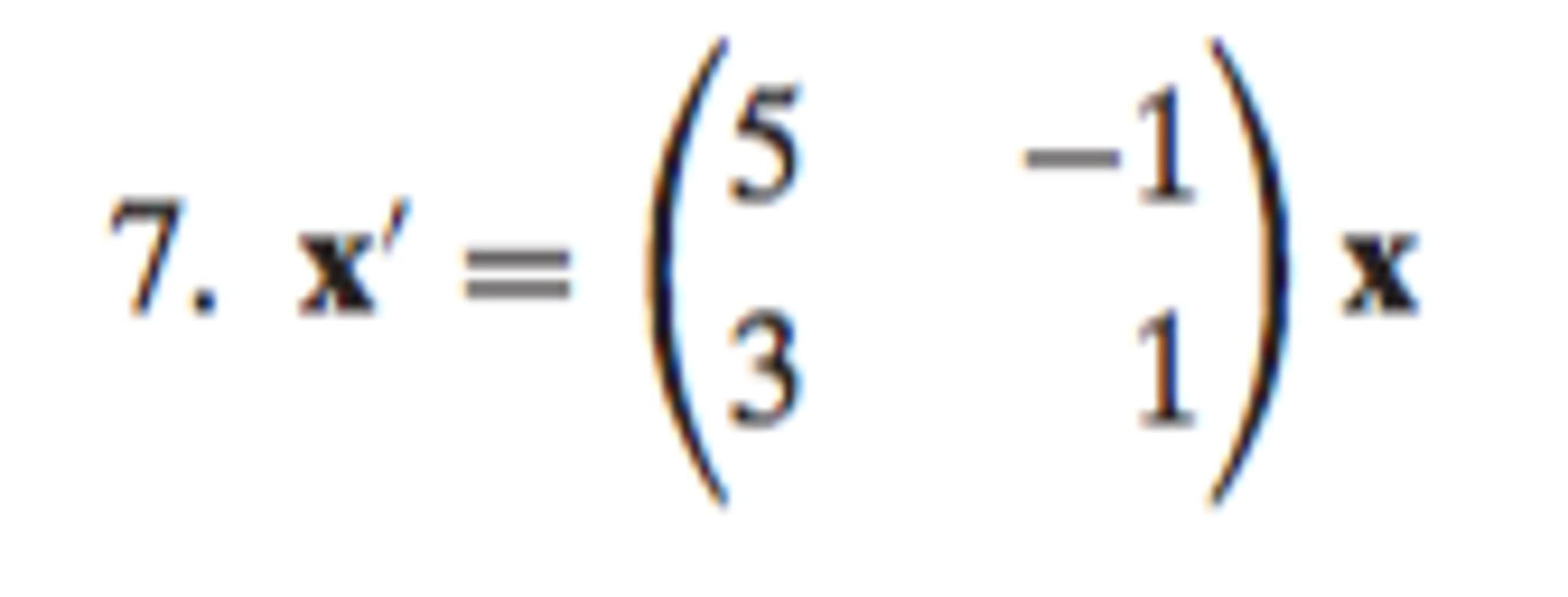Fundamental Matrix Differential Equations

Fundamental Matrix Differential Equations - It is therefore useful to have a. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. As t varies, the point x(t) traces out a curve in rn. A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. This section is devoted to fundamental matrices for linear differential equations. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. There are many ways to pick two independent solu tions of x = a x to form the columns of φ.

This section is devoted to fundamental matrices for linear differential equations. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. As t varies, the point x(t) traces out a curve in rn. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). It is therefore useful to have a. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown.

There are many ways to pick two independent solu tions of x = a x to form the columns of φ. It is therefore useful to have a. As t varies, the point x(t) traces out a curve in rn. A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). This section is devoted to fundamental matrices for linear differential equations.

Fundamental PrinciplesDifferential Equations and Their Solutions

A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. As t varies, the point x(t) traces out a.

(PDF) On graph differential equations and its associated matrix

The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the.

arrays Implement solution of differential equations system using

A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. There are many ways to pick two independent solu tions of x =.

Matrix differential equation Alchetron, the free social encyclopedia

As t varies, the point x(t) traces out a curve in rn. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. It is.

(PDF) FourthOrder Approximation of the Fundamental Matrix of Linear

The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. There are many ways to pick two independent solu tions of x = a x to form the columns of φ..

Modelling Motion with Differential Equations

It is therefore useful to have a. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. As t varies, the point x(t) traces out a curve in rn. Fundamental matrix.

Systems of Matrix Differential Equations for Surfaces

A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. It is therefore useful to have a. As t varies, the point x(t) traces out a curve in rn. The matrix valued function \( x (t) \) is called the fundamental.

Textbooks Differential Equations Freeup

There are many ways to pick two independent solu tions of x = a x to form the columns of φ. It is therefore useful to have a. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. As t varies, the point x(t) traces out a curve.

Complex Solution and Fundamental MatrixDifferential Equations and

It is therefore useful to have a. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the.

Solved Find a fundamental matrix for the given system of

It is therefore useful to have a. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. As t varies, the point x(t) traces out a curve in rn. This section is devoted to fundamental matrices for linear differential equations. The fundamental matrix φ(t,t0) is a mapping of the initial condition a.

As T Varies, The Point X(T) Traces Out A Curve In Rn.

There are many ways to pick two independent solu tions of x = a x to form the columns of φ. A fundamental matrix for (1) is any matrix ψ(t) that satisﬁes ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a ﬁrst order diﬀerential equation for an unknown. This section is devoted to fundamental matrices for linear differential equations. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution.