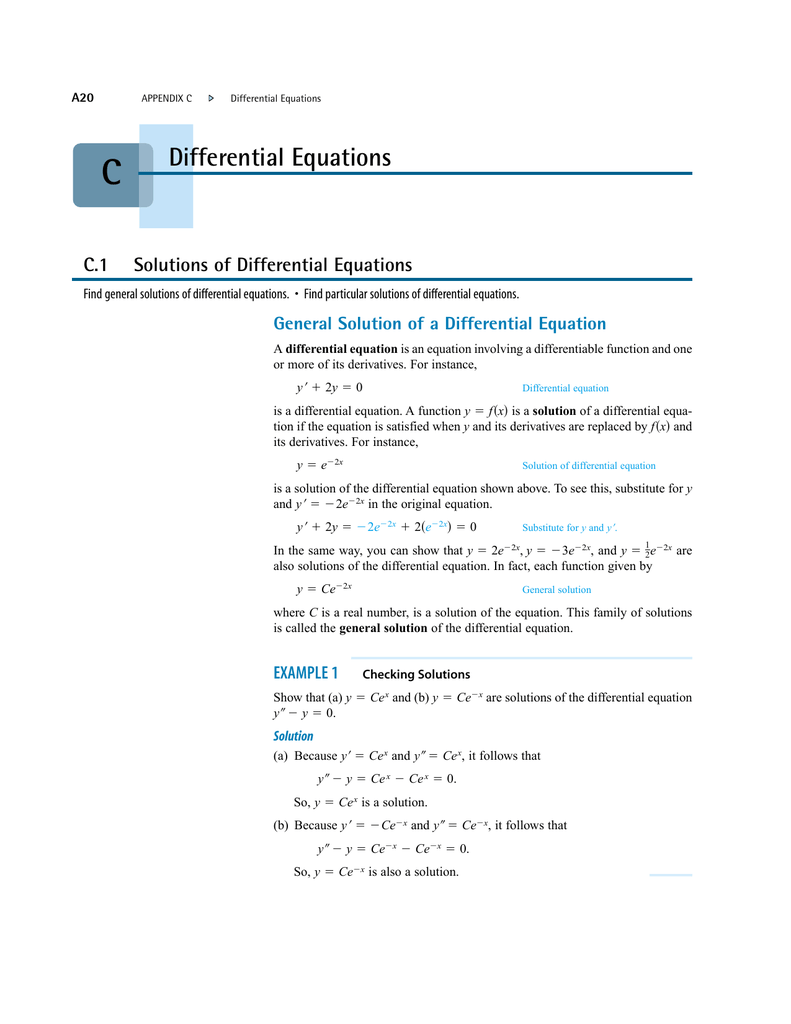
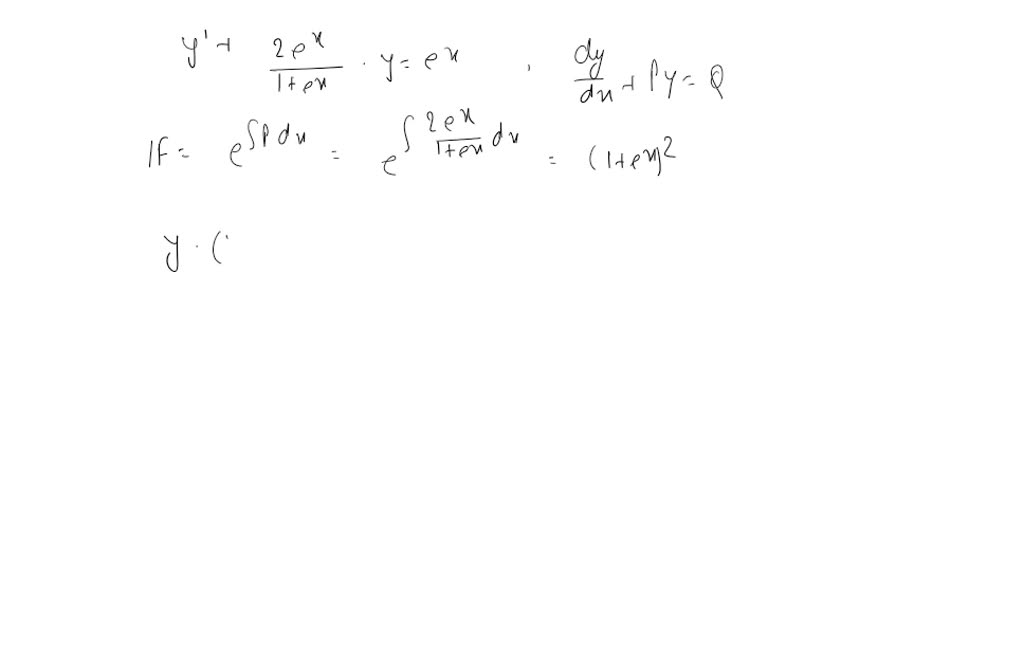General Solutions Of Differential Equations - General solution of differential equations. When n = 1 the equation can be solved using separation of. The general solution of the differential equation encompasses all possible solutions. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. When n = 0 the equation can be solved as a first order linear differential equation.
When n = 1 the equation can be solved using separation of. The general solution of the differential equation encompasses all possible solutions. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. When n = 0 the equation can be solved as a first order linear differential equation. General solution of differential equations.
When n = 0 the equation can be solved as a first order linear differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. The general solution of the differential equation encompasses all possible solutions. General solution of differential equations. When n = 1 the equation can be solved using separation of. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of.
General and Particular Differential Equations Solutions Videos, Examples
When n = 1 the equation can be solved using separation of. When n = 0 the equation can be solved as a first order linear differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. In this article, we will learn about the general solution.
Boundary Conditions for NonHomogeneous Differential Equations
When n = 1 the equation can be solved using separation of. General solution of differential equations. When n = 0 the equation can be solved as a first order linear differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. The general solution of the.
General Solutions to Second Order Differential Equations Mr
In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. When n = 1 the equation can be solved using separation of. When n = 0 the equation can be solved as a first order linear differential equation. We define fundamental sets of solutions and discuss how.
Boundary Conditions for NonHomogeneous Differential Equations
We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. When n = 1 the equation can be solved using separation of. The general solution of the differential equation encompasses all possible solutions. In this article, we will learn about the general solution and the method of finding.
⏩SOLVEDFind the general solutions to the given differential… Numerade
General solution of differential equations. The general solution of the differential equation encompasses all possible solutions. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. When n = 1 the equation can be solved using separation of. When n = 0 the equation can be solved.
Ordinary Differential Equations
When n = 0 the equation can be solved as a first order linear differential equation. General solution of differential equations. When n = 1 the equation can be solved using separation of. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. The general solution of.
[Solved] Find the general solution of the following differential
When n = 0 the equation can be solved as a first order linear differential equation. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous.
Particular Solution of NonHomogeneous Differential Equations Mr
General solution of differential equations. When n = 0 the equation can be solved as a first order linear differential equation. In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. The general solution of the differential equation encompasses all possible solutions. We define fundamental sets of.
Solutions of Differential Equations
We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. When n = 1 the equation can be solved using separation of. General solution of differential equations. In this article, we will learn about the general solution and the method of finding the particular solution from the general.
Differential Equations
When n = 0 the equation can be solved as a first order linear differential equation. General solution of differential equations. The general solution of the differential equation encompasses all possible solutions. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. When n = 1 the equation.
When N = 0 The Equation Can Be Solved As A First Order Linear Differential Equation.
In this article, we will learn about the general solution and the method of finding the particular solution from the general solution of. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. General solution of differential equations. The general solution of the differential equation encompasses all possible solutions.