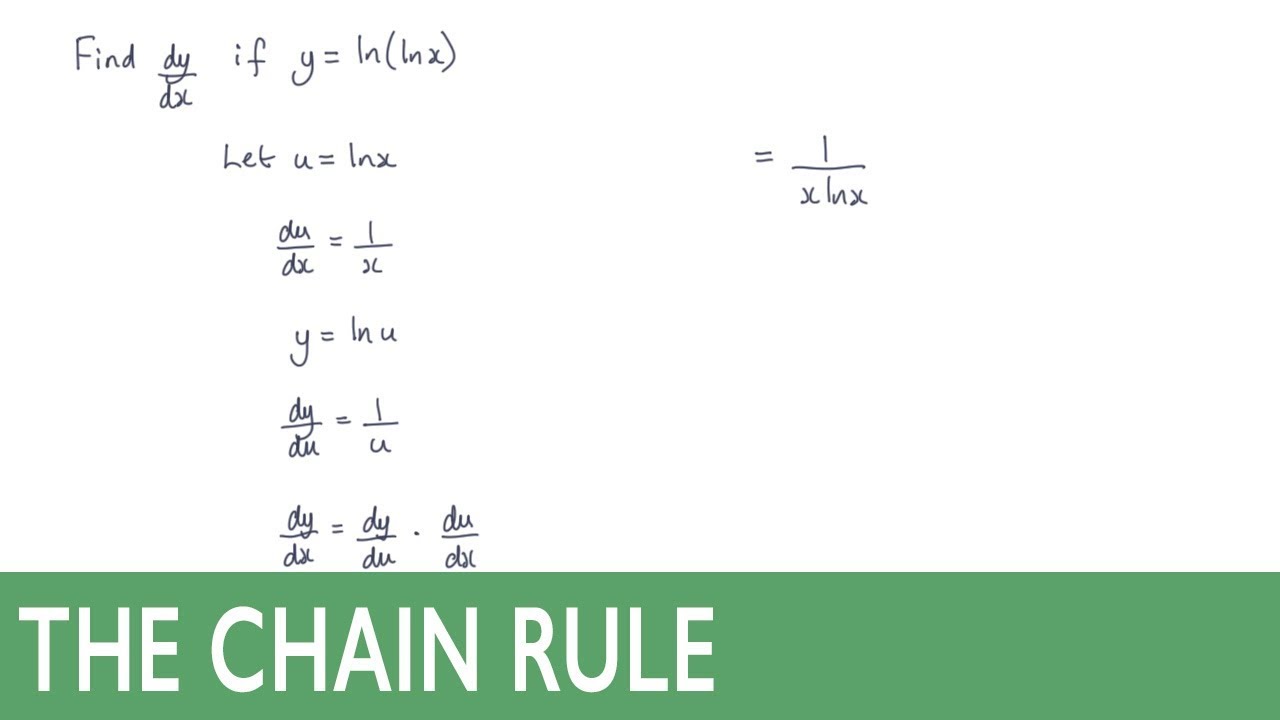
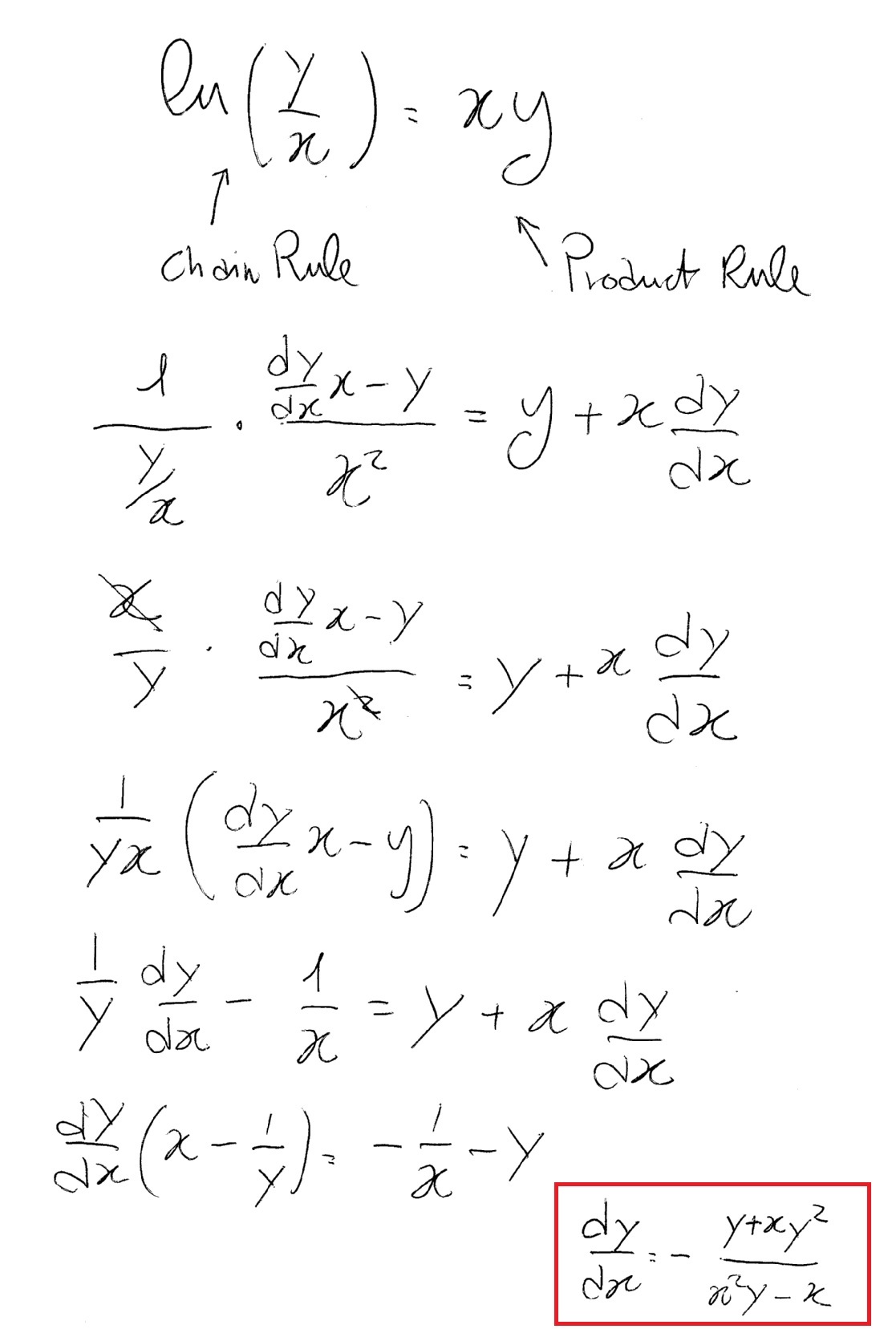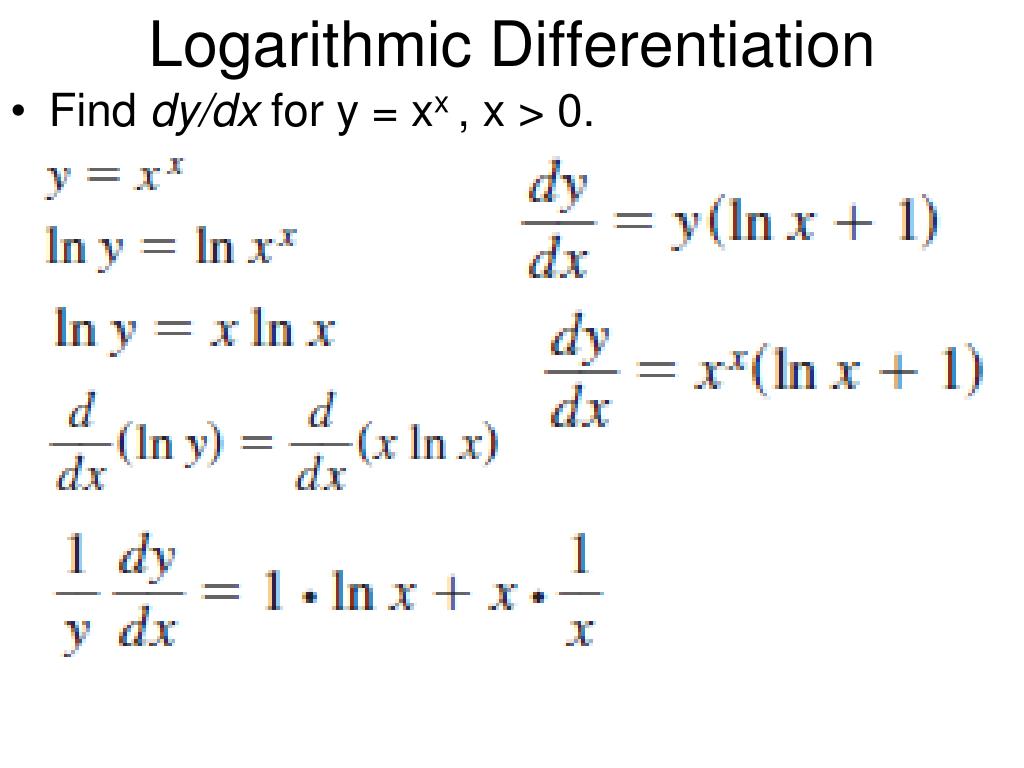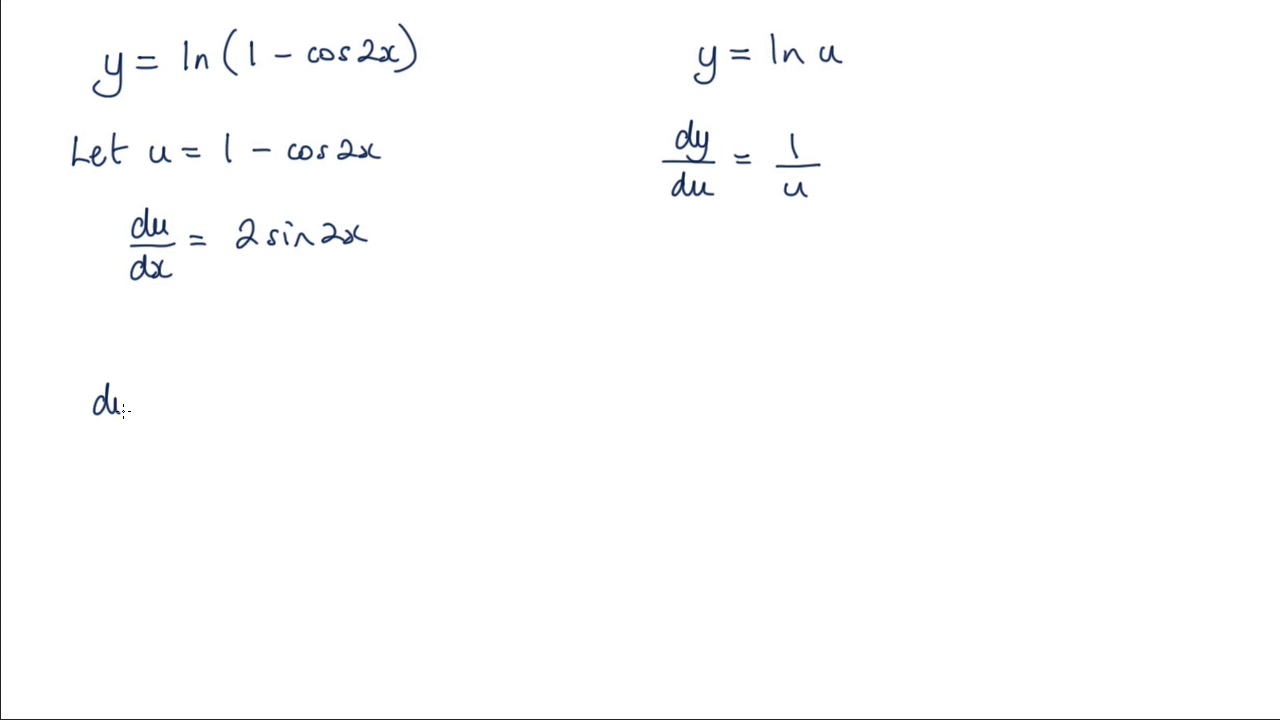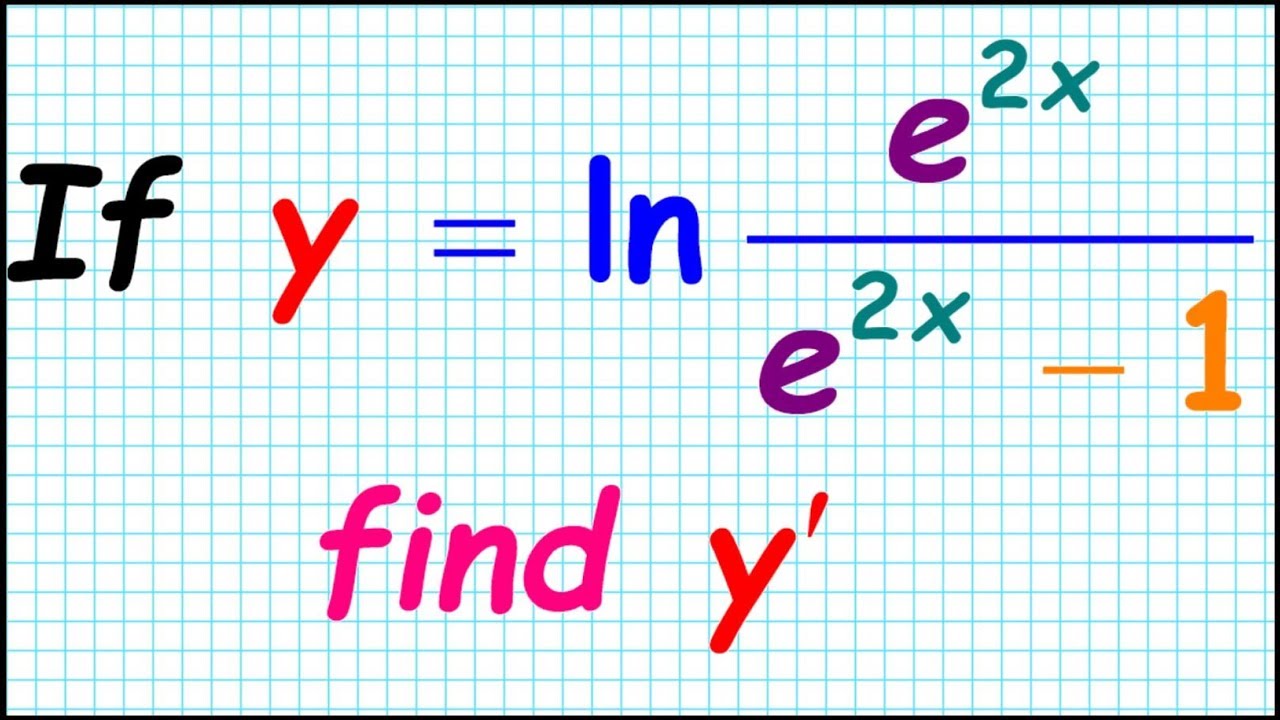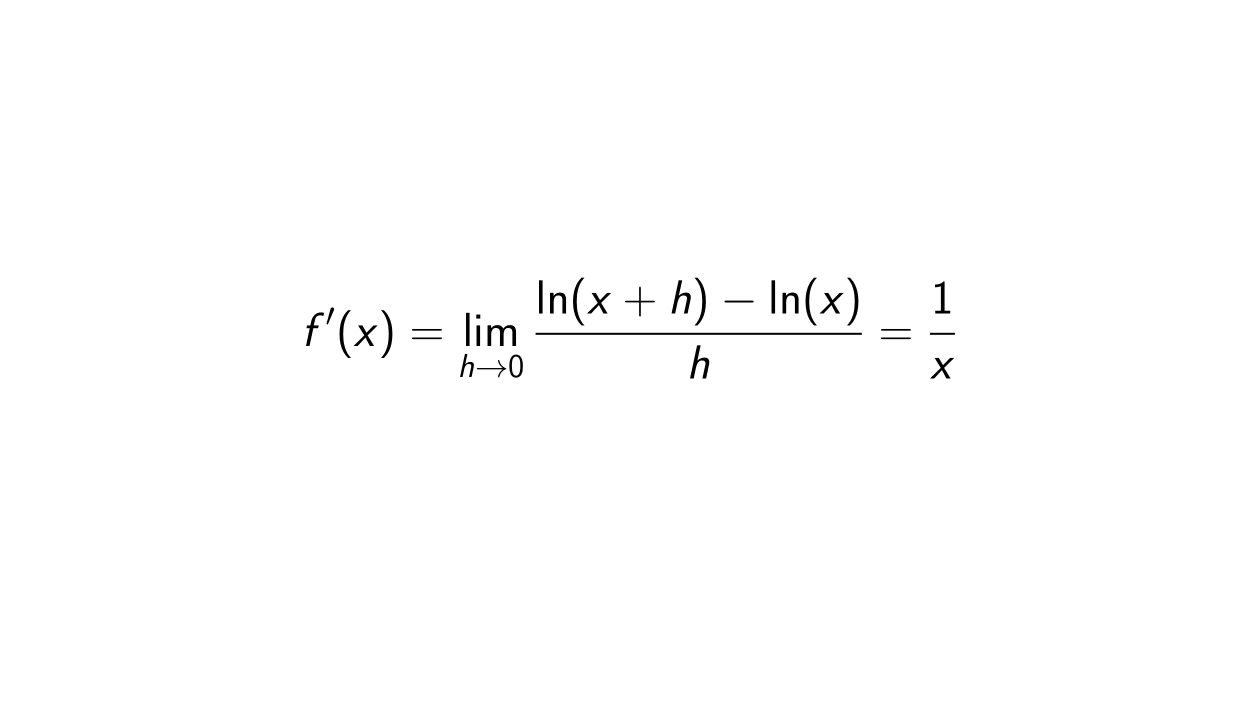How To Differentiate Ln X - We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
We Can Use A Formula To Find The Derivative Of \(Y=\Ln X\), And The Relationship \(Log_Bx=\Frac{\Ln X}{\Ln B}\) Allows Us To Extend.
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.