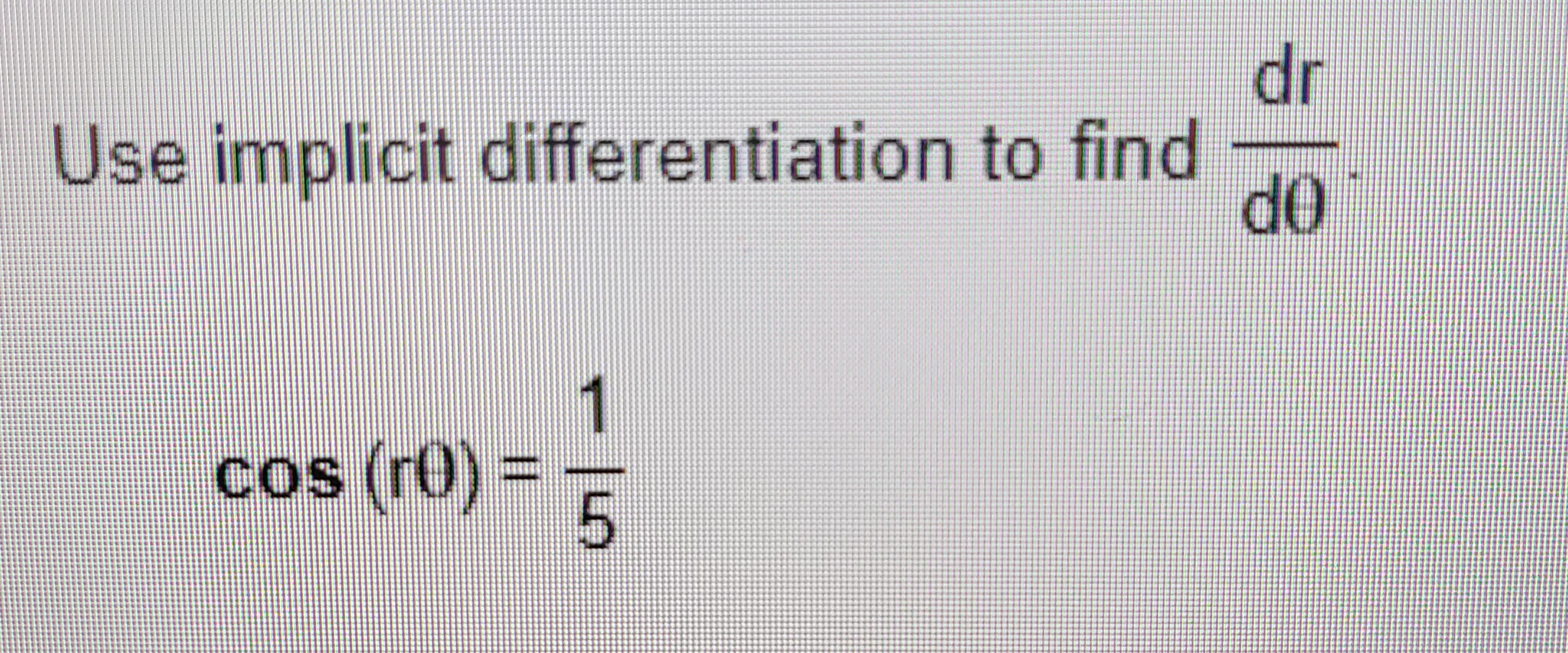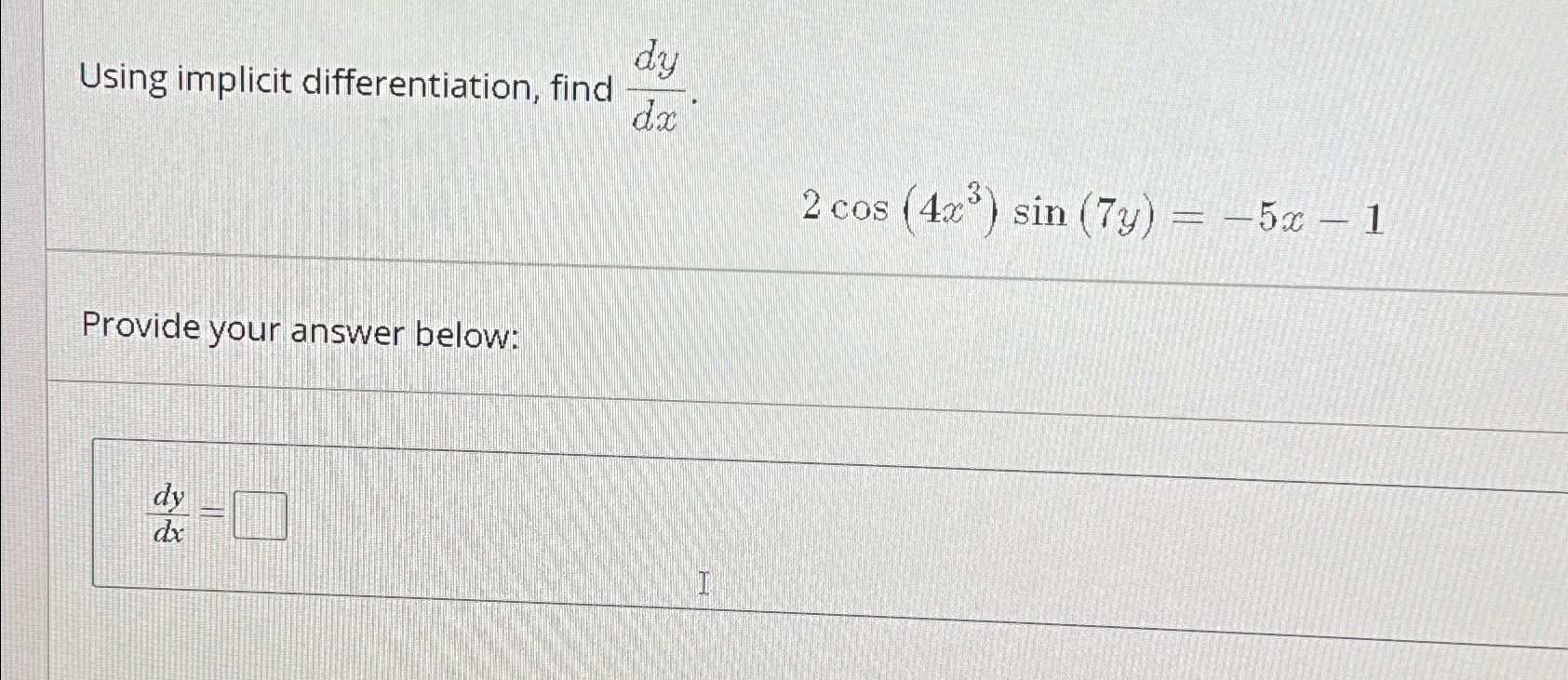Implicit Differentiation Help Cos - Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. In these lessons, we will learn how implicit differentiation can be used the find the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In this section we will discuss implicit differentiation.
Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In this section we will discuss implicit differentiation. In these lessons, we will learn how implicit differentiation can be used the find the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Implicit differentiation is useful for, among other things, finding tangent and normal lines to a.
Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this section we will discuss implicit differentiation. In these lessons, we will learn how implicit differentiation can be used the find the. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$
How to Do Implicit Differentiation 7 Steps (with Pictures)
In this section we will discuss implicit differentiation. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In these lessons, we will learn how implicit differentiation can be used the find the.
intro to Implicit differentiation PDF Free Download
In these lessons, we will learn how implicit differentiation can be used the find the. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this section we will discuss implicit differentiation.
Solved Use implicit differentiation to find drdθ.cos(rθ)=15
Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In these lessons, we will learn how implicit differentiation can be used the find the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this section we will discuss implicit differentiation. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a.
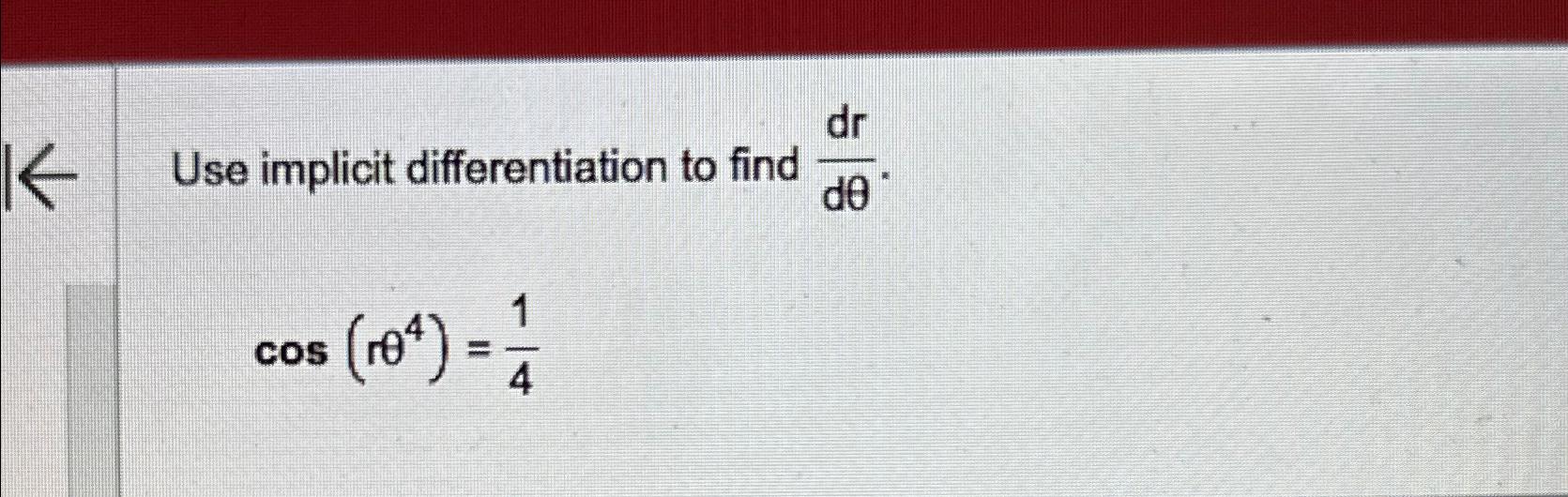
Solved Use implicit differentiation to find drdθ.cos(rθ4)=14
In these lessons, we will learn how implicit differentiation can be used the find the. In this section we will discuss implicit differentiation. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ Implicit differentiation is useful for, among other things, finding tangent and normal lines to a.
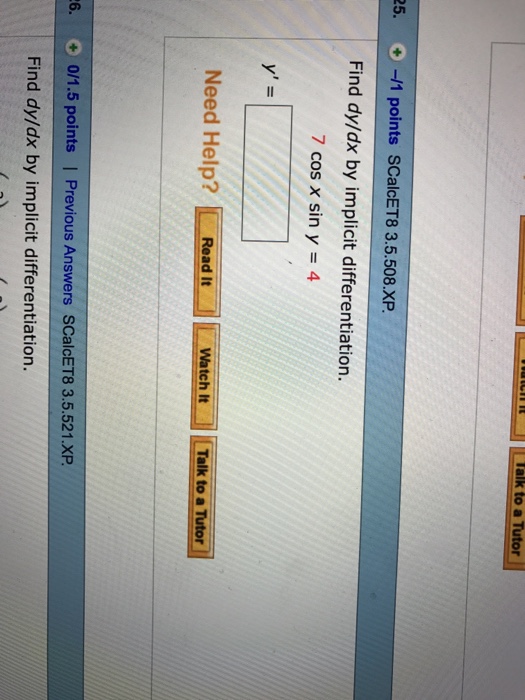
Solved Find dy/dx by implicit differentiation. 8 cos x sin y
In this section we will discuss implicit differentiation. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. In these lessons, we will learn how implicit differentiation can be used the find the.
Solved Using implicit differentiation, find
In these lessons, we will learn how implicit differentiation can be used the find the. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In this section we will discuss implicit differentiation. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more
Solved 4. Implicit Differentiation a. Find it cos(xy) = cos
Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In these lessons, we will learn how implicit differentiation can be used the find the. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. In this section we will discuss implicit differentiation. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more
Solved Find dy/dx by implicit differentiation. 7 cos x sin
Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In this section we will discuss implicit differentiation. In these lessons, we will learn how implicit differentiation can be used the find the. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more
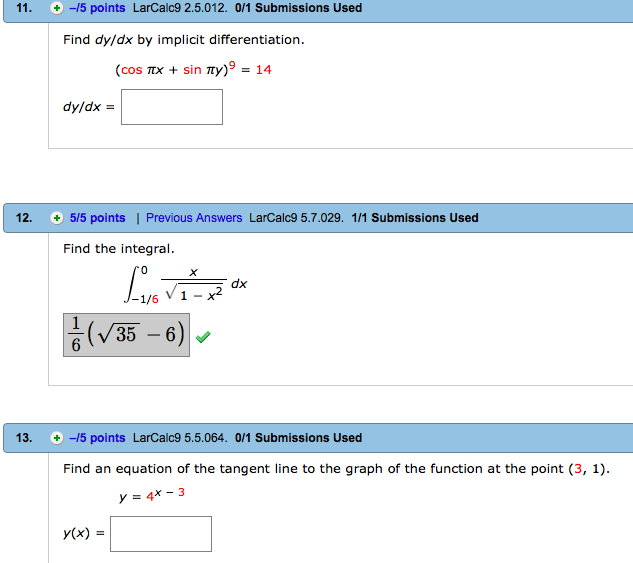
Solved Find dy/dx by implicit differentiation. (cos pix +
\int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this section we will discuss implicit differentiation. In these lessons, we will learn how implicit differentiation can be used the find the. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ Implicit differentiation is useful for, among other things, finding tangent and normal lines to a.
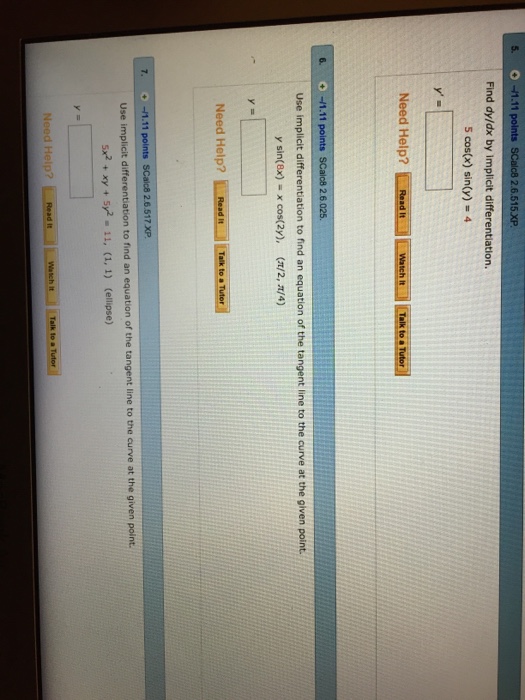
Solved Find dy/dx by implicit differentiation. 5 cos (x)
Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$ In these lessons, we will learn how implicit differentiation can be used the find the. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. In this section we will discuss implicit differentiation. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more
In This Section We Will Discuss Implicit Differentiation.
\int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In these lessons, we will learn how implicit differentiation can be used the find the. Implicit differentiation is useful for, among other things, finding tangent and normal lines to a. Use logarithmic differentiation to find the derivative of $y = (\cos{(x))}^{ln(x)}$