Is A Cusp Differentiable - I'm trying to grasp what's going on at a cusp geometrically. A cusp is a point where you have a vertical tangent, but with the following property: For instance, $y^2=x^3$ is not. If the graph of a function has a sharp corner (also known as a corner point) or a. A function is not differentiable at a point if it has a sharp corner.
A function is not differentiable at a point if it has a sharp corner. A cusp is a point where you have a vertical tangent, but with the following property: I'm trying to grasp what's going on at a cusp geometrically. For instance, $y^2=x^3$ is not. If the graph of a function has a sharp corner (also known as a corner point) or a.
If the graph of a function has a sharp corner (also known as a corner point) or a. I'm trying to grasp what's going on at a cusp geometrically. A function is not differentiable at a point if it has a sharp corner. For instance, $y^2=x^3$ is not. A cusp is a point where you have a vertical tangent, but with the following property:
CUSP© by CUSP on Dribbble
If the graph of a function has a sharp corner (also known as a corner point) or a. For instance, $y^2=x^3$ is not. A function is not differentiable at a point if it has a sharp corner. A cusp is a point where you have a vertical tangent, but with the following property: I'm trying to grasp what's going on.
Cusp Names Diagram Quizlet
A function is not differentiable at a point if it has a sharp corner. For instance, $y^2=x^3$ is not. If the graph of a function has a sharp corner (also known as a corner point) or a. I'm trying to grasp what's going on at a cusp geometrically. A cusp is a point where you have a vertical tangent, but.
The Frisky TaurusGemini Cusp
If the graph of a function has a sharp corner (also known as a corner point) or a. A cusp is a point where you have a vertical tangent, but with the following property: A function is not differentiable at a point if it has a sharp corner. For instance, $y^2=x^3$ is not. I'm trying to grasp what's going on.
Behavior Cusp Definition & Examples
If the graph of a function has a sharp corner (also known as a corner point) or a. A function is not differentiable at a point if it has a sharp corner. A cusp is a point where you have a vertical tangent, but with the following property: I'm trying to grasp what's going on at a cusp geometrically. For.
Discover The Cusp
For instance, $y^2=x^3$ is not. If the graph of a function has a sharp corner (also known as a corner point) or a. A cusp is a point where you have a vertical tangent, but with the following property: A function is not differentiable at a point if it has a sharp corner. I'm trying to grasp what's going on.
Mini cusp hoops Goldplated DAMERNES MAGASIN
I'm trying to grasp what's going on at a cusp geometrically. For instance, $y^2=x^3$ is not. A function is not differentiable at a point if it has a sharp corner. If the graph of a function has a sharp corner (also known as a corner point) or a. A cusp is a point where you have a vertical tangent, but.
Education The Cusp
If the graph of a function has a sharp corner (also known as a corner point) or a. A cusp is a point where you have a vertical tangent, but with the following property: For instance, $y^2=x^3$ is not. I'm trying to grasp what's going on at a cusp geometrically. A function is not differentiable at a point if it.
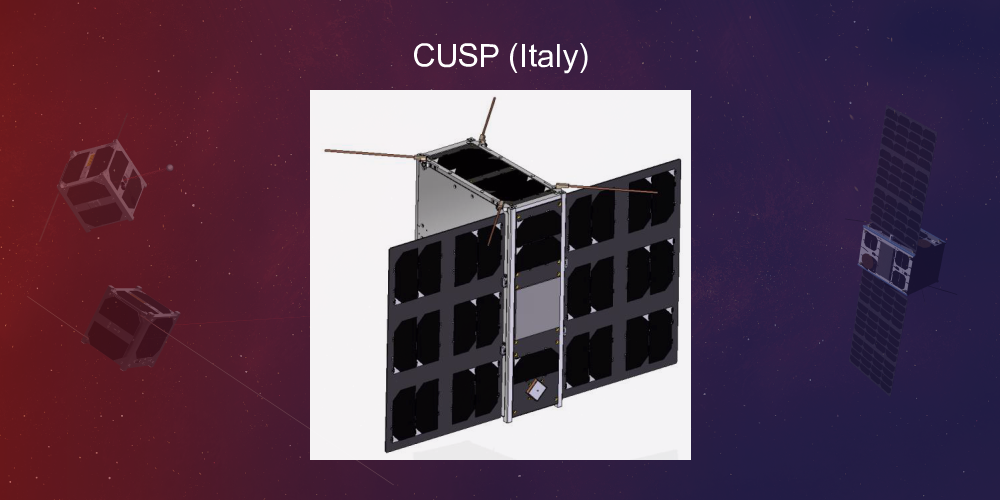
CUSP (Italy) Spacecraft Nanosats Database
If the graph of a function has a sharp corner (also known as a corner point) or a. I'm trying to grasp what's going on at a cusp geometrically. A cusp is a point where you have a vertical tangent, but with the following property: A function is not differentiable at a point if it has a sharp corner. For.
CUSP login
If the graph of a function has a sharp corner (also known as a corner point) or a. For instance, $y^2=x^3$ is not. I'm trying to grasp what's going on at a cusp geometrically. A function is not differentiable at a point if it has a sharp corner. A cusp is a point where you have a vertical tangent, but.
φ is not differentiable at the common cusp point of μ 1,2. Download
A function is not differentiable at a point if it has a sharp corner. If the graph of a function has a sharp corner (also known as a corner point) or a. A cusp is a point where you have a vertical tangent, but with the following property: I'm trying to grasp what's going on at a cusp geometrically. For.
A Cusp Is A Point Where You Have A Vertical Tangent, But With The Following Property:
A function is not differentiable at a point if it has a sharp corner. I'm trying to grasp what's going on at a cusp geometrically. For instance, $y^2=x^3$ is not. If the graph of a function has a sharp corner (also known as a corner point) or a.