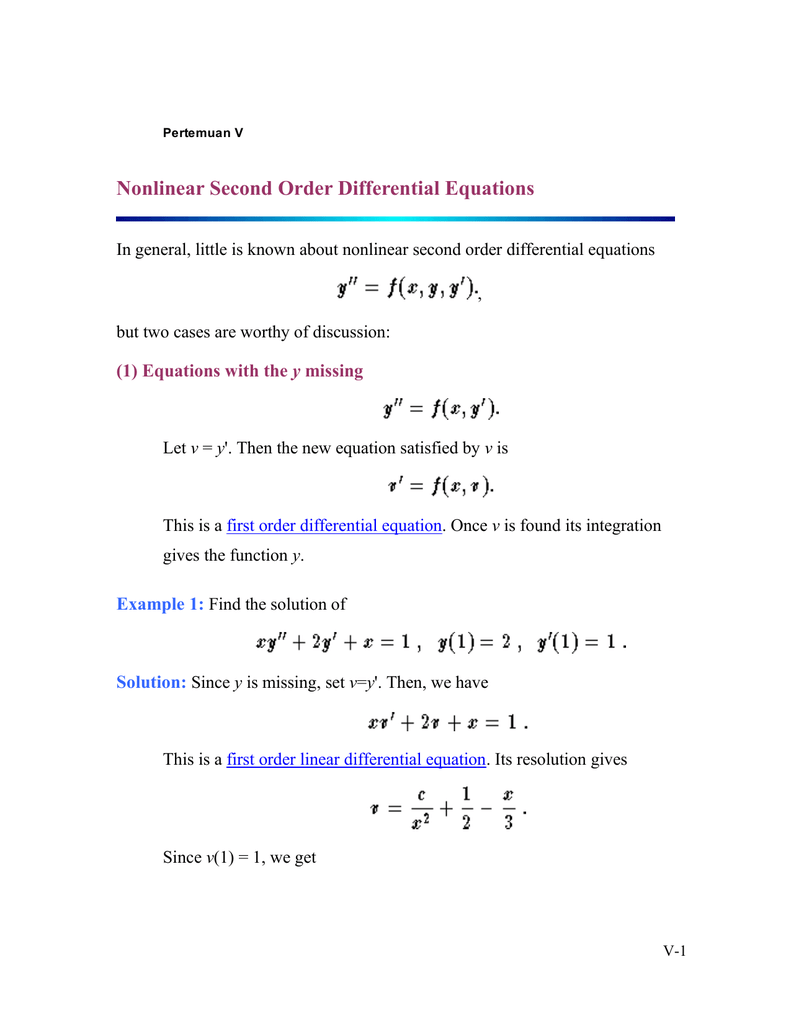
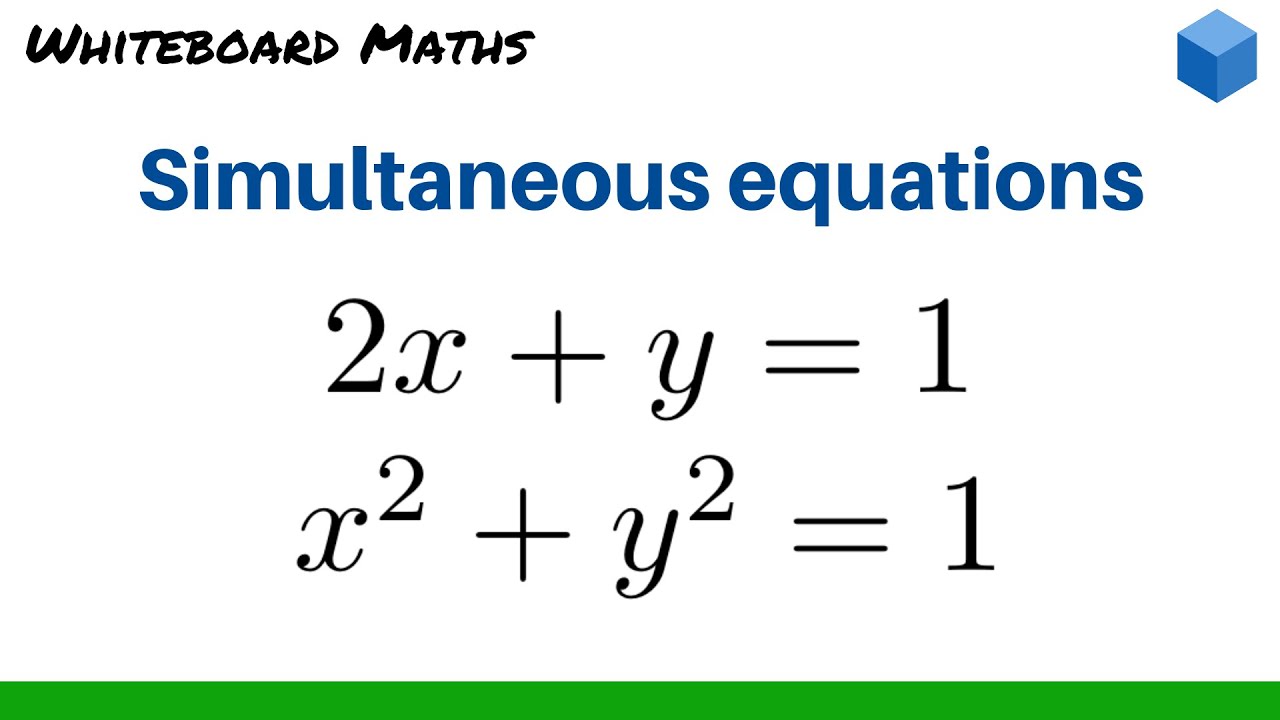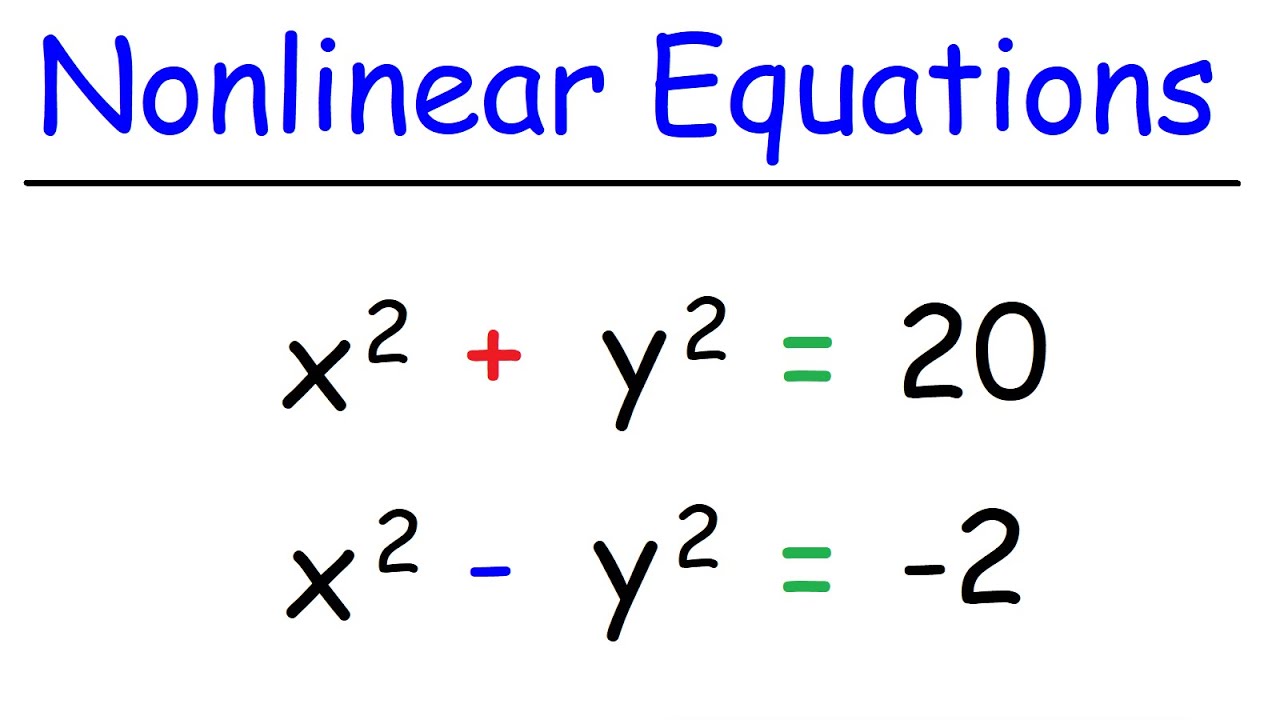Linear And Nonlinear Differential Equations Examples - Linear and nonlinear differential equations are two classifications within the vast field of. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. In a differential equation, when the variables and their derivatives are only. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x.
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. In a differential equation, when the variables and their derivatives are only. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. Linear and nonlinear differential equations are two classifications within the vast field of.
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. Linear and nonlinear differential equations are two classifications within the vast field of. In a differential equation, when the variables and their derivatives are only.
Partial Differential Equations Examples
Linear and nonlinear differential equations are two classifications within the vast field of. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. In a differential.
Second Order Differential Equations
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. The logistic equation introduces the first example of. Differential equations are classified into linear des or nonlinear des. Linear and nonlinear differential equations are two classifications within the vast field of. In a differential.
(PDF) Solving System of First Order Linear and Differential
Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. In a differential equation, when the variables and their derivatives are only. Linear and nonlinear differential.
RealWorld Modeling with (Linear & Differential Equations)
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Differential equations are classified into linear des or nonlinear des. Linear and nonlinear differential equations are two classifications within the vast field of. In a differential equation, when the variables and their derivatives are.
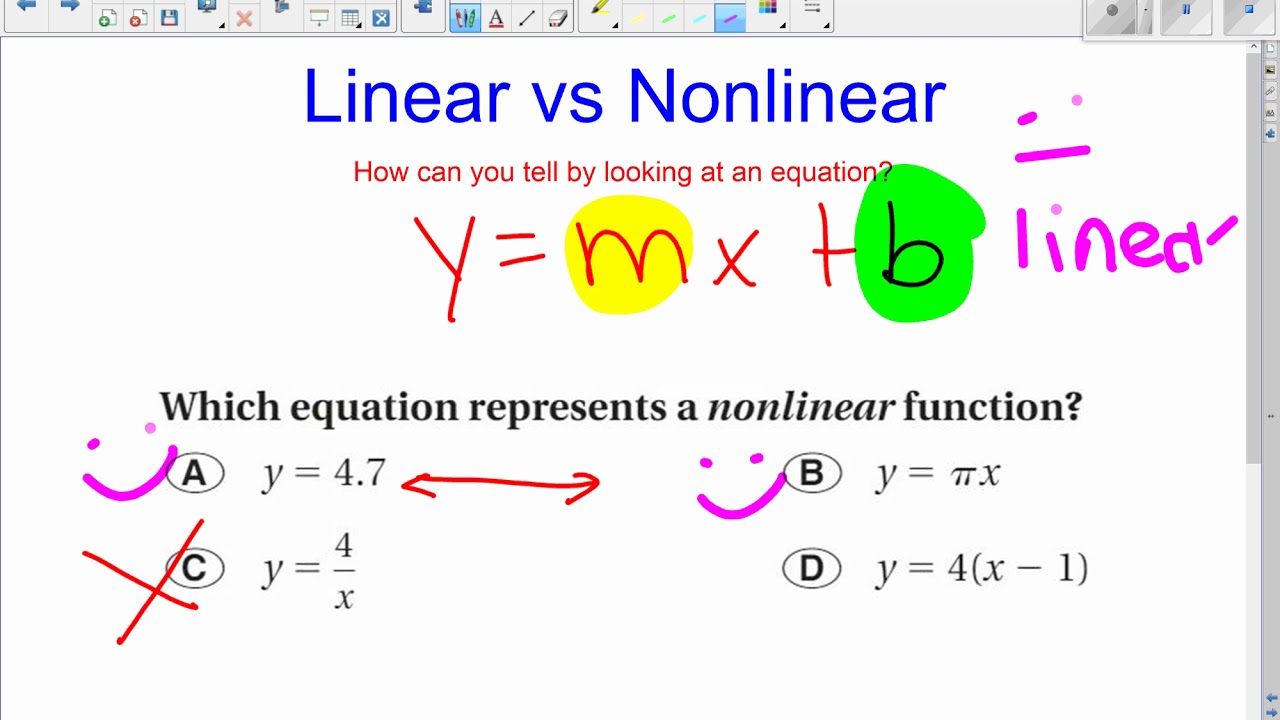
Linear Versus Equations
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. The logistic equation introduces the first example of. In a differential equation, when the variables and their derivatives are only. Linear and nonlinear differential equations are two classifications within the vast field of. Differential.
SOLUTION linear and non linear differential equation examples
Differential equations are classified into linear des or nonlinear des. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Linear and nonlinear differential equations are two classifications within the vast field of. In a differential equation, when the variables and their derivatives are.
Linear Versus Equations
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Linear and nonlinear differential equations are two classifications within the vast field of. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. In a differential.
PPT Differences Between Linear and Equations PowerPoint
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Differential equations are classified into linear des or nonlinear des. The logistic equation introduces the first example of. In a differential equation, when the variables and their derivatives are only. Linear and nonlinear differential.
And Linear Equations
Linear and nonlinear differential equations are two classifications within the vast field of. In a differential equation, when the variables and their derivatives are only. The logistic equation introduces the first example of. Differential equations are classified into linear des or nonlinear des. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after.
Solved Classify each differential equations as linear or
Differential equations are classified into linear des or nonlinear des. In a differential equation, when the variables and their derivatives are only. The logistic equation introduces the first example of. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x. Linear and nonlinear differential.
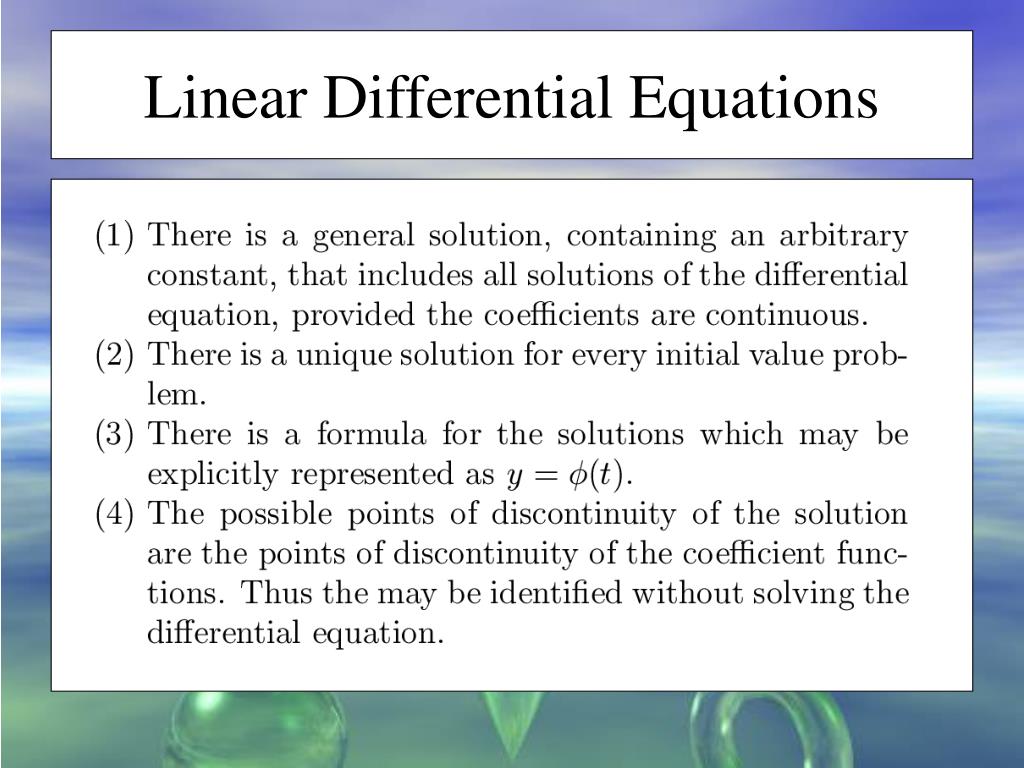
Differential Equations Are Classified Into Linear Des Or Nonlinear Des.
Linear and nonlinear differential equations are two classifications within the vast field of. In a differential equation, when the variables and their derivatives are only. The logistic equation introduces the first example of. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x.