Matrices Differential Equations - In this section we will give a brief review of matrices and vectors. U(t) = c1eλ1tx1 + c2eλ2tx2. In this session we will learn the basic linear theory for systems. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Is eλ1tx 1 really a solution to d dt u =. We will also see how we can write.
Is eλ1tx 1 really a solution to d dt u =. In this session we will learn the basic linear theory for systems. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. We will also see how we can write. In this section we will give a brief review of matrices and vectors.
In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =. In this session we will learn the basic linear theory for systems. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. We will also see how we can write.
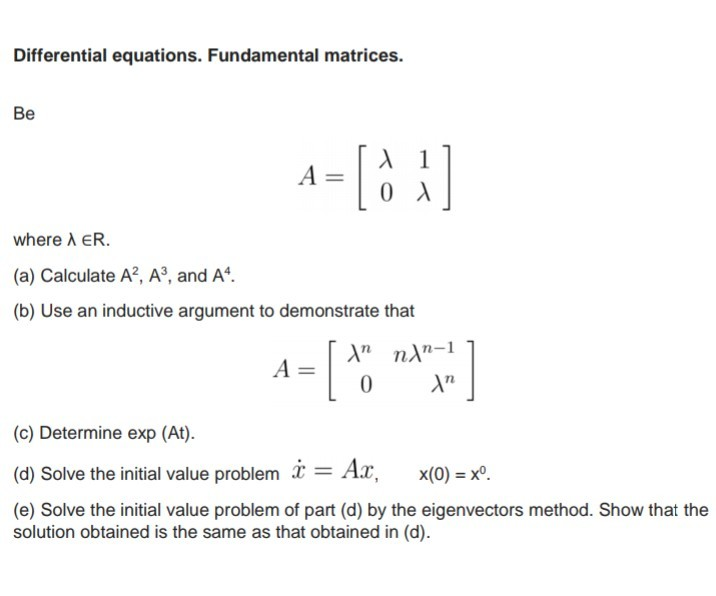
Solved Differential equations. Fundamental matrices. Be
We will also see how we can write. In this session we will learn the basic linear theory for systems. U(t) = c1eλ1tx1 + c2eλ2tx2. Is eλ1tx 1 really a solution to d dt u =. In this section we will give a brief review of matrices and vectors.
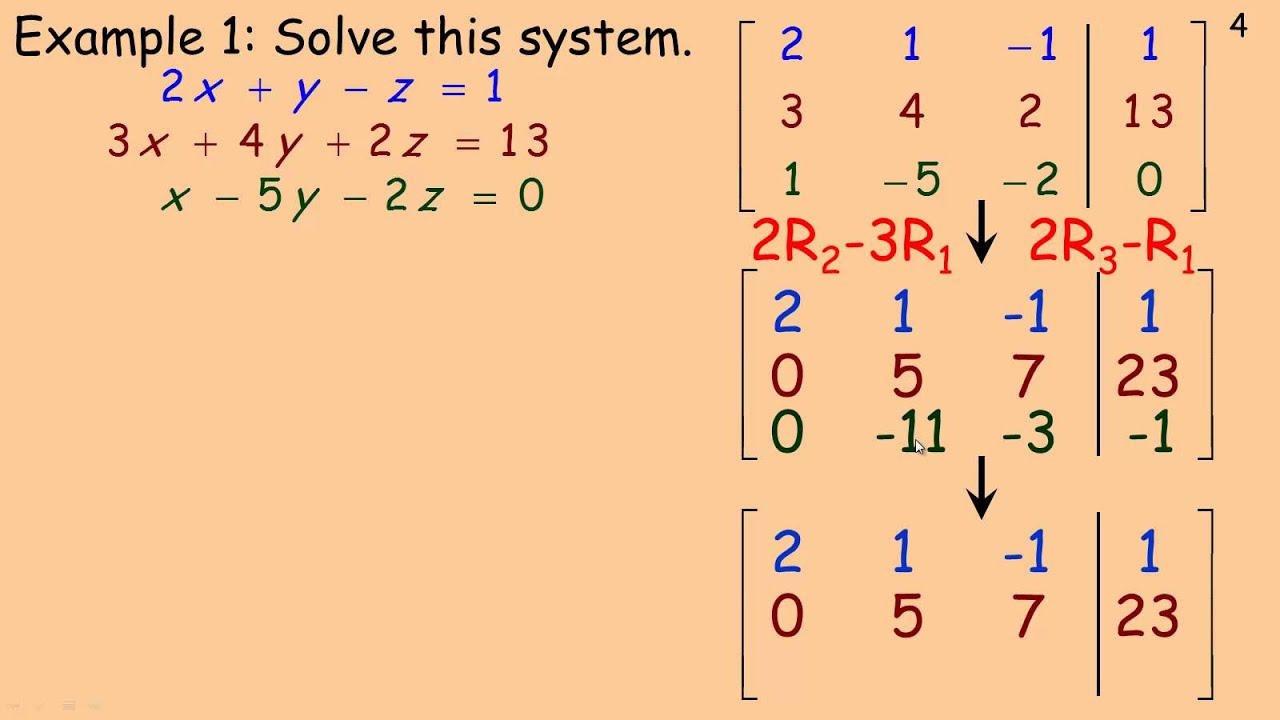
Solving 3 Systems Of Equations Using Matrices Tessshebaylo
In this session we will learn the basic linear theory for systems. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. We will also see how we can write. Is eλ1tx 1 really a solution to d dt u =. In this section we will give a brief review of matrices and vectors.
MATRICES Using matrices to solve Systems of Equations
We will also see how we can write. In this section we will give a brief review of matrices and vectors. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Is eλ1tx 1 really a solution to d dt u =.
Matrices And Linear System Of Equations Pdf Tessshebaylo
U(t) = c1eλ1tx1 + c2eλ2tx2. In this session we will learn the basic linear theory for systems. We will also see how we can write. In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =.
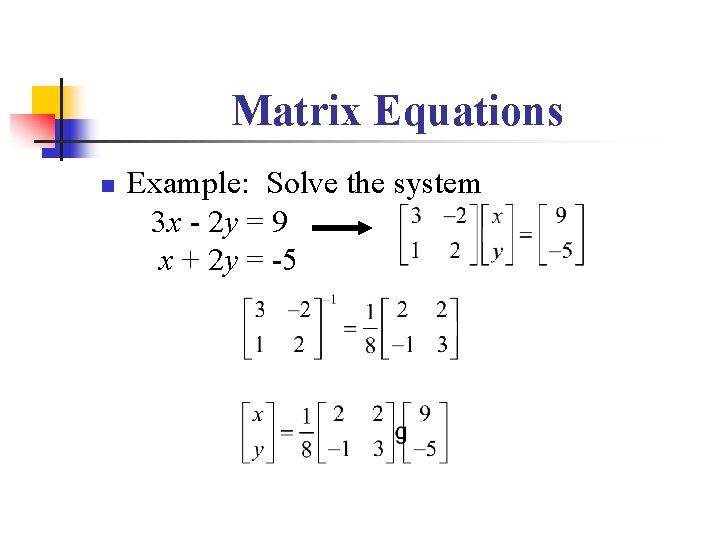
Solve 2 Variable Equations Using Matrices Tessshebaylo
We will also see how we can write. In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =. In this session we will learn the basic linear theory for systems. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
MATRICES Using matrices to solve Systems of Equations
We will also see how we can write. U(t) = c1eλ1tx1 + c2eλ2tx2. In this session we will learn the basic linear theory for systems. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. In this section we will give a brief review of matrices and vectors.
Modelling Motion with Differential Equations
Is eλ1tx 1 really a solution to d dt u =. In this session we will learn the basic linear theory for systems. We will also see how we can write. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
(PDF) Some linear differential equations generated by matrices
In this session we will learn the basic linear theory for systems. Is eλ1tx 1 really a solution to d dt u =. In this section we will give a brief review of matrices and vectors. We will also see how we can write. U(t) = c1eλ1tx1 + c2eλ2tx2.
Matrices differential equations
U(t) = c1eλ1tx1 + c2eλ2tx2. In this section we will give a brief review of matrices and vectors. In this session we will learn the basic linear theory for systems. Is eλ1tx 1 really a solution to d dt u =. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
(PDF) Compound matrices and ordinary differential equations
In this session we will learn the basic linear theory for systems. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. We will also see how we can write. In this section we will give a brief review of matrices and vectors. U(t) = c1eλ1tx1 + c2eλ2tx2.
In This Session We Will Learn The Basic Linear Theory For Systems.
We will also see how we can write. Is eλ1tx 1 really a solution to d dt u =. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. U(t) = c1eλ1tx1 + c2eλ2tx2.