Non Homogeneous First Order Differential Equation - An example of a first order linear non. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =. (we use c1 to save c for later.) p(t)dt. We can find the so lution as follows: We’ve shown you how to use integrating factors to write the general equation for a first order non. In this section we will discuss the basics of solving nonhomogeneous differential. In this section, we examine how to solve nonhomogeneous differential equations.
In this section, we examine how to solve nonhomogeneous differential equations. In this section we will discuss the basics of solving nonhomogeneous differential. (we use c1 to save c for later.) p(t)dt. We can find the so lution as follows: We’ve shown you how to use integrating factors to write the general equation for a first order non. An example of a first order linear non. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =.
Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =. In this section we will discuss the basics of solving nonhomogeneous differential. We’ve shown you how to use integrating factors to write the general equation for a first order non. We can find the so lution as follows: An example of a first order linear non. In this section, we examine how to solve nonhomogeneous differential equations. (we use c1 to save c for later.) p(t)dt.
Solving 2nd Order non homogeneous differential equation using Wronskian
An example of a first order linear non. We can find the so lution as follows: (we use c1 to save c for later.) p(t)dt. In this section, we examine how to solve nonhomogeneous differential equations. We’ve shown you how to use integrating factors to write the general equation for a first order non.
Solved Consider the first order nonhomogeneous differential
In this section, we examine how to solve nonhomogeneous differential equations. We’ve shown you how to use integrating factors to write the general equation for a first order non. (we use c1 to save c for later.) p(t)dt. In this section we will discuss the basics of solving nonhomogeneous differential. Let us first focus on the nonhomogeneous first order equation.
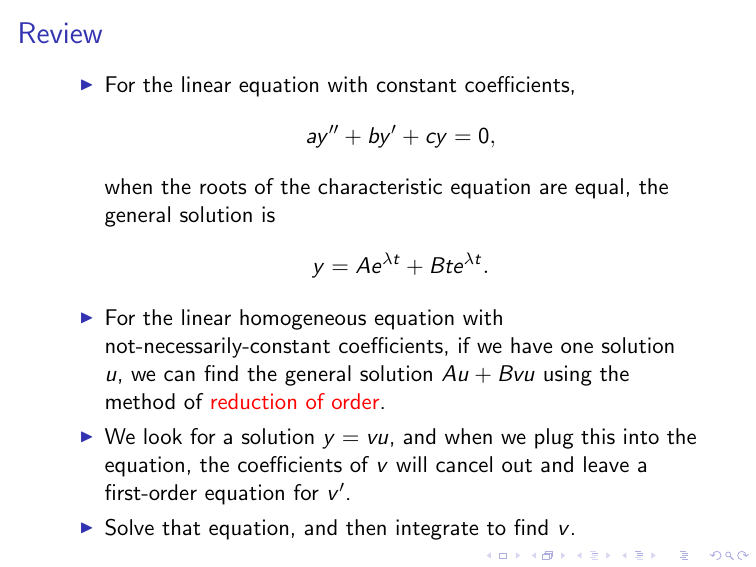
First Order Linear Homogeneous Differential Equation Examples
In this section we will discuss the basics of solving nonhomogeneous differential. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =. In this section, we examine how to solve nonhomogeneous differential equations. An example of a first order linear non. (we use c1 to save c for later.) p(t)dt.
Answered Consider the following nonhomogeneous… bartleby
In this section, we examine how to solve nonhomogeneous differential equations. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =. We’ve shown you how to use integrating factors to write the general equation for a first order non. An example of a first order linear non. (we use c1 to save c for later.) p(t)dt.
SOLUTION Homogeneous first order example 2 differential equation
We’ve shown you how to use integrating factors to write the general equation for a first order non. In this section we will discuss the basics of solving nonhomogeneous differential. An example of a first order linear non. We can find the so lution as follows: (we use c1 to save c for later.) p(t)dt.
Solving a nonhomogeneous equation
In this section we will discuss the basics of solving nonhomogeneous differential. An example of a first order linear non. In this section, we examine how to solve nonhomogeneous differential equations. We can find the so lution as follows: We’ve shown you how to use integrating factors to write the general equation for a first order non.
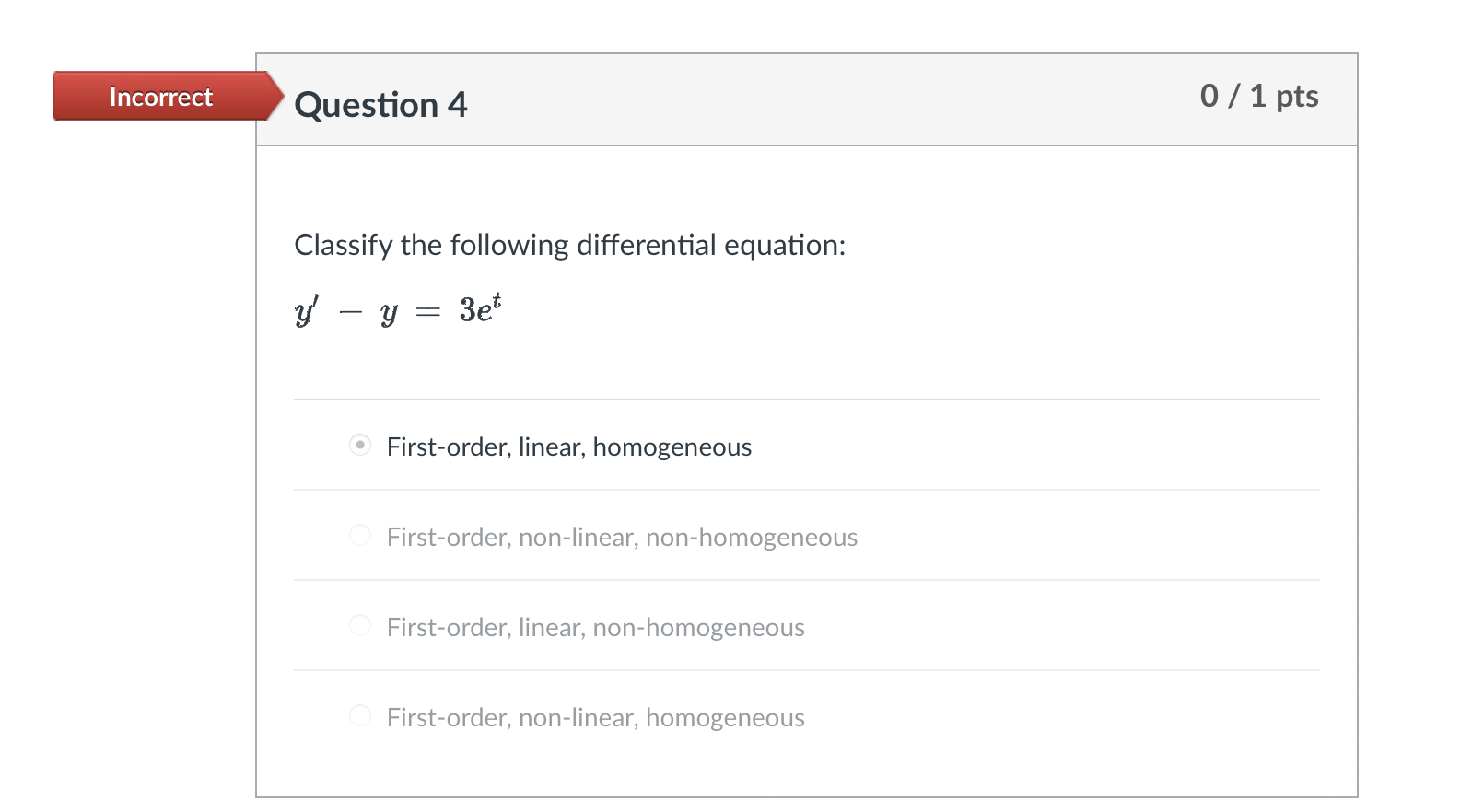
SOLVED Incorrect Question 4 0 / 1 pts Classify the following
We’ve shown you how to use integrating factors to write the general equation for a first order non. (we use c1 to save c for later.) p(t)dt. In this section we will discuss the basics of solving nonhomogeneous differential. An example of a first order linear non. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =.
First Order Differential Equation
We can find the so lution as follows: (we use c1 to save c for later.) p(t)dt. In this section, we examine how to solve nonhomogeneous differential equations. In this section we will discuss the basics of solving nonhomogeneous differential. We’ve shown you how to use integrating factors to write the general equation for a first order non.
(PDF) Solution of First Order Linear Non Homogeneous Ordinary
We’ve shown you how to use integrating factors to write the general equation for a first order non. In this section we will discuss the basics of solving nonhomogeneous differential. In this section, we examine how to solve nonhomogeneous differential equations. An example of a first order linear non. Let us first focus on the nonhomogeneous first order equation \begin{equation*}.
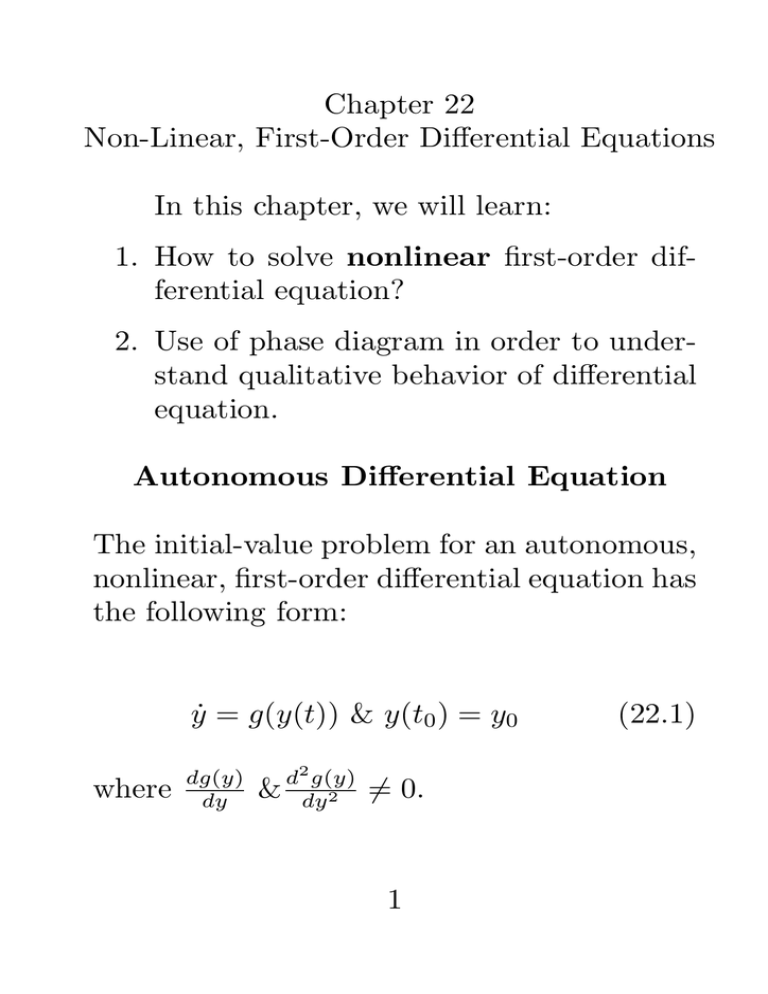
SOLVED Activity 2 Give one example of a secondorder nonhomogeneous
In this section we will discuss the basics of solving nonhomogeneous differential. (we use c1 to save c for later.) p(t)dt. In this section, we examine how to solve nonhomogeneous differential equations. We can find the so lution as follows: An example of a first order linear non.
An Example Of A First Order Linear Non.
In this section, we examine how to solve nonhomogeneous differential equations. We’ve shown you how to use integrating factors to write the general equation for a first order non. Let us first focus on the nonhomogeneous first order equation \begin{equation*} {\vec{x}}'(t) =. In this section we will discuss the basics of solving nonhomogeneous differential.
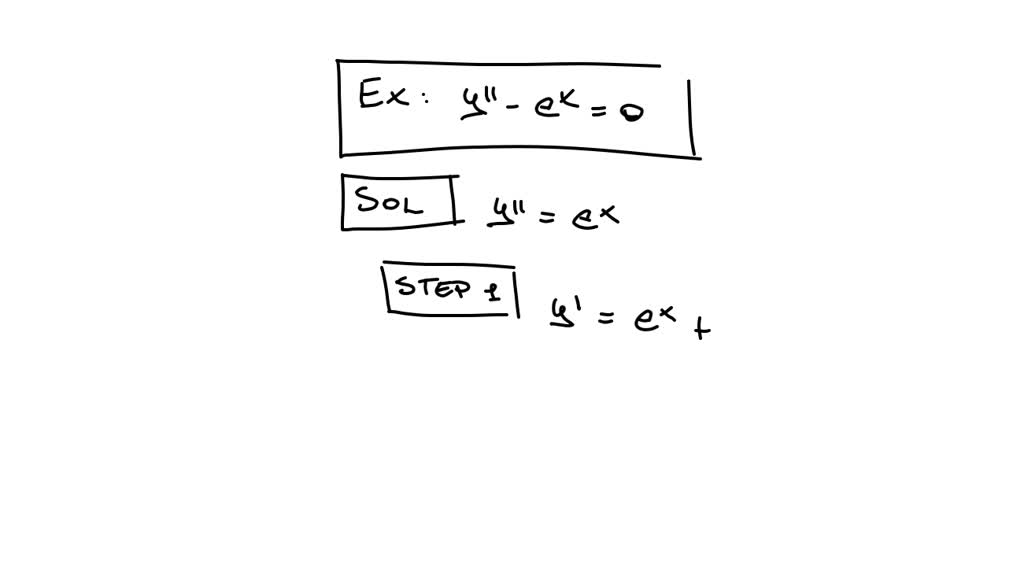
We Can Find The So Lution As Follows:
(we use c1 to save c for later.) p(t)dt.