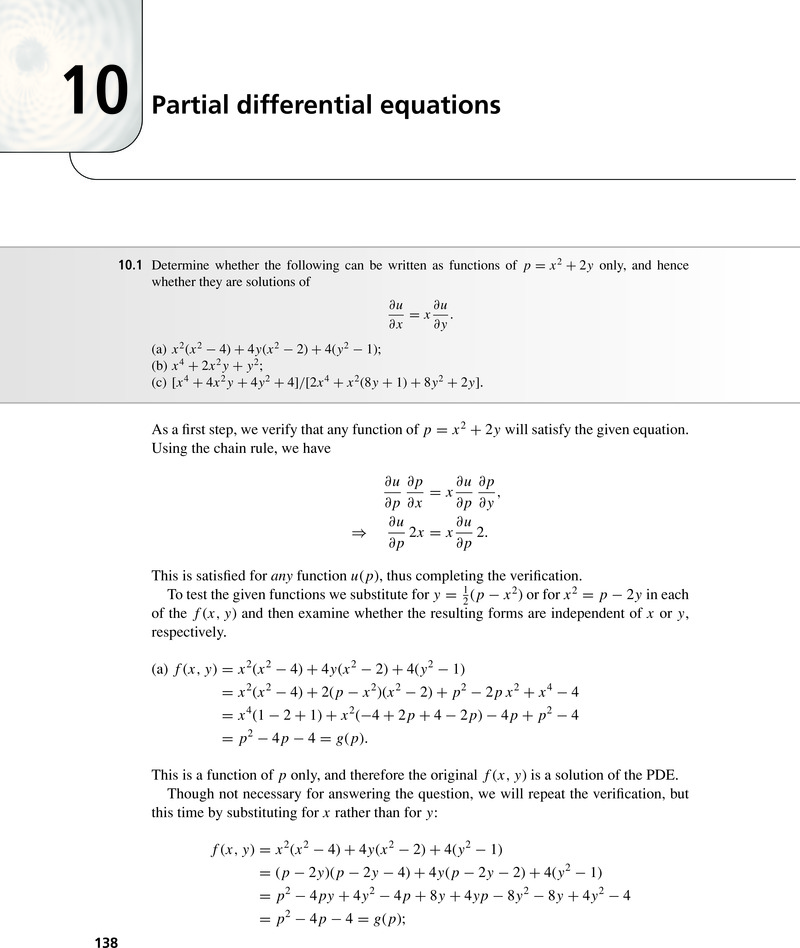
Partial Differential Equations Evans Solutions - We have _z(s) = ut(x+bs; Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. T+s) = cz(s), thus the pde reduces to. Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Partial differential equations by evans (appendix). Then, z(t) = u(x bt;0) = g(x bt) = dect. We can solve for d by letting s = t.
Then, z(t) = u(x bt;0) = g(x bt) = dect. Partial differential equations by evans (appendix). Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. We have _z(s) = ut(x+bs; T+s) = cz(s), thus the pde reduces to. We can solve for d by letting s = t. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book.
Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Then, z(t) = u(x bt;0) = g(x bt) = dect. Partial differential equations by evans (appendix). T+s) = cz(s), thus the pde reduces to. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. We have _z(s) = ut(x+bs; We can solve for d by letting s = t.
Partial Differential Equations Theory, Numerical Methods and IllPosed
Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Then, z(t) = u(x bt;0) = g(x bt) = dect. We can solve for d by letting s = t. Partial differential equations by evans (appendix).
Partial Differential Equations Evans Solutions Manual PDF Partial
We have _z(s) = ut(x+bs; Then, z(t) = u(x bt;0) = g(x bt) = dect. We can solve for d by letting s = t. Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Partial differential equations by evans (appendix).
Partial differential equations (Chapter 10) Student Solution Manual
Partial differential equations by evans (appendix). Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. Then, z(t) = u(x bt;0) = g(x bt) = dect. We can solve for d by letting s = t. T+s) = cz(s), thus the pde reduces to.
PPT Partial Differential Equations PowerPoint Presentation ID353900
We can solve for d by letting s = t. Partial differential equations by evans (appendix). Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. Then, z(t) = u(x bt;0) = g(x bt) = dect.
Partial differential equations PPT
Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. We have _z(s) = ut(x+bs; We can solve for d by letting s = t. T+s) = cz(s), thus the pde reduces to.
Partial Differential Equations (Lawrence C. Evans) (ZLibrary)
Partial differential equations by evans (appendix). T+s) = cz(s), thus the pde reduces to. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. Then, z(t) = u(x bt;0) = g(x bt) = dect. We have _z(s) = ut(x+bs;
PARTIAL DIFFERENTIAL EQUATIONS (2ND EDN) (English) LAWRENCE C.EVANS
Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. T+s) = cz(s), thus the pde reduces to. We have _z(s) = ut(x+bs; Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. We can solve for d by letting s = t.
Partial differential equations PPT
Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. We can solve for d by letting s = t. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. T+s) = cz(s), thus the pde reduces to. Then, z(t) = u(x bt;0) = g(x bt) = dect.
Lawrence C. EvansPartial Differential Equations385394 PDF
We can solve for d by letting s = t. Partial differential equations by evans (appendix). Then, z(t) = u(x bt;0) = g(x bt) = dect. Evans pde solutions for ch2 and ch3 osman akar july 2016 this document is written for the book. We have _z(s) = ut(x+bs;
(PDF) Solution of Partial Differential Equations Combination of
We can solve for d by letting s = t. T+s) = cz(s), thus the pde reduces to. Partial differential equations by evans (appendix). Then, z(t) = u(x bt;0) = g(x bt) = dect. Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021.
We Have _Z(S) = Ut(X+Bs;
T+s) = cz(s), thus the pde reduces to. Solutions to partial differential equations by lawrence evans matthew kehoe may 22, 2021. Partial differential equations by evans (appendix). We can solve for d by letting s = t.
Evans Pde Solutions For Ch2 And Ch3 Osman Akar July 2016 This Document Is Written For The Book.
Then, z(t) = u(x bt;0) = g(x bt) = dect.