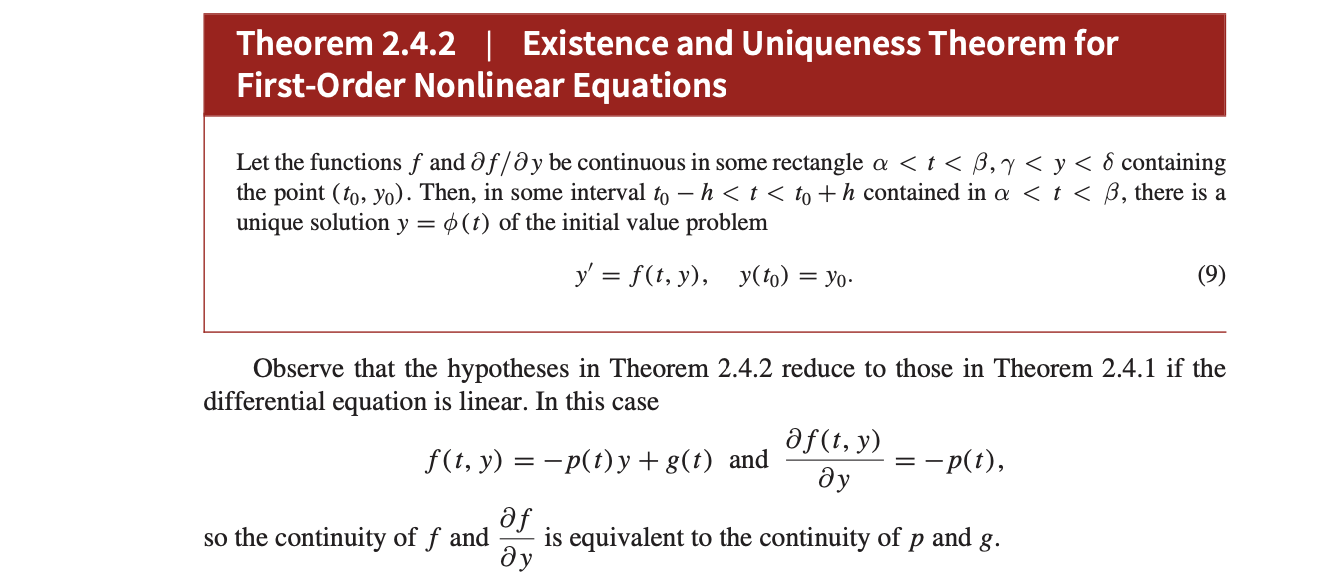
Uniqueness Theorem For Differential Equations - Notes on the existence and uniqueness theorem for first order differential equations i. Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Then the differential equation (2) with initial con. (b) is a uniqueness theorem. The existence and uniqueness of solutions to differential equations 5 theorem 3.9.
(b) is a uniqueness theorem. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Then the differential equation (2) with initial con. Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Notes on the existence and uniqueness theorem for first order differential equations i.
Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. I!rnis a solution to x_ = v(t;x) with. (b) is a uniqueness theorem. Let the function f(t,y) be continuous and satisfy the bound (3). It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). The existence and uniqueness of solutions to differential equations 5 theorem 3.9.
ordinary differential equations Application of Picard's existence
The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Then the differential equation (2) with initial con. I!rnis a solution to x_ = v(t;x) with. Notes on the existence and uniqueness theorem for first order differential equations i. (b) is a uniqueness theorem.
Solved Theorem 2.4.1 Existence and Uniqueness Theorem for
I!rnis a solution to x_ = v(t;x) with. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Then the differential equation (2) with initial con. Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for first order differential equations i.
PPT Existence and Uniqueness Theorem (Local Theorem) PowerPoint
The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous and satisfy the bound (3). Then the differential equation (2) with initial con. I!rnis a solution to x_ = v(t;x) with. Notes on the existence and uniqueness theorem for first order differential equations i.
(PDF) Existence and Uniqueness Theorem for Uncertain Delay Differential
I!rnis a solution to x_ = v(t;x) with. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Notes on the existence and uniqueness theorem for first order differential equations i. (b) is a uniqueness theorem. Then the differential equation (2) with initial con.
Differential Equations Existence and Uniqueness Theorem Is my answer
(b) is a uniqueness theorem. Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for first order differential equations i. I!rnis a solution to x_ = v(t;x) with. Then the differential equation (2) with initial con.
SOLUTION Differential Equations) Initial value problem Uniqueness and
Then the differential equation (2) with initial con. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with. The existence and uniqueness of solutions to differential equations 5 theorem 3.9.
Delay differential equation uniqueness Please help ( Mathematics
Notes on the existence and uniqueness theorem for first order differential equations i. Let the function f(t,y) be continuous and satisfy the bound (3). Then the differential equation (2) with initial con. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). I!rnis a solution to x_ = v(t;x) with.
(PDF) A Picard Theorem for iterative differential equations
(b) is a uniqueness theorem. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). Notes on the existence and uniqueness theorem for first order differential equations i. I!rnis a solution to x_ = v(t;x) with. The existence and uniqueness of solutions to differential equations 5 theorem 3.9.
Lesson 7 Existence And Uniqueness Theorem (Differential Equations
I!rnis a solution to x_ = v(t;x) with. Let the function f(t,y) be continuous and satisfy the bound (3). The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con.
[Solved] Consider the first order differential equation. y′ + t/(t^2−4
It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\). The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. Let the function f(t,y) be continuous and satisfy the bound (3).
Then The Differential Equation (2) With Initial Con.
Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with. Notes on the existence and uniqueness theorem for first order differential equations i. It guarantees that equation \ref{eq:2.3.1} has a unique solution on some open interval \((a,b)\).
(B) Is A Uniqueness Theorem.
The existence and uniqueness of solutions to differential equations 5 theorem 3.9.