Homogeneous Vs Inhomogeneous Differential Equations - Thus, these differential equations are. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. You can write down many examples of linear differential equations to. Homogeneity of a linear de. We say that it is homogenous if and only if g(x) ≡ 0. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator.
Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Thus, these differential equations are. We say that it is homogenous if and only if g(x) ≡ 0. Homogeneity of a linear de. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. You can write down many examples of linear differential equations to. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator.
You can write down many examples of linear differential equations to. We say that it is homogenous if and only if g(x) ≡ 0. Thus, these differential equations are. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Homogeneity of a linear de. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the.
[Solved] Determine whether the given differential equations are
Homogeneity of a linear de. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. Thus, these differential equations are. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. We say.
2nd Order Homogeneous Equations
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. You can write down many examples of linear differential equations to. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Thus, these differential equations are. Where f i(x) f i.
(PDF) Differential Equations Educación
Homogeneity of a linear de. We say that it is homogenous if and only if g(x) ≡ 0. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Thus,.
Inhomogeneous Linear Differential Equations KZHU.ai 🚀
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;..
Particular Solution of NonHomogeneous Differential Equations Mr
Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. We say that it is homogenous if and only if g(x) ≡ 0. You can write down many examples of linear differential equations to. Homogeneity of a linear de. The simplest way to.
Homogeneous Differential Equations Docsity
We say that it is homogenous if and only if g(x) ≡ 0. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute.
![10. [Inhomogeneous Equations Variation of Parameters] Differential](https://www.educator.com/media/lesson/poster/differential-equations-murray/inhomogeneous-equations--variation-of-parameters.jpg)
10. [Inhomogeneous Equations Variation of Parameters] Differential
We say that it is homogenous if and only if g(x) ≡ 0. Thus, these differential equations are. Homogeneity of a linear de. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous.
Second Order Inhomogeneous Differential Equations
If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Homogeneity of a linear de. You can write down many examples of linear differential equations to. Thus, these differential equations are. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said.
02 Tugas Kelompok Homogeneous Vs Inhomogeneous PDF
Thus, these differential equations are. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. We say that it is homogenous if and only if g(x) ≡ 0. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Where f i(x).
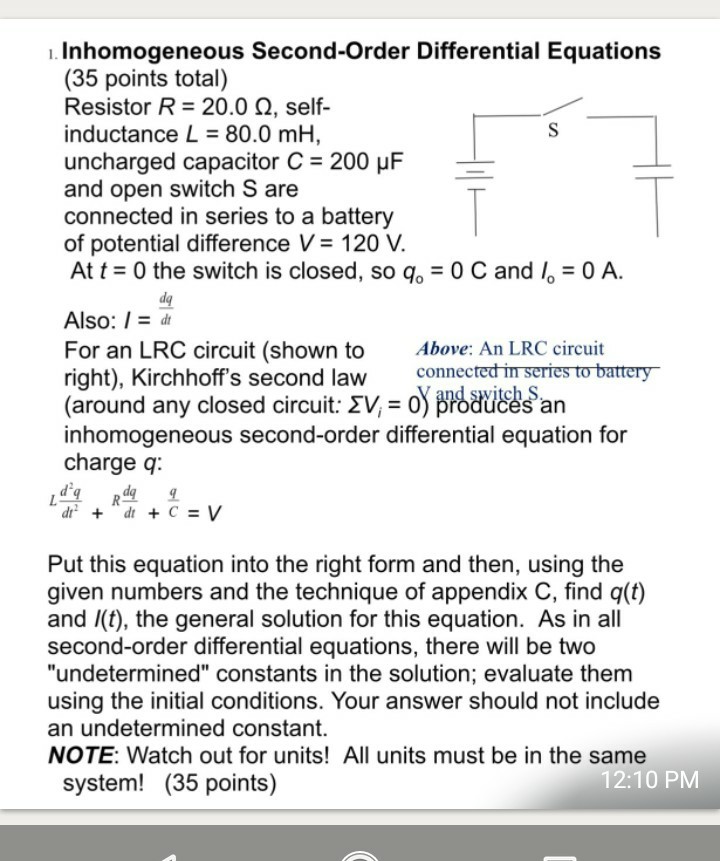
Solved Inhomogeneous SecondOrder Differential Equations (35
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. We say that it is homogenous if and only if g(x) ≡ 0. Homogeneity of a linear de. You can write down many examples of linear differential equations to. Where f i(x) f i (x) and g(x) g (x) are.
You Can Write Down Many Examples Of Linear Differential Equations To.
We say that it is homogenous if and only if g(x) ≡ 0. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Thus, these differential equations are. Homogeneity of a linear de.
The Simplest Way To Test Whether An Equation (Here The Equation For The Boundary Conditions) Is Homogeneous Is To Substitute The.
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g.